A particle is moving along+x aixes according to equation x=5(1−e−2t
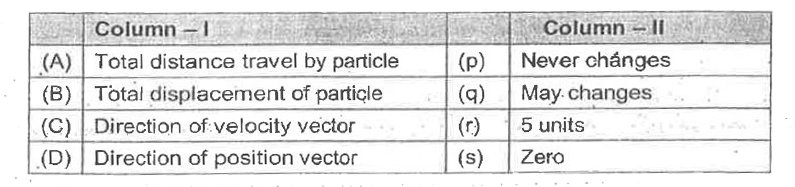
(A)→(r),(B)→(r),(C)→(p),(D)→(p)
x=5(1−e−2t)
v=10(e−2t
Topper's Solved these Questions
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Comprehension
4 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Exercise
23 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Numerical based question
4 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Video
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
Similar Questions
The position of a particle moving along x-axis varies with time t according to equation x=√3sinωt−cosωt where ω is constants. Find the refion in which the particle is confined.
View SolutionA particle moves in x-y plane according to equations x=4t2+5t and 6y=5t The acceleration of the particle must be
View Solution
Knowledge Check
A particle is moving along X-axis according to the following equation: x=u(t−4)+a(t−3)2
All terms in above equation are measured in MKS systemAAcceleration of the particle is a.BAcceleration of the particle is 2a.CVelocity of particle at t=3 s is u.DAt t=0 particle is at the origin.A particle moves along the X−axis according to the equation x=10sin3(πt). The amplitudes and frequency of component SHMs are.
Aamplitude 30/4,10/4: frequencies 3/2,1/2Bamplitude 30/4,10/4: frequencies 1/2,3/2Camplitude 10,10, frequencies 1/2,1/2Damplitude 30/4,10: frequencies 3/2,2A particle moves along the x-axis according to the equation x=4+3sin(2πt)
Hence, x in cm and t in seconds. Select the correct alternativesAThe motion of the particle is simple harmonic with mean position at x=0Bthe motion of the particle is simple harmonic with mean position at x=4cmCThe motion of the particle is simple harmonic with mean position at x=−4cmDAmplitude of osciallation is 3cm
A particle moves along x-axis according to relation x=1+2sinωt. The amplitude of S.H.M. is
View SolutionA particle move a distance x in time t according to equation x=(t+5)−1. The acceleration of particle is alphaortional to.
View SolutionA particle moves a distance x in time t according to equation x2=1+t2 . The acceleration of the particle is
View SolutionA particle moves along x-axis according to the law x=(t3−3t2−9t+5)m. Then :-
View SolutionA particle moves in x-y plane according to the equations x=4t2+5t+16andy=5t where x, y are in metre and t is in second. The acceleration of the particle is
View Solution
