In the adjoining , O is the centre of the circle. ACB is a segment. If ∠OAB=30∘, then find the value of ∠ACB.
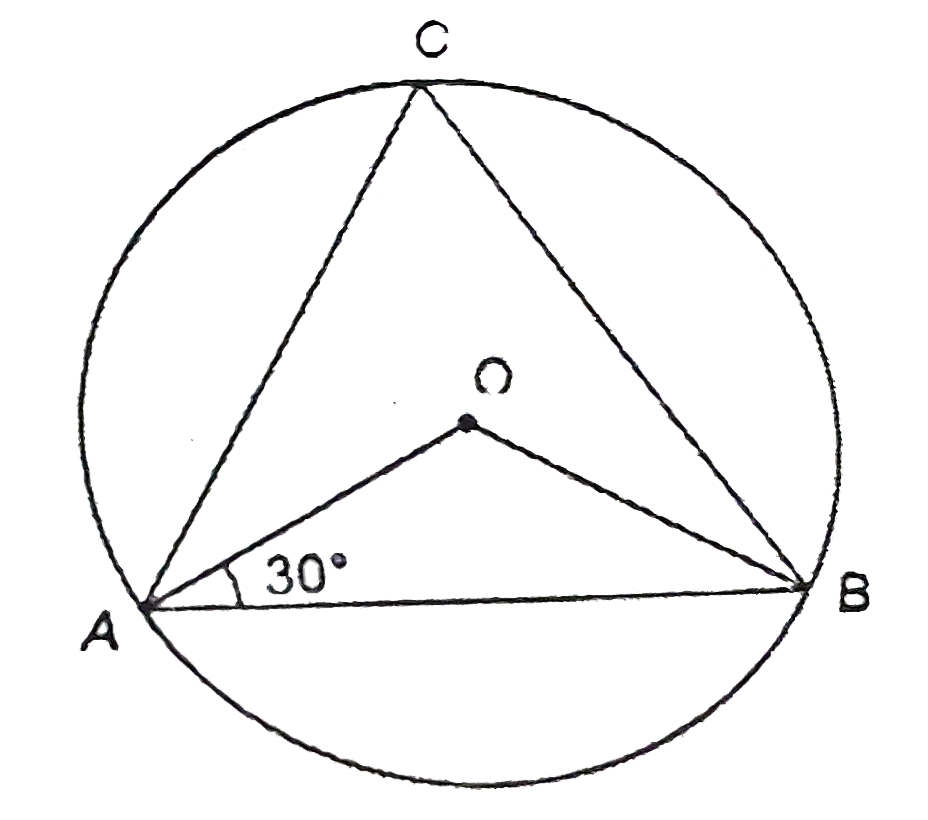
=60∘
=45∘
=30∘
=50∘
The correct Answer is:A
In ΔOAB,
AO=BO (radii of circle)
⇒∠ABO=∠BAO
⇒∠ABO=30∘
Now, ∠AOB=180∘−(∠ABO+∠BAO)
=180∘−(30∘+30∘)
120∘
Arc AB subtends angles at
centre =∠AOB
remaining circle =∠ACB
∴∠ACB=12∠AOB
=12×120∘
=60∘
Topper's Solved these Questions
- CIRCLE BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Exercise 10a
22 Videos
- CIRCLE BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Exercise 10b
19 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CO-ORDINATE GEOMETRY BOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Exercise
8 Videos
Similar Questions
Knowledge Check
In the adjoining figure O is the centre of the circle . If chord AB=2 cm radius OA=2 cm, then find the value of ∠ACB.
 A=60∘B=45∘C=30∘D=50∘
A=60∘B=45∘C=30∘D=50∘
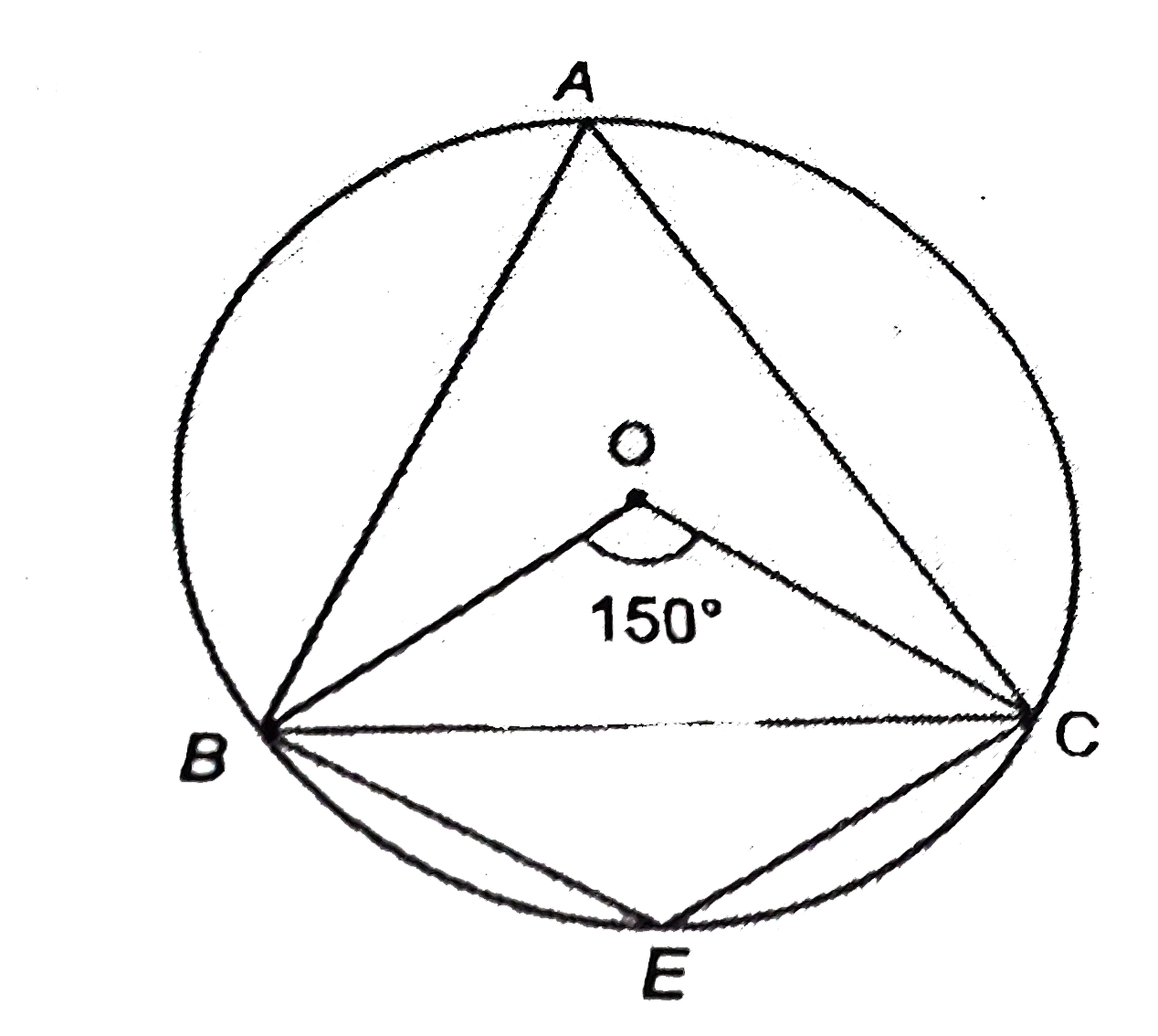
In the adjoining figure, O is the centre of the circle. Find the value of ∠BEC.
View Solution(i) In the adjoining figure, O is the centre of the circle. If ∠OBC=35∘, then find the value of ∠BAC.

(ii) In the adjoining figure, O is the centre of the circle. If ∠OBC=40∘, then find the value of ∠BAC.

View SolutionIn the adjoining figure, AOB is the diameter and O is the centre of the circle. If ∠BDC=60∘, then find the value of ∠ABC.

View SolutionIn the adjoining figure, O is the centre of the circle. If ∠BOP=130∘, then find the value of x.
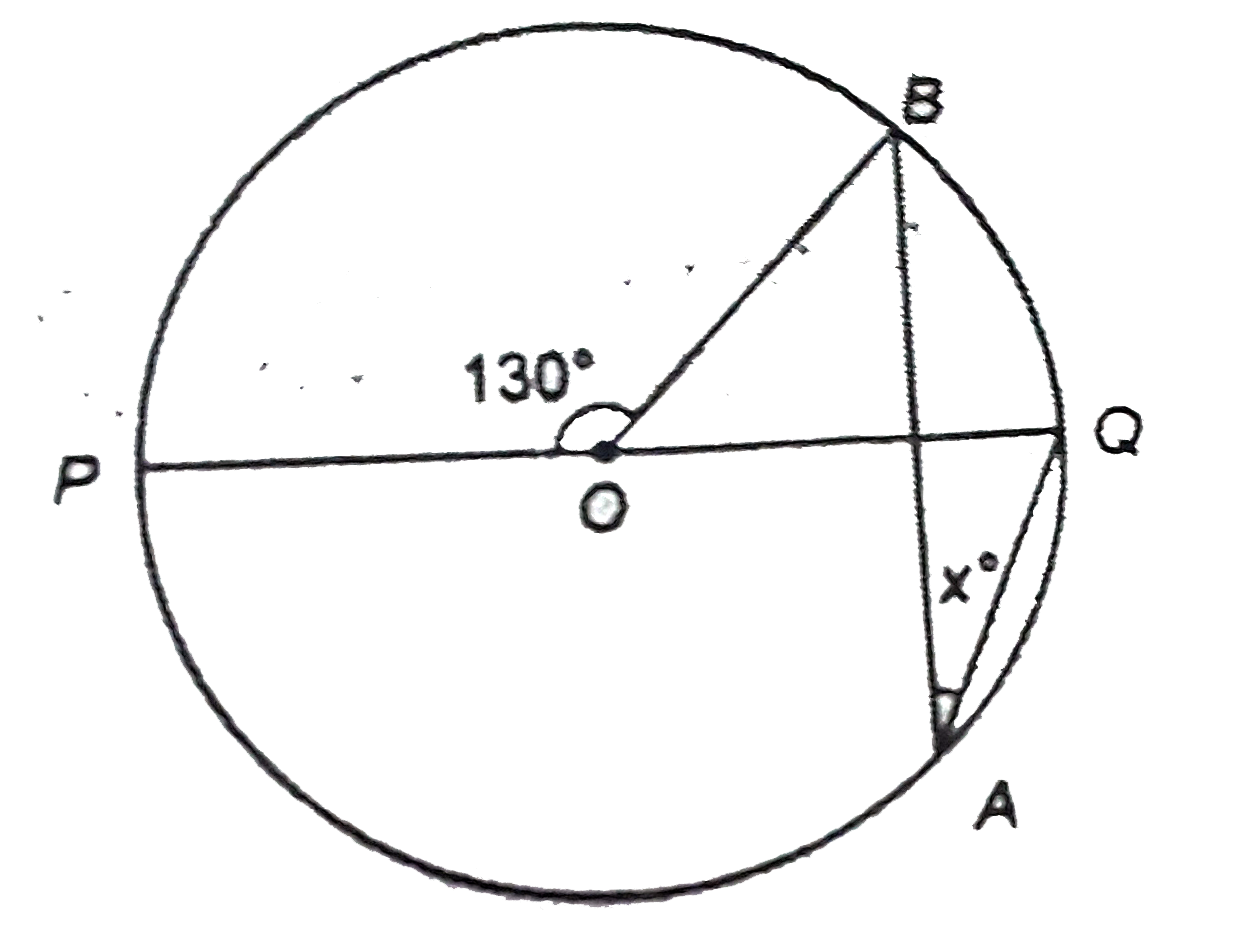
View SolutionIn the adjoining figure, O is the centre of the circle. If ∠AOB=70∘, then find the value of ∠OCD.

View SolutionIn the adjoining figure,O is the centre of the circle. If ∠ABC=110∘ , then find the value of ∠AOC.
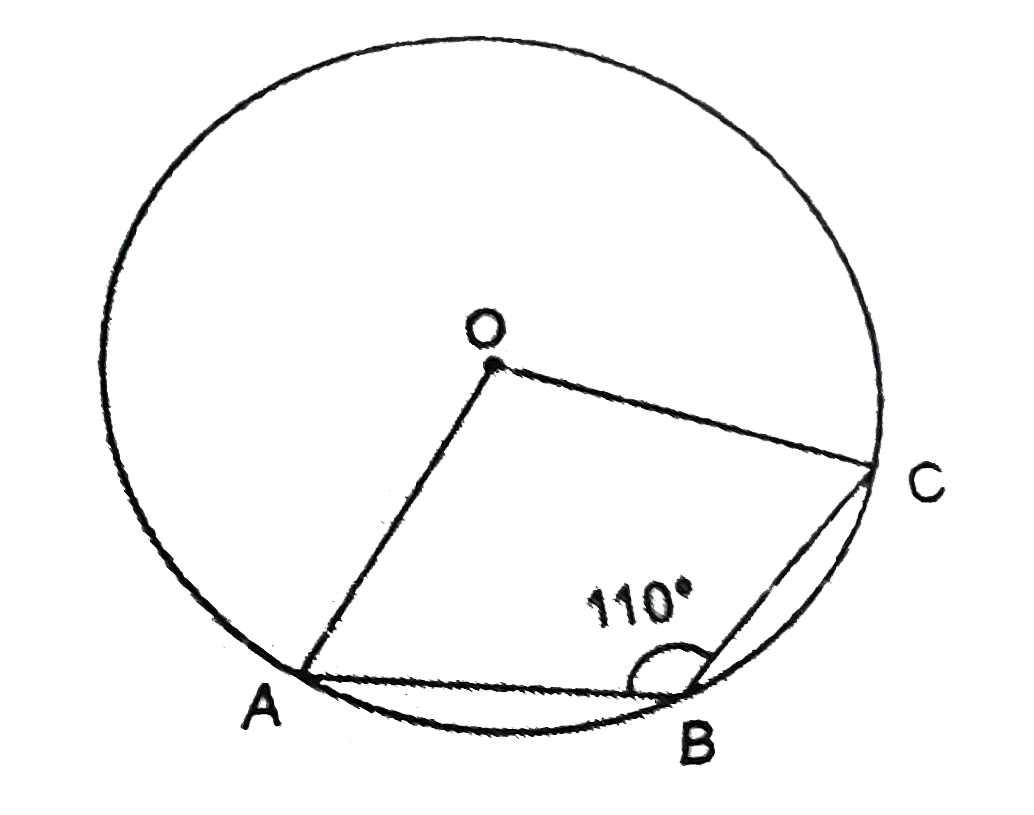
View Solution‘O’ is the centre of the circle. Find ∠(ADB)
View Solution


