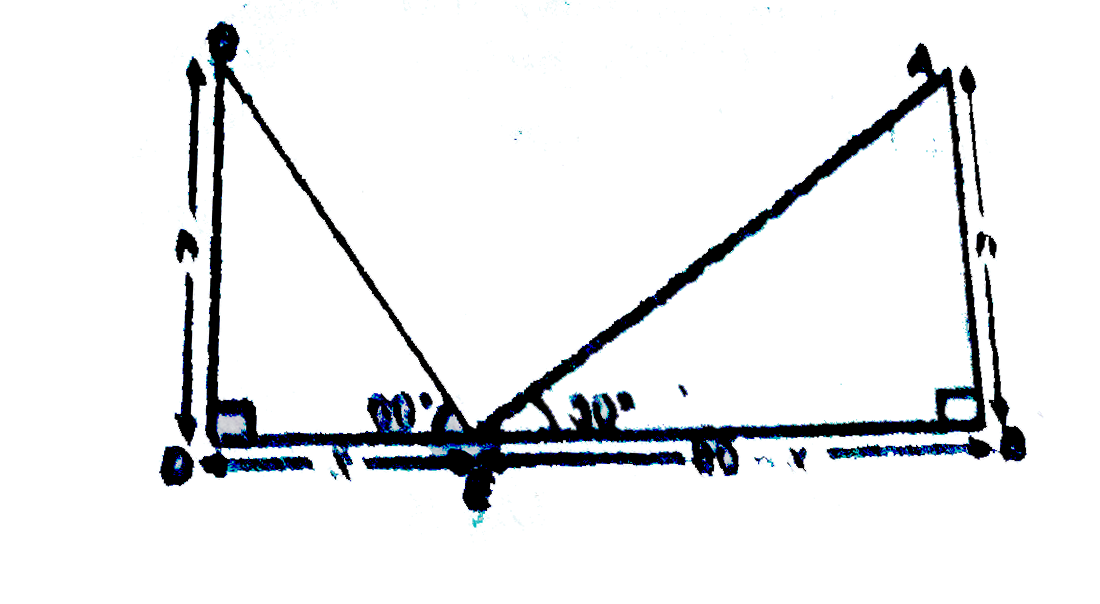
Two poles of equal heights are standing opposite to each other on either side of the road which is 80m wide. From a point between them on the road the angles of elevation of the top of the poles are and respectively. Find the height of the poles and the distances of the point from the poles.
Let AB and CD be two poles of equal height 'h', standing on either side of a road of width 80 m.
At point E, given that
Let
In ,
In ,
Distance of point E from two poles =20 m and 60 m
Topper's Solved these Questions
- SOME APPLICATIONS OF TRIGONOMETRYBook:NAGEEN PRAKASHANChapter:SOME APPLICATIONS OF TRIGONOMETRYExercise:Exercise
Explore 32 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBook:NAGEEN PRAKASHANChapter:SOME APPLICATIONS OF TRIGONOMETRYExercise:Revision Exercise (short Answer Questions)
Explore 5 Videos
- REAL NUMBERSBook:NAGEEN PRAKASHANChapter:REAL NUMBERSExercise:Revision Exercise Long Answer Questions
Explore 5 Videos
- STATISTICS Book:NAGEEN PRAKASHANChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60∘and 30∘, respectively. Find the height of the poles and the distances of the point from the poles.
View SolutionTwo poles of equal heights are standing opposite to each other on either side of the road , which is 100 m wide . From a point between them on the road , the angles of elevation of the top of the poles are 60∘and30∘ respectively .Find the height of the poles .
View Solution
Knowledge Check
Two poles of equal height are standing opposite to each other on either side of a road which is 100 m wide .From a point between them on road , angle of elevation of their tops are 30∘and60∘ .The height of each pole (in metre ) is
A25√3B20√3C28√3D30√3
The two palm trees are of equal heights and are standing opposite each other on either side of the river, which is 80 m wide. From a point O between them on the river the angles of elevation of the top of the trees are 60∘ and 30∘, respectively. Find the height of the trees and the distances of the point O from the trees.
OR
The angles of depression of the top and bottom of a building 50 meters high as observed from the top of a tower are 30∘ and 60∘ respectively. Find the height of the tower, and also the horizontal distance between the building and the tower.
View SolutionTwo poles of equal heights are standing opposite to each other on either side of the road which is 80 m wide. From a point P between them on the road, the angle of elevation of the top of one pole is 60∘ and the angle of depression from the top of another pole is 30∘ find the height of pole and distances of the point P from the poles.
View SolutionTwo pillars of equal height are on either side of a roadway which is 30 m wide. At a point on the roadway between the pillars, the elevations of the top of the pillars are 60∘ and 30∘. Find the height of the pillars and the position of the point.
View SolutionTwo pillars of equal heights stand on either side of a road which is 100 m wide. At a point on the road between the pillars, the angles of elevations of the tops of the pillars are 30∘and45∘. Find the height of each pillar and position of the point on the road.
View SolutionTwo pillars of equal height stand on either side of a roadway which is 120 m wide. At a point in the road between the pillars, the angles of elevation of the pillars are 60∘ and 30∘. Find the height of each pillars to the nearest metre and position of the point from both the pillars.
View SolutionFrom te top of a tower , 60 meters high, the angles of depression of the top and bottom of a pole are αandβ respectively .Find the height of the pole.
View SolutionTwo poles are erected on either side of the bank of a river just opposite to each other. One pole is 40 m high. Form the top and foot of this pole , the angles of elevation of the top of the other pole are 30∘ and 60∘ respectively. Find the height of the other pole and the width of the river.
View Solution