Two stations due south of a leaning tower which leans towards the north are at distances a and b from its foot If α and β are the elevations of the top of the tower from these stations then prove that its inclination θ to the horizontal is given by cotθ=bcotα−acotβb−a
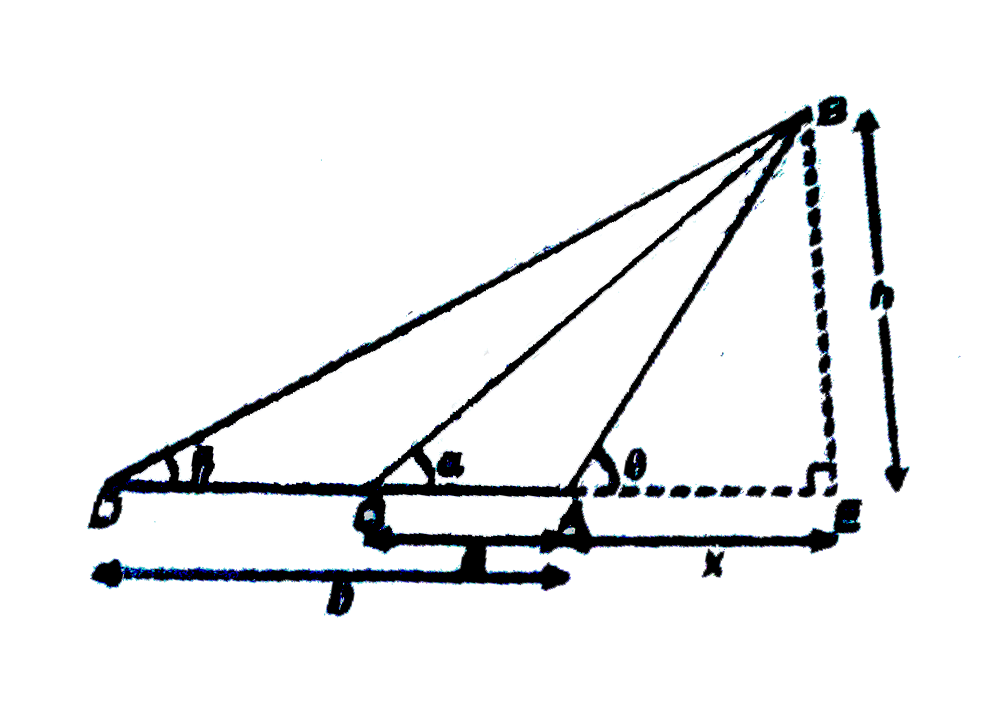
Let AB be a leaning tower and let C and D be two stations due south at distances a and b respectively from the foot A of the tower.
Let AE = x and BE = h
In right ΔBEA,
tanθ=hx⇒x=hcotθ…(1)
In ΔCEB,
tanαha+x⇒a+x=hcotα...(2)
In ΔDEB,
tanβ=hb+x⇒b+x=hcotβ...(3)
Multiplying equation (2) by b and equation (3) by a, we get
ab+bx=bhcotα...(4)
and _ab±ax=±ahcotβx(b−a)=h(bcotα−αcotβ)
∴xh=bcotα−acotβb−a⇒cotθ=bcotα−acotβb−a [from(1)]
Topper's Solved these Questions
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise
32 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)
5 Videos
- REAL NUMBERSBOOK - NAGEEN PRAKASHANCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- STATISTICS BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
5 Videos
Similar Questions
Two stations due south of a leaning tower which leans towards the north are at distances a and b from its foot If α and β are the elevations of the top of the tower from these stations then prove that its inclination θ to the horizontal is given by cotθ=bcotα−acotβb−a
View SolutionTwo stations due south of a leaning tower which leans towards the north are at distances aandb from its foot. If αβ be the elevations of the top of the tower from these stations, prove that its inclination θ to the horizontal is given by cotθ=bcotα−acotβb−a
View Solution
Knowledge Check
Two stations due south of a leaning tower which leans towards the north are respectively at distance x and y from its foot (y>x). If αandβ be the elevations of the top of the tower from these stations and θ is inclination of the tower to the horizontal then cotθ equals
Axcotα−ycotβx−yBx−yxcotα−ycotβCycotα−xcotβy−xDy−xycotα−xcotβThe respective ratio between the height of tower and the point at some distance from its foot is 5√3:5 .What will be the angle of elevation of the top of the tower ?
A30∘B60∘C90∘D45∘PQ is a part of a given height a and AB is a tower at some distance, αandβ are the angles of elevation of B, the top of the tower at P and Q respectively. The height of the tower is
Aasinαsinβsin(α−β)Bacosαcosβsin(α−β)Casinαcosβsin(α−β)DNone
Two stations due south of a leaning tower which leans towards the north are respectively at distance x and y from its foot (y>x). If αandβ be the elevations of the top of the tower from these stations and θ is inclination of the tower to the horizontal then cotθ equals
View SolutionPQ is a post of given height a , and AB is a tower at some distance. If α and β are the angles of elevation of B , the top of the tower, at P and Q respectively. Find the height of the tower and its distance from the post.
View SolutionThe angle of elevation of the top of a tower from a point 40 m away from its foot is 60∘. Find the height of the tower.
View SolutionFrom the foot of a building the elevation of the top of a tower is 45∘ and from the foot of the twoer the elevation of the top of the building is 60∘ . Which of the two is shorter ?
View SolutionFrom a point on the ground which is at a distance of 50 m from the foot of the towe, the angle of elevation of the top of the tower is observed to be 30∘. Find the height of the tower.
View Solution