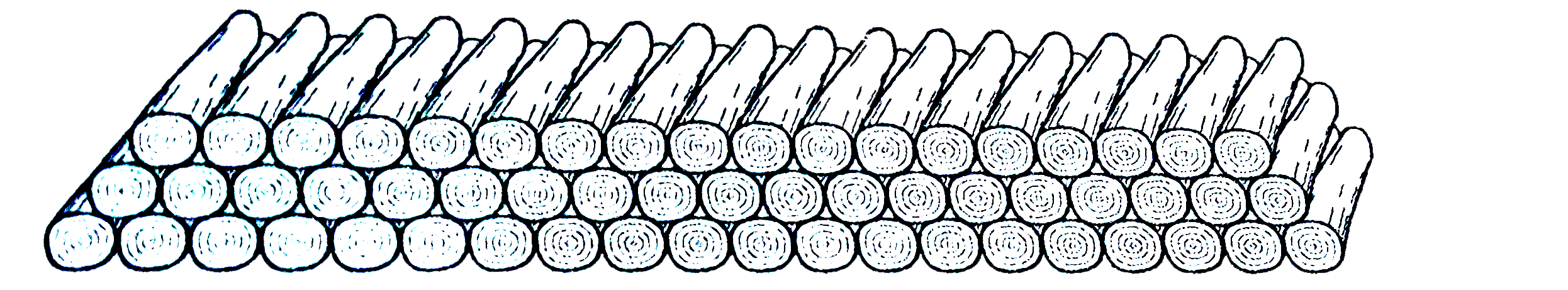
200 logs are stacked in such a way that there are 20 logs in the bottom row, 19 in the next row, 18 in the next row and so on. In how many rows, 200 logs are placed and how many logs are there in the top row?
The correct Answer is:16 rows, 5 logs
To solve the problem of how many rows are there when 200 logs are stacked in decreasing order, we can follow these steps:
Step 1: Understand the problem
We have 200 logs stacked in rows where the first row has 20 logs, the second row has 19 logs, and this pattern continues until we reach the top row. We need to find out how many rows there are and how many logs are in the top row.
Step 2: Identify the arithmetic progression (AP)
The number of logs in each row forms an arithmetic progression (AP) where:
- The first term A=20 (logs in the bottom row)
- The common difference D=−1 (each subsequent row has one log less)
Step 3: Write the formula for the sum of the first n terms of an AP
The sum Sn of the first n terms of an AP can be calculated using the formula:
Sn=n2×(2A+(n−1)D)
Where:
- Sn is the total number of logs (200 in this case)
- n is the number of rows
- A is the first term (20)
- D is the common difference (-1)
Step 4: Set up the equation
Substituting the known values into the formula:
200=n2×(2×20+(n−1)(−1))
This simplifies to:
200=n2×(40−n+1)
200=n2×(41−n)
Step 5: Eliminate the fraction
Multiply both sides by 2 to eliminate the fraction:
400=n(41−n)
Step 6: Rearrange the equation
Rearranging gives us a quadratic equation:
n2−41n+400=0
Step 7: Solve the quadratic equation
Using the quadratic formula n=−b±√b2−4ac2a:
Here, a=1, b=−41, and c=400.
n=41±√(−41)2−4⋅1⋅4002⋅1
n=41±√1681−16002
n=41±√812
n=41±92
Calculating the two possible values:
1. n=502=25
2. n=322=16
Step 8: Determine the valid solution
Since we are stacking logs, n must be a positive integer. Thus, we have two potential solutions: n=25 or n=16. However, since the logs decrease from 20 to 1, we can only have 20 rows maximum. Therefore, the valid solution is n=25.
Step 9: Find the number of logs in the top row
To find the number of logs in the top row, we can use the formula for the n-th term of an AP:
L=A+(n−1)D
Substituting n=25:
L=20+(25−1)(−1)=20−24=−4
Since this doesn't make sense, we should check our calculations. The maximum number of rows we can have is 20, so we can only have 20 rows.
Final Answer:
- Number of rows = 20
- Logs in the top row = 1
We have 200 logs stacked in rows where the first row has 20 logs, the second row has 19 logs, and this pattern continues until we reach the top row. We need to find out how many rows there are and how many logs are in the top row.
The number of logs in each row forms an arithmetic progression (AP) where:
- The first term A=20 (logs in the bottom row)
- The common difference D=−1 (each subsequent row has one log less)
The sum Sn of the first n terms of an AP can be calculated using the formula:
Sn=n2×(2A+(n−1)D)
Where:
- Sn is the total number of logs (200 in this case)
- n is the number of rows
- A is the first term (20)
- D is the common difference (-1)
Substituting the known values into the formula:
200=n2×(2×20+(n−1)(−1))
This simplifies to:
200=n2×(40−n+1)
200=n2×(41−n)
Multiply both sides by 2 to eliminate the fraction:
400=n(41−n)
Rearranging gives us a quadratic equation:
n2−41n+400=0
Using the quadratic formula n=−b±√b2−4ac2a:
Here, a=1, b=−41, and c=400.
n=41±√(−41)2−4⋅1⋅4002⋅1
n=41±√1681−16002
n=41±√812
n=41±92
1. n=502=25
2. n=322=16
Since we are stacking logs, n must be a positive integer. Thus, we have two potential solutions: n=25 or n=16. However, since the logs decrease from 20 to 1, we can only have 20 rows maximum. Therefore, the valid solution is n=25.
To find the number of logs in the top row, we can use the formula for the n-th term of an AP:
L=A+(n−1)D
Substituting n=25:
L=20+(25−1)(−1)=20−24=−4
Since this doesn't make sense, we should check our calculations. The maximum number of rows we can have is 20, so we can only have 20 rows.
- Number of rows = 20
- Logs in the top row = 1
Topper's Solved these Questions
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Exercise 5d
10 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Very Short Answer Questions
10 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Exercise 5b
23 Videos
- AREA RELATED TO CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - AREA RELATED TO CIRCLESEXERCISE - Revision Exercise Long Answer Question
6 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Revision Exercise Long Answer Questions
3 Videos
Similar Questions
200 logs are stacked in the following manner: 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on (see Figure). In how may rows are the 200 logs placed and how many logs are in the top row?
View SolutionThree hundred sixty bricks are stacked in the following manner: 30 bricks in the bottom row, 29 in the next row, 28 in the row next to it and so on. In how many rows are the 360 bricks placed and how many bricks are there in the top row?
View Solution
Knowledge Check
Aman's rank is 10th from top and 12th from bottom in a row of people. How many people are there in the row?
A21B22C15D18
In a flower bed, there are 51 plants in the first row, 48 plants in the second row, 45 plants in the third row and so on. There are 12 plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed, there are 43 rose plants in the first row, 41 in the second, 39 in the third, and so on. There are 11 rose plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed, there are 23 rose plants in the first row, 21 in the second, 19 in the third, and so on. There are 5 rose plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed there are 23 rose plants in the first row, twenty one in the second row, nineteen in the third row and so on. There are five plants in the last row. How many rows are there in the flower bed?
View SolutionIn a rose garden, there are 32 rose plants in the first row, 29 in the second row, 26 in the third row and so on. There are 5 rose plants in the last row. How many rows of rose plants are there in the rose garden ?
View SolutionWhat is the next row of numbers?
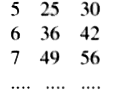
View SolutionWhat would be the next row of the numbers ?
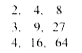
View Solution