Question

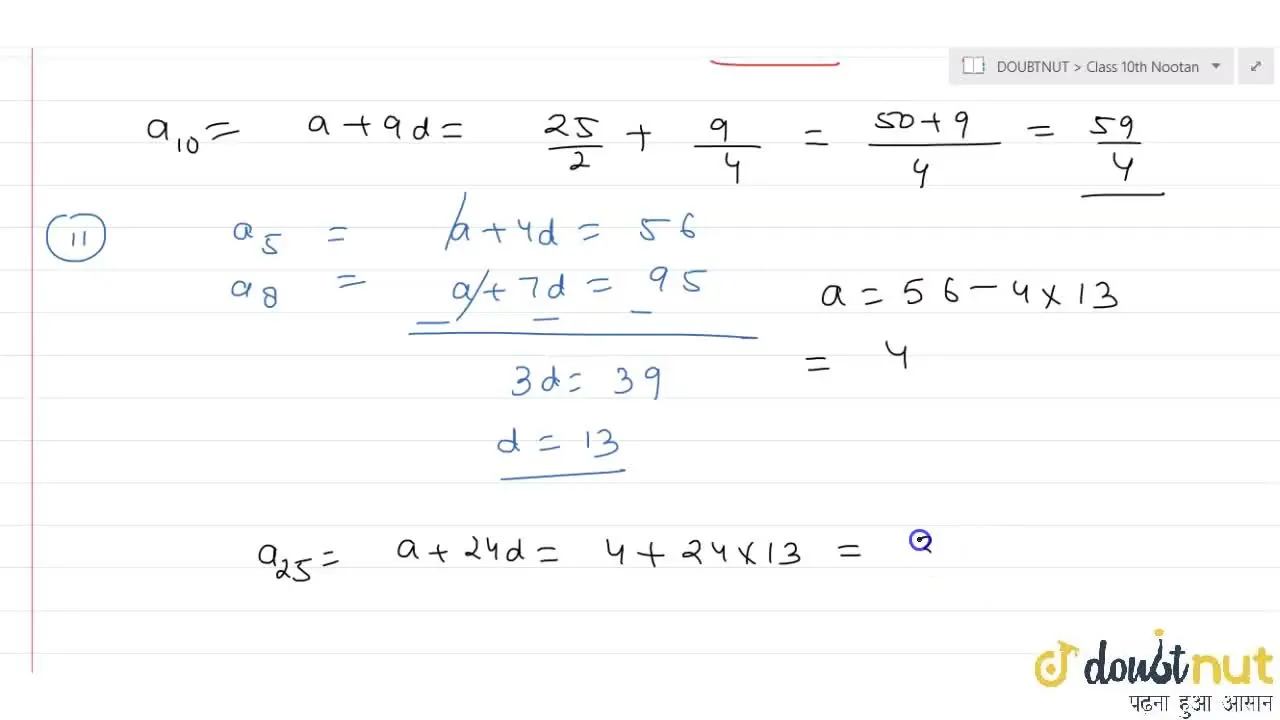
(i) The 3rd and 19th terms of an A.P. are 13 and 17 respectively. Find its 10th term. (ii) The 5th and 8th terms of an A.P. are 56 and 95 respectively. Find its 25th term.

Answer

(i) 41 (ii)316

Explanation

To solve the given problems step by step, we will break down each part clearly.

Part (i)

Given:
- The 3rd term $$A_3=13$$
- The 19th term $$A_{19}=17$$

Step 1: Write the formulas for the terms of an A.P.
The nth term of an A.P. can be expressed as:
\( A_n = A + (n-1)d \)
where \( A \) is the first term and \( d \) is the common difference.

Step 2: Set up equations for the given terms.
For the 3rd term:
\( A + 2d = 13 \quad \text{(1)} \)
For the 19th term:
\( A + 18d = 17 \quad \text{(2)} \)

Step 3: Solve the equations simultaneously.
Subtract equation (1) from equation (2):
\( (A + 18d) - (A + 2d) = 17 - 13 \)
This simplifies to:
\( 16d = 4 \)
Thus, we find:
\( d = \frac{4}{16} = \frac{1}{4} \)

Step 4: Substitute \( d \) back to find \( A \).
Substituting \( d \) into equation (1):
\( A + 2 \left(\frac{1}{4}\right) = 13 \)
This simplifies to:
\( A + \frac{1}{2} = 13 \)
So,
\( A = 13 - \frac{1}{2} = \frac{26}{2} - \frac{1}{2} = \frac{25}{2} \)

Step 5: Find the 10th term \( A_{10} \).
Using the formula for the 10th term:
\( A_{10} = A + 9d \)
Substituting the values of \( A \) and \( d \):
\( A_{10} = \frac{25}{2} + 9 \left(\frac{1}{4}\right) \)
This simplifies to:
\( A_{10} = \frac{25}{2} + \frac{9}{4} \)
To add these fractions, convert \( \frac{25}{2} \) to a fraction with a denominator of 4:
\( A_{10} = \frac{50}{4} + \frac{9}{4} = \frac{59}{4} \)

Final Answer for Part (i):
The 10th term is \( \frac{59}{4} \).

Part (ii)

Given:
- The 5th term \( A_5 = 56 \)
- The 8th term \( A_8 = 95 \)

Step 1: Set up equations for the given terms.
For the 5th term:
\( A + 4d = 56 \quad \text{(3)} \)
For the 8th term:
\( A + 7d = 95 \quad \text{(4)} \)

Step 2: Solve the equations simultaneously.
Subtract equation (3) from equation (4):
\( (A + 7d) - (A + 4d) = 95 - 56 \)
This simplifies to:
\( 3d = 39 \)
Thus, we find:
\( d = \frac{39}{3} = 13 \)

Step 3: Substitute \( d \) back to find \( A \).
Substituting \( d \) into equation (3):
\( A + 4(13) = 56 \)
This simplifies to:
\( A + 52 = 56 \)
So,
\( A = 56 - 52 = 4 \)

Step 4: Find the 25th term \( A_{25} \).
Using the formula for the 25th term:
\( A_{25} = A + 24d \)
Substituting the values of \( A \) and \( d \):
\( A_{25} = 4 + 24(13) \)
This simplifies to:
\( A_{25} = 4 + 312 = 316 \)

Final Answer for Part (ii):
The 25th term is \( 316 \).