In the given figure two circles touch each other at the point C. Prove that the common tangent at P and Q.
In the given figure, PR and CR are both tangents drawn to the same circle from an external point R
∴ PR=CR …(1)
Also, QR and CR are both tangent drawn to the same circle from an external point R.
∴ QR=CR
From (1) and (2) we get
PR=QR
∴ R is the mid-point of PQ
i.e., the common tangent at C bisects the common tangents at P and Q.
 Hence Proved.
Hence Proved.
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Exercise
25 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
9 Videos
Similar Questions
In Fig. 10.21, two circles touch each other at the point C . Prove that the common tangent to the circles at C , bisects the common tangent at P and Q . (FIGURE)
View SolutionIn the given figure, two circles touch each other internally at point P., where Q is the centre of larger circle. If RS = 17 cm, TU = 9 cm and TV⊥RP , then find the area of shaded region.
View Solution
Knowledge Check
The two circles touch each other if
Ac=√a2+b2B1c=1a2+1b2Cc=1a2+1b2Dc=1a2+b2Statement 1 : The number of common tangents to the circles x2+y2−x=0 and x2+y2+x=0 is 3.
Statement 2 : If two circles touch each other externally then it has two direct common tangents and one indirect common tangent.AStatement-1 : is True, Statement-2 is True and Statement-2 is a correct explanation for Statement-1BStatement-1 is True, Statement-2 is True and Statement-2 is NOT a correct explanation for Statement -1CStatement-1 is True, Statement -2 is FalseDStatement-1 is False, Statement-2 is TrueIf two circles touch each other internally, only _____ common tangent can be drawn to them.
A0B1C2D3
In figure, two circles intersect each other at points S and R. Their common tangent PQ touches the circle at points P,Q. Prove that, ∠PRQ+∠PSQ=180∘.

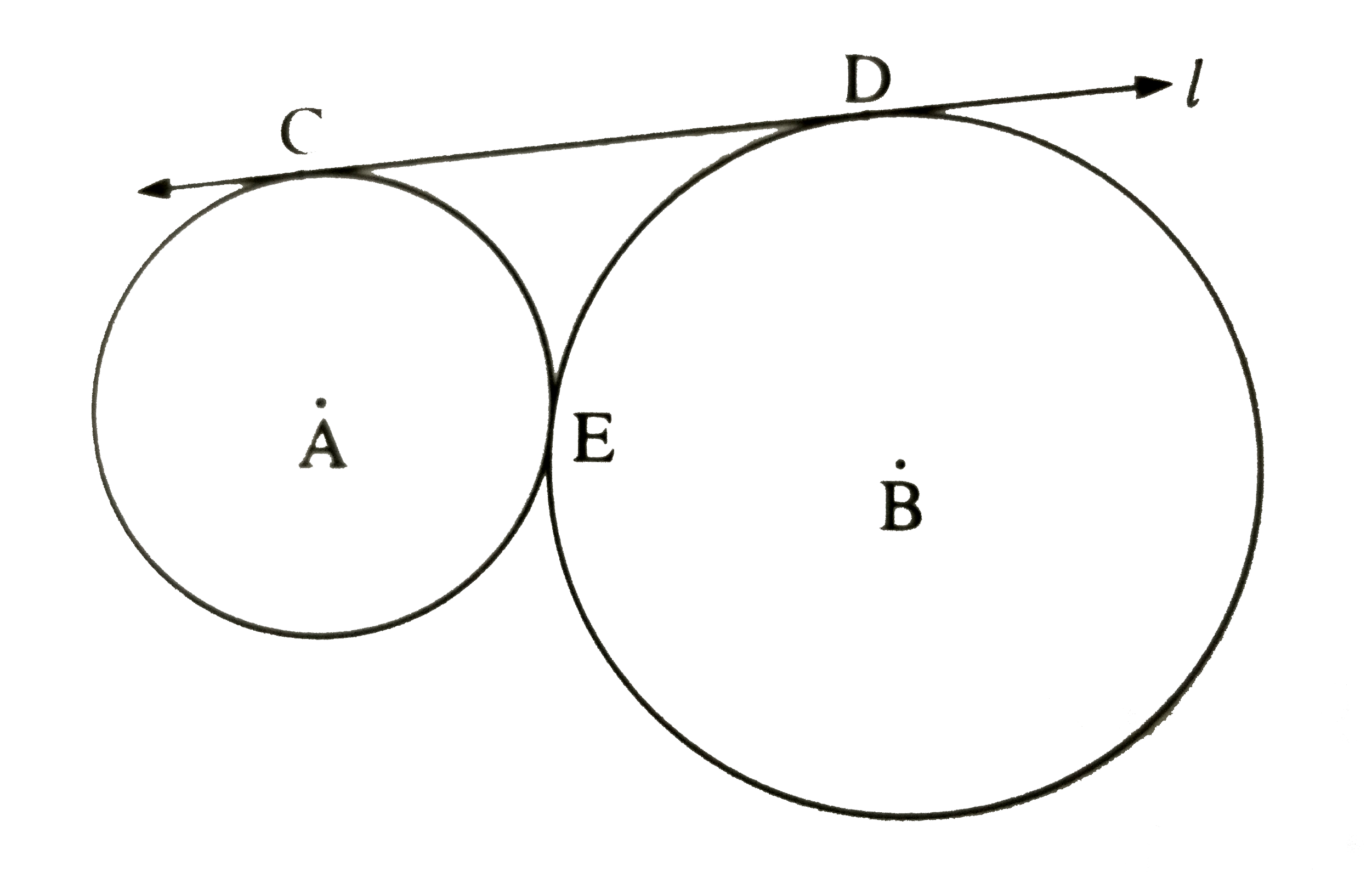
View SolutionIn the figure, the circles with centeres A and B touch each other at E. Line l is a common tangent which touches the circles at C and D respectively. Find the length of seg CD, if the radii of the circle are 4 cm, 6 cm.
View SolutionStatement 1: The number of common tangents to the circles x2+y2=4 and x2+y2−6x−6y=24 is 3.
Statement 2 : If two circles touch each other externally thenit has two direct common tangents and one indirect common tangent.
View SolutionQR is a common tangent to the given circles touching each other externally at the point T. The tangents at T meets QR at P. if PT = 3.8 cm , then the length of QR ( in cm) is
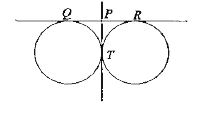
View SolutionO and O' are the centres of two circles which touch each other externally at P. AB is a common tangent. Find ∠APO
View Solution
