Question

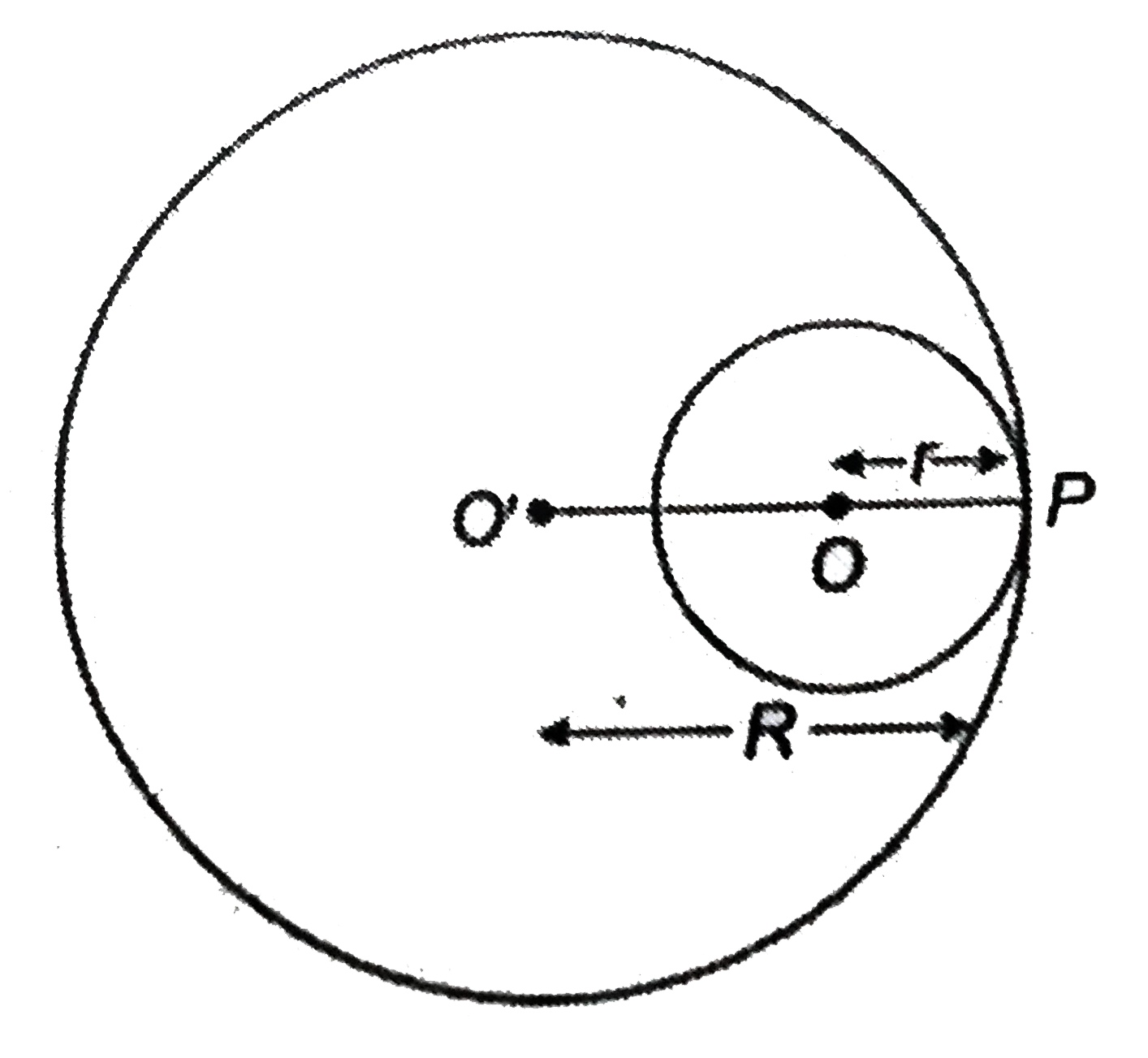
Two circules touch internally. The sum of their areas is $116\pi c{m}^2$ and the distance between their centres is 6 cm. find the radii of the circles.

Explanation

To solve the problem of finding the radii of two circles that touch internally, we can follow these steps:

1. Define Variables:
Let the radius of the outer circle be \( R \) and the radius of the inner circle be \( r \).

2. Use the Given Information:
We know that the distance between the centers of the two circles is 6 cm. Since the circles touch internally, we can express this relationship as:
\( R - r = 6 \quad \text{(1)} \)

3. Area Relationship:
The sum of the areas of the two circles is given as \( 116\pi \, \text{cm}^2 \). The area of a circle is given by \( \pi r^2 \). Therefore, we can write:
\( \pi R^2 + \pi r^2 = 116\pi \)
Dividing through by \( \pi \) gives:
\( R^2 + r^2 = 116 \quad \text{(2)} \)

4. Substituting Equation (1) into Equation (2):
From equation (1), we can express \( R \) in terms of \( r \):
\( R = r + 6 \)
Now substitute this expression for \( R \) into equation (2):
\( (r + 6)^2 + r^2 = 116 \)

5. Expand and Simplify:
Expanding \( (r + 6)^2 \):
\( r^2 + 12r + 36 + r^2 = 116 \)
Combine like terms:
\( 2r^2 + 12r + 36 = 116 \)
Subtract 116 from both sides:
\( 2r^2 + 12r - 80 = 0 \)

6. Divide the Equation by 2:
Simplifying the equation:
\( r^2 + 6r - 40 = 0 \)

7. Factor the Quadratic Equation:
We need to factor the quadratic:
\( (r + 10)(r - 4) = 0 \)
This gives us two possible solutions for \( r \):
\( r + 10 = 0 \quad \Rightarrow \quad r = -10 \quad \text{(not valid)} \)
\( r - 4 = 0 \quad \Rightarrow \quad r = 4 \quad \text{(valid)} \)

8. Find \( R \):
Now substitute \( r = 4 \) back into equation (1) to find \( R \):
\( R = r + 6 = 4 + 6 = 10 \)

9. Final Answer:
The radii of the circles are:
\( R = 10 \, \text{cm} \quad \text{and} \quad r = 4 \, \text{cm} \)