Question

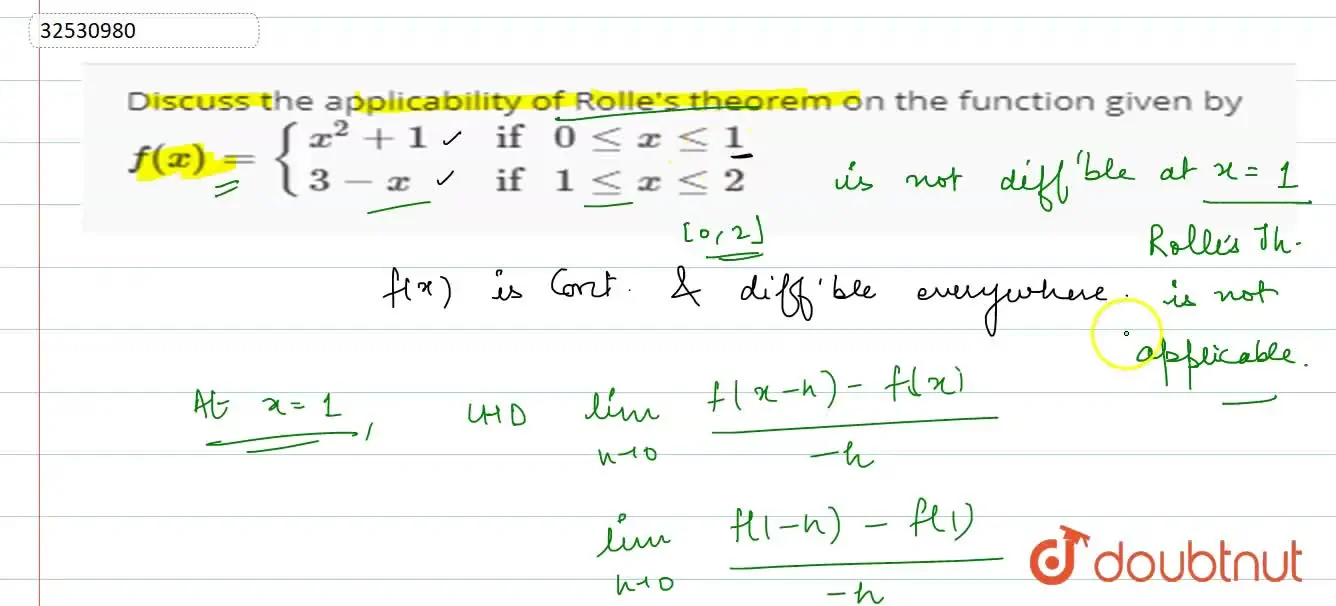
Discuss the applicability of Rolle's theorem on the function given by
${\displaystyle f\left(x\right)=\{\begin{array}{ll}{x}^2+1& if0\le x\le 1\\ 3-x& if1\le x\le 2\end{array}}$

Answer

N/a

Explanation

To discuss the applicability of Rolle's theorem for the given function $$f\left(x\right)$$, we need to follow these steps:

Step 1: Define the function
The function \( f(x) \) is defined piecewise as follows:
\( f(x) = \begin{cases} x^2 + 1 & \text{if } 0 \leq x \leq 1 \\ 3 - x & \text{if } 1 < x \leq 2 \end{cases} \)

Step 2: Check continuity
To apply Rolle's theorem, the function must be continuous on the closed interval \((0, 2)\). We need to check the continuity at the point where the definition of the function changes, which is at \( x = 1 \).

1. Calculate \( f(1) \):
\( f(1) = 1^2 + 1 = 2 \)

2. Calculate the left-hand limit as \( x \) approaches 1:
\( \lim_{x \to 1^-} f(x) = f(1) = 2 \)

3. Calculate the right-hand limit as \( x \) approaches 1:
\( \lim_{x \to 1^+} f(x) = 3 - 1 = 2 \)

Since both the left-hand limit and right-hand limit at \( x = 1 \) are equal to \( f(1) \), we conclude that \( f(x) \) is continuous on \((0, 2)\).

Step 3: Check differentiability
Next, we need to check if \( f(x) \) is differentiable on the open interval \( (0, 2) \). We specifically need to check the differentiability at \( x = 1 \).

1. Calculate the left-hand derivative at \( x = 1 \):
\( f'(1^-) = \lim_{h \to 0} \frac{f(1) - f(1-h)}{h} \)
Here, \( f(1-h) = (1-h)^2 + 1 = 1 - 2h + h^2 + 1 = 2 - 2h + h^2 \).
\( f'(1^-) = \lim_{h \to 0} \frac{2 - (2 - 2h + h^2)}{h} = \lim_{h \to 0} \frac{2h - h^2}{h} = \lim_{h \to 0} (2 - h) = 2 \)

2. Calculate the right-hand derivative at \( x = 1 \):
\( f'(1^+) = \lim_{h \to 0} \frac{f(1+h) - f(1)}{h} \)
Here, \( f(1+h) = 3 - (1+h) = 2 - h \).
\( f'(1^+) = \lim_{h \to 0} \frac{(2 - h) - 2}{h} = \lim_{h \to 0} \frac{-h}{h} = -1 \)

Since the left-hand derivative \( f'(1^-) = 2 \) and the right-hand derivative \( f'(1^+) = -1 \) are not equal, \( f(x) \) is not differentiable at \( x = 1 \).

Step 4: Conclusion
Since \( f(x) \) is continuous on \((0, 2)\) but not differentiable at \( x = 1\), we conclude that Rolle's theorem is not applicable for the function \( f(x) \) on the interval \((0, 2)\).

---