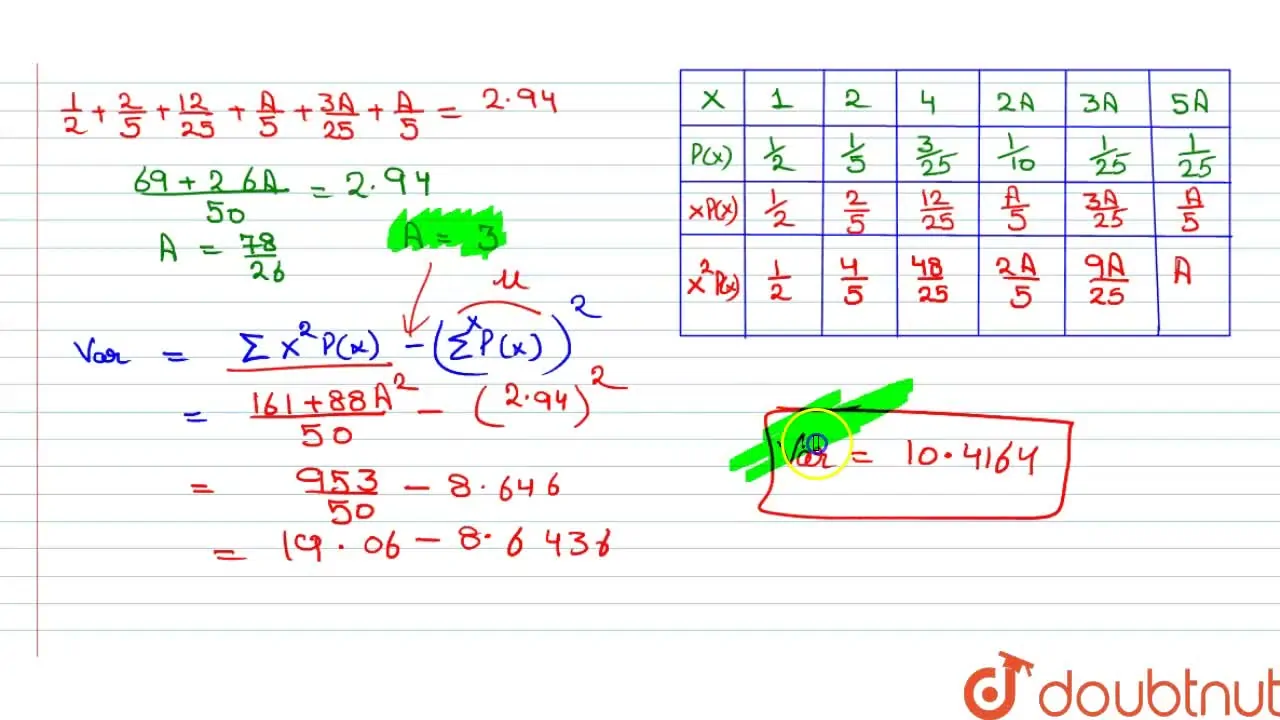
The probability distribution of a discrete random variable x is given as under
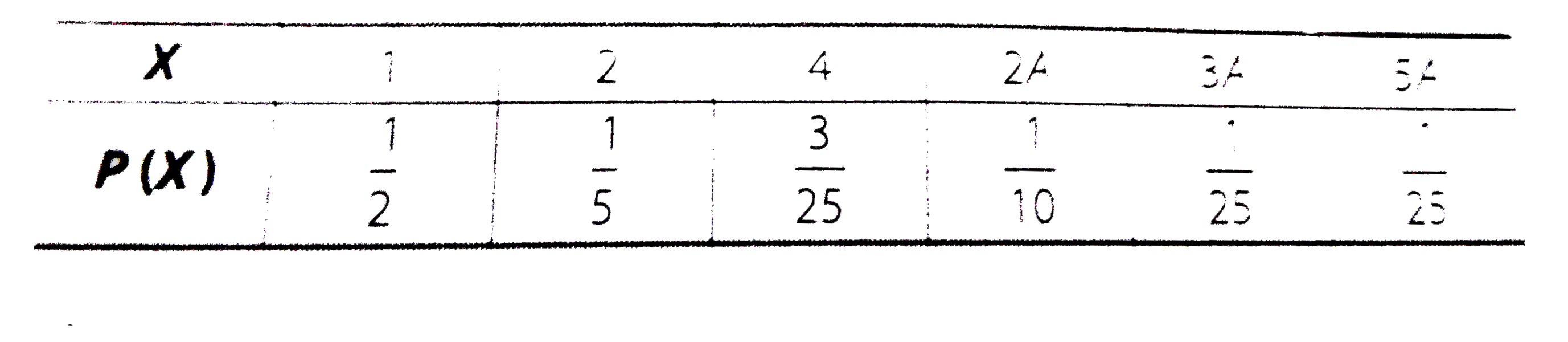
Calculate
(i) the value of A, if E(X)=2.94.
(ii) variance of X.
(i) We have,∑XP(X)=12+25+1225+2A10+3A25+5A25
=25+20+24+10A+6A+10A50=69+26A50
Since, E(X)=∑XP(X)
⇒2.94=69+26A50
⇒26A=50×2.94−69
⇒A=147−6926=7826=3
(ii) We know that,
Var(X)=E(X2)−[E(X)]2
=∑X2P(X)−[∑XP(X)]2
=12+45+4825+4A210+9A225+25A225−[E(X)]2
=20+40+96+20A2+18A2+50A250−[E(X)]2
=161+88A250−[E(C)]2=161+88×(3)250−[E(X)]2 [∵A=3]
=95350−[2.94]2 [∵E(X)=2.94]
=19.0600-8.6436=10.4164
Related Playlists
Similar Questions
The probability distribution of a discrete random variable X is given below
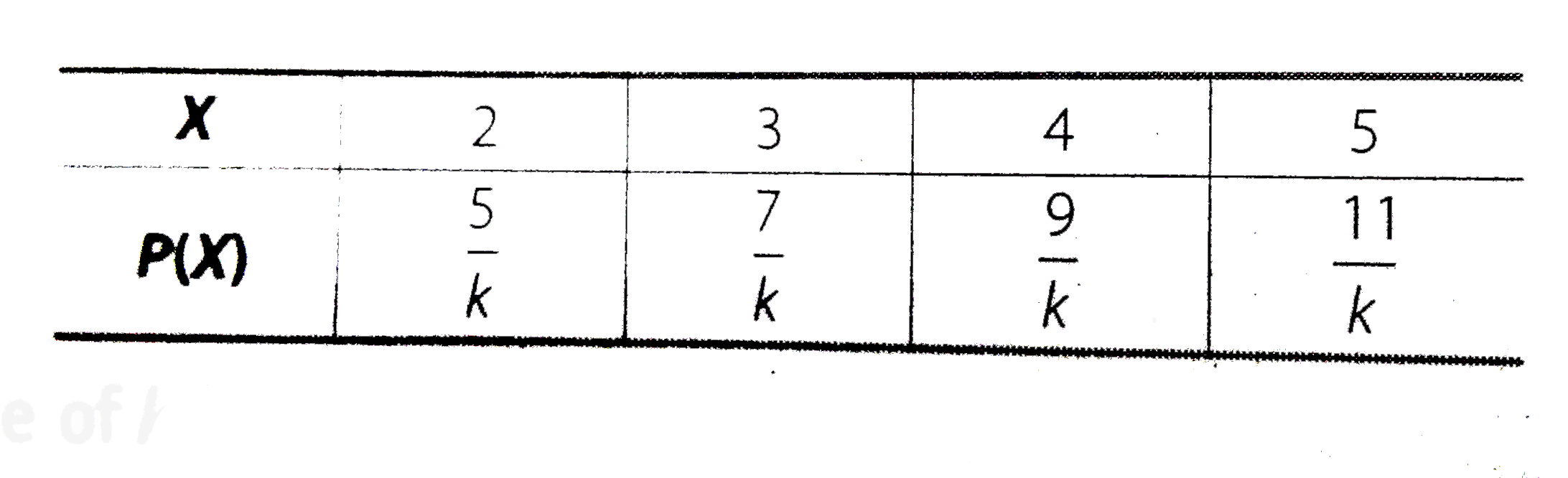
The value of k isView SolutionThe probability distribution of a random variable X is given as
Then, the value of p is
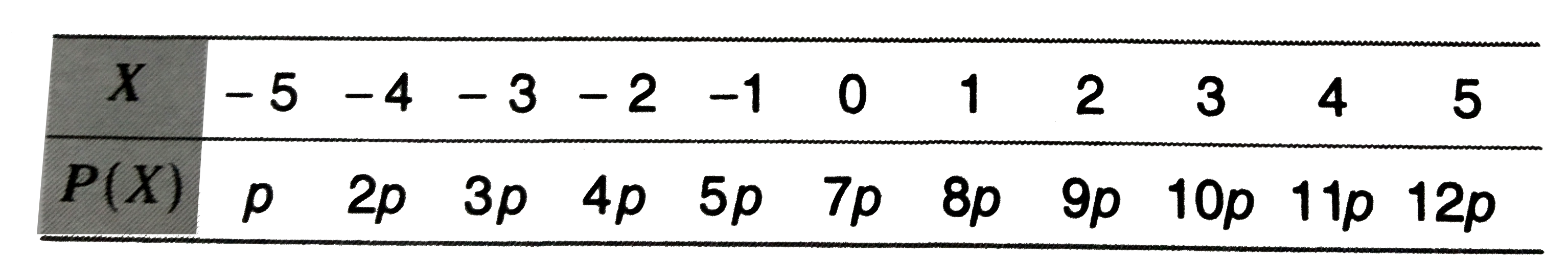 View Solution
View SolutionThe probability distribution of a random variable X is given by

Then 12σ=View SolutionThe probability distrubtion of a discrete random variable X is as below :
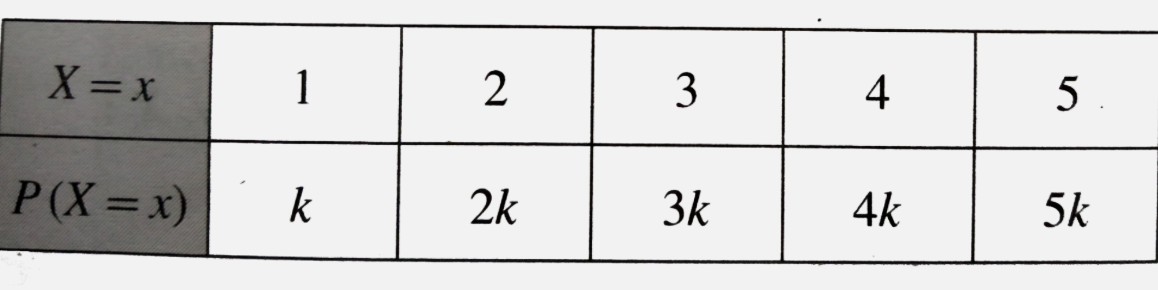
Find (PX≤4)View SolutionThe probability distribution of the random variable X is given by
Then, the value of V(X) is equal to
 View Solution
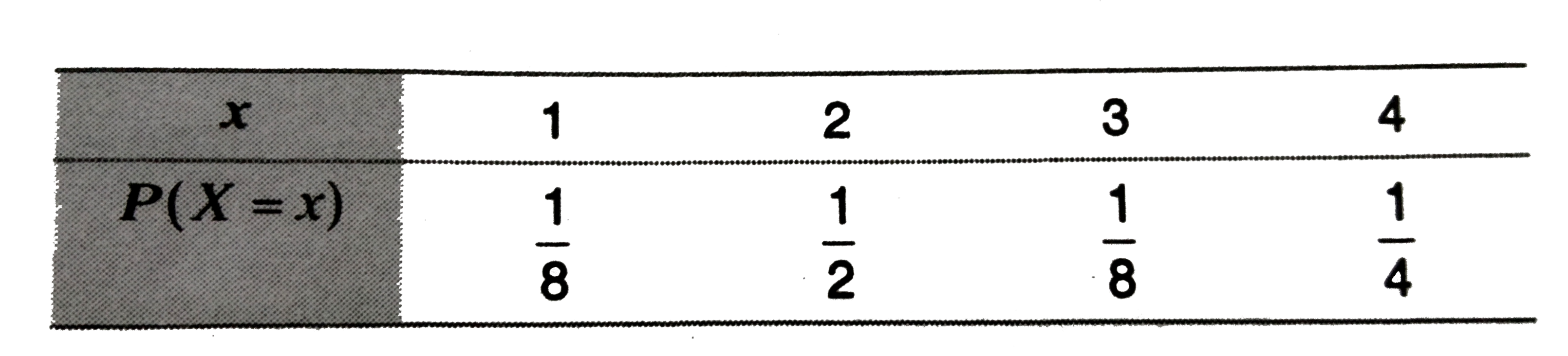
View SolutionThe probability distribution of a random variable X is given as follows:
If E(X)=2.3 , then value of k isView SolutionThe probability distribution of a random variable is as follows: The variance of x is
View SolutionConsider the probability distribution of a random variable X.

Variance of XView SolutionThe probability distribution of a random variable x is given below: What is the standard deviation of x?
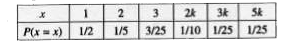
View SolutionThe probability distribution of a discrete r. v. X is as follows.

Find the value of k.View Solution