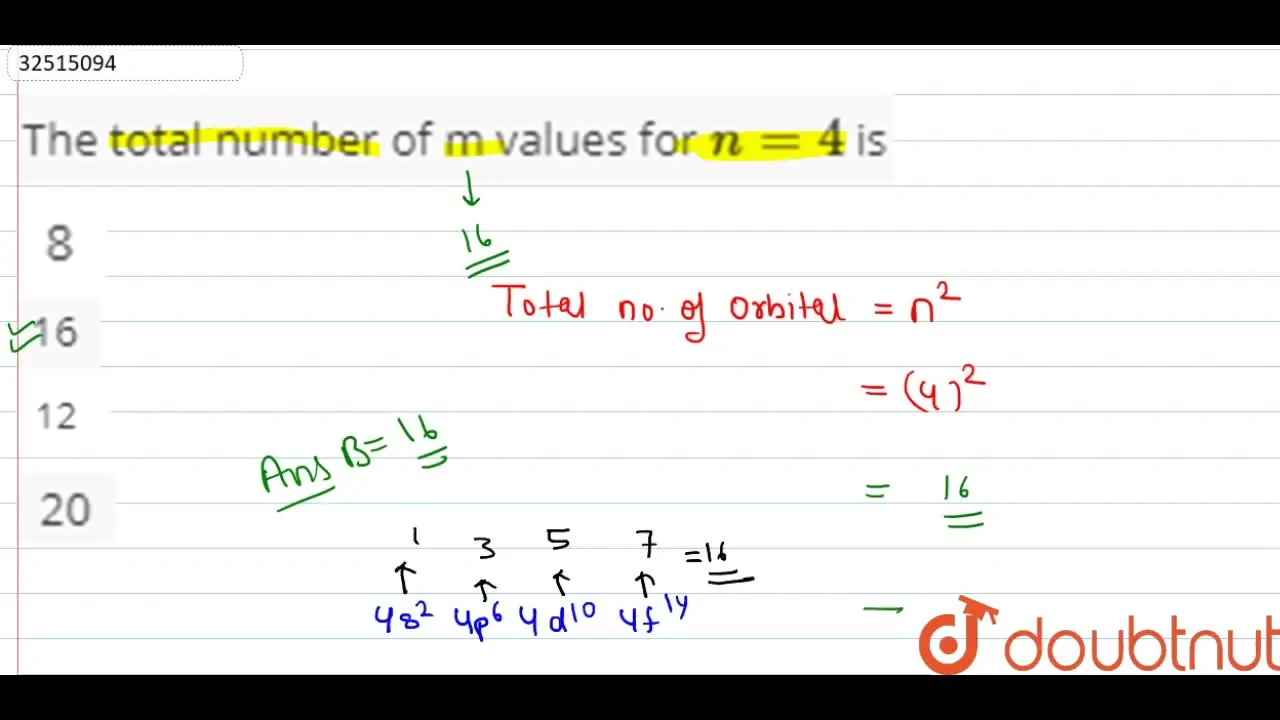
The total number of m values for n=4 is
8
16
12
20
The correct Answer is:B
no of orbitals =n2
Related Playlists
Similar Questions
Consider the equation x2+2x−n=0m where n∈N and n∈[5,100]. The total number of different values of n so that the given equation has integral roots is
View SolutionTotal number of DEB value in :
 View Solution
View SolutionIf N=123×34×52, then the total number of even factors of N is
View SolutionFor a given value of 'l' the total number of 'm' values is ____________
View SolutionTwo finite sets have m and n elements. The total number of subsets of the first set is 56 more than the total number of subsets of the second set. The value of m and n is
View SolutionTwo finite sets have m and n elements, respectively. The total number of subsets of first set is 56 more than the total number of subsets of the second set. The value of m and n respectively are
View SolutionCalculate the total number of electrons for n=3,l=2andm=−2
View SolutionCalculate the total number of electrons for Mn having n+l+m=2
View SolutionThe total number of valence electrons in 4.2g of N−3 ion are :
View Solution(l and m are resecctively the azimuthal and megentic quantum numbers )
column−Icolumn-II(a)total number of values of (l) for a shell(p)0,1,....(n−1)(b)values of (l) for a shell (q)+l....,+2,+1,0,−1,−2..,−1(c)total number of values of (m) for a subshell (r)(2l+1)(d)values of (m) for a subshell(s)nView Solution