A sphere of ice at 0∘C having initial radius R is placed in an environment having ambient temperature >0∘C. The ice melts uniformly, such that shape remains spherical. After a time 't' the radius of the sphere has reduced to r. which graph best depicts r(t)
_E01_119_O01.png)
_E01_119_O02.png)
_E01_119_O03.png)
_E01_119_O04.png)
The correct Answer is:B
To solve the problem of how the radius r(t) of a melting ice sphere changes over time, we can follow these steps:
Step 1: Understand the Problem
We have a sphere of ice at 0∘C with an initial radius R. It is placed in an environment with a temperature greater than 0∘C. As the ice melts uniformly, it retains its spherical shape. We need to find out how the radius r changes with time t.
Step 2: Apply Heat Transfer Principles
The melting of the ice sphere can be analyzed using Newton's law of cooling, which states that the rate of heat transfer is proportional to the temperature difference between the object and its surroundings. The heat loss from the sphere can be expressed as:
dQdt=−σA(T4−T40)
where:
- σ is the Stefan-Boltzmann constant,
- A is the surface area of the sphere,
- T is the temperature of the sphere,
- T0 is the ambient temperature.
Step 3: Calculate the Surface Area
The surface area A of a sphere with radius r is given by:
A=4πr2
Step 4: Relate Heat Loss to Mass Loss
The heat loss can also be related to the mass loss of the ice, which can be expressed as:
dQdt=dmdt⋅L
where L is the latent heat of fusion of ice, and m is the mass of the ice.
Step 5: Express Mass in Terms of Radius
The mass m of the ice can be expressed as:
m=ρV=ρ(43πr3)
where ρ is the density of ice.
Step 6: Differentiate the Mass with Respect to Time
Differentiating the mass with respect to time gives:
dmdt=ρ⋅4πr2drdt
Step 7: Set Up the Equation
Equating the two expressions for heat loss, we have:
−σ(4πr2)(T4−T40)=ρ⋅4πr2drdt⋅L
Step 8: Simplify the Equation
We can cancel 4πr2 from both sides (assuming r≠0):
−σ(T4−T40)=ρLdrdt
Step 9: Rearrange and Integrate
Rearranging gives:
drdt=−σ(T4−T40)ρL
This indicates that the radius decreases over time. Integrating this equation will yield the relationship between r and t.
Step 10: Determine the Graph
The relationship derived indicates that r(t) is a linear function with a negative slope. This means that as time increases, the radius decreases linearly. The graph of r(t) will be a straight line that starts at R and decreases over time.
Conclusion
The graph that best depicts r(t) is a straight line with a negative slope, indicating that the radius decreases uniformly over time.
We have a sphere of ice at 0∘C with an initial radius R. It is placed in an environment with a temperature greater than 0∘C. As the ice melts uniformly, it retains its spherical shape. We need to find out how the radius r changes with time t.
The melting of the ice sphere can be analyzed using Newton's law of cooling, which states that the rate of heat transfer is proportional to the temperature difference between the object and its surroundings. The heat loss from the sphere can be expressed as:
dQdt=−σA(T4−T40)
where:
- σ is the Stefan-Boltzmann constant,
- A is the surface area of the sphere,
- T is the temperature of the sphere,
- T0 is the ambient temperature.
The surface area A of a sphere with radius r is given by:
A=4πr2
The heat loss can also be related to the mass loss of the ice, which can be expressed as:
dQdt=dmdt⋅L
where L is the latent heat of fusion of ice, and m is the mass of the ice.
The mass m of the ice can be expressed as:
m=ρV=ρ(43πr3)
where ρ is the density of ice.
Differentiating the mass with respect to time gives:
dmdt=ρ⋅4πr2drdt
Equating the two expressions for heat loss, we have:
−σ(4πr2)(T4−T40)=ρ⋅4πr2drdt⋅L
We can cancel 4πr2 from both sides (assuming r≠0):
−σ(T4−T40)=ρLdrdt
Rearranging gives:
drdt=−σ(T4−T40)ρL
This indicates that the radius decreases over time. Integrating this equation will yield the relationship between r and t.
The relationship derived indicates that r(t) is a linear function with a negative slope. This means that as time increases, the radius decreases linearly. The graph of r(t) will be a straight line that starts at R and decreases over time.
The graph that best depicts r(t) is a straight line with a negative slope, indicating that the radius decreases uniformly over time.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -03
11 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - ASSERTION-REASON
40 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -01
65 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
427 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A sphere of radius 0.350m, temperature 27.0∘C , and emissivity 0.850 is located in an environment of temperature 77.0∘C. At what rate does the sphere (a) emit and (b) absorb thermal radiation ? (c ) What is the sphere's net change in energy in 3.50 min ?
View Solutionblack colored solid sphere of radius "R" and mass "M" is inside a cavity with vacuum inside.The walls of the cavity are maintained at temperature T0 .The initial temperature of the sphere is 3T0 .If the specific heat of the material of the sphere varies as αT3 per unit mass with the temperature of the sphere,where α is a constant,then the time taken for the sphere to cool down to temperature 2T0 will be ( σ Stefan Boltzmann constant)
View Solution
Knowledge Check
A solid sphere of radius 'R' density ρ and specific heat 'S' initially at temperature T0 Kelvin is suspended in a surrounding at temperature 0K. Then the time required to decrease the temperature of the sphere from T0 to T02 kelvin is (Assume sphere behaves like a black body)
AρSR9σT30B5ρSR9σT30C7ρSR9σT30D8ρSR9σT30Three solid spheres have their radii r1,r2andr3 .The spheres are melted to form a solid sphere of bigger radius of the new sphere is:
A(r1+r2+r3)B(r21+r22+r23)12C(r31+r32+r33)13D(r41+r42+r43)14A spherical conductor having charge q and radius r is placed at the centre of a spherical shell of radius R and having charge Q(R>r0. The potential difference between the two is
AProportional to QBProportional to qCDependent on both Q and qDIndependent of both Q and q
A solid copper sphere of density ρ, specific heat c and radius r is at temperature T1. It is suspended inside a chamber whose walls are at temperature 0K. What is the time required for the temperature of sphere to drop to T2? Take the emmissivity of the sphere to be equal to e.
View SolutionA spherical shell of radius r carries a uniformly distributed surface charge q on it. A hemispherical shell of radius R(gt r) is placed covering it with its centre coinciding with that of the sphere of radius r. The hemisphere has a uniform surface charge Q on it. The charge distribution on the sphere and the hemisphere is not affected due to each other. Calculate the force that the sphere will exert on the hemisphere.
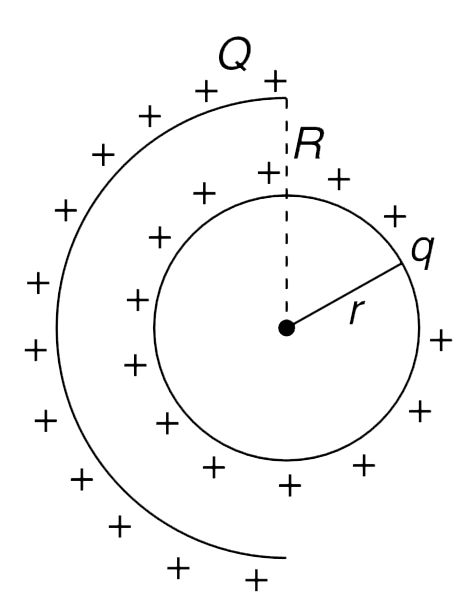
View SolutionA solid sphere of radius 'R' density 'ρ' , and specific heat 'S' initially at temperature T0 Kelvin is suspended in a surrounding at temperature 0 K. Then the time required to decrease the temperature of the sphere from T0 to T02 Kelvin is α×ρSRσT30 . Find α. (Assume sphere behaves like a black body )
View SolutionThe ice with the initial temperature t1=0∘C was first melted, then heated to the temperature t2=100∘C and evaporated. Find the increment of the system's specific entropy.
View SolutionA solid sphere of radius r is melted and cast into the shape of a solid cone of height r , the radius of the base of the cone is 2r (b) 3r (c) r (d) 4r
View Solution
