Three rods of equal of length are joined to from an equilateral triangle ABC. $D$ is the midpoint of AB. The coefficient of linear expansion is $α_{1}$ for AB and $α_{2}$ for $A C$ and $B C$ . If the distance $D C$ remains constant for small changes in temperature,

$α_{1} = α_{2}$

$α_{1} = 2 α_{2}$

$α_{1} = 4 α_{2}$

$α_{1} = \frac{1}{2} α_{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

An equilateral triangle $A B C$ is formed by joining three rods of equal length and $D$ is the mid-point of $A B$ . The coefficient of linear expansion for $A B$ is $α_{1}$ and for $A C$ and $B C$ is $α_{2}$ . The relation between $α_{1}$ and $α_{2}$ , if distance $D C$ remains constant for small changes in temperture is

A $α_{1} = α_{2}$
B $α_{1} = 4 α_{2}$
C $α_{2} = 4 α_{1}$
D $α_{1} = \frac{1}{2} α_{2}$
Two rods , one of aluminium and the other made of steel, having initial length $l_{1}$ and $l_{2}$ are connected together to from a sinlge rod of length $l_{1} + l_{2}$ . The coefficient of linear expansion for aluminium and steel are $α_{a}$ and $α_{s}$ for $A C$ and $B C$ . If the distance $D C$ remains constant for small changes in temperature,
A $\frac{α_{s}}{α_{a}}$
B $\frac{α_{a}}{α_{s}}$
C $\frac{α_{s}}{(α_{a} + α_{s})}$
D $\frac{α_{a}}{(α_{a} + α_{s})}$
Three rods of equal l are joined to from an equilateral triangle PQR.O is the mid-point of PQ. Distance OR remains same for small change in temperature. Coefficient of linear expansion for PR and RQ is same i.e., $α_{2}$ but for PQ is $α_{1^{.}}^{.}$ Then

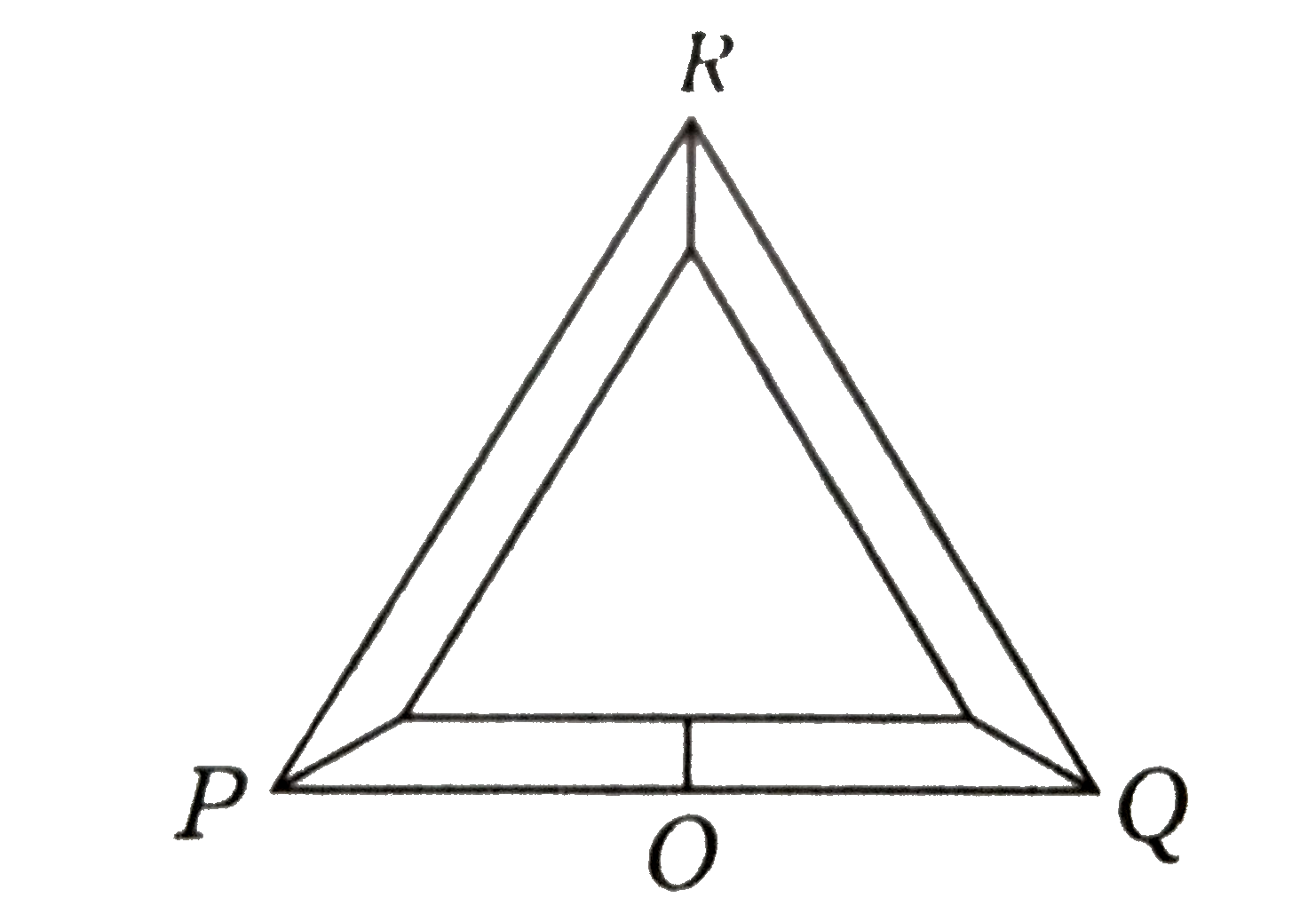A $α_{2} = 3 α_{1}$
B $α_{2} = 4 α_{1}$
C $α_{1} = 3 α_{2}$
D $α_{1} = 4 α_{2}$