Two forces →F1=(3N)ˆi−(4N)ˆj and →F2=−(1N)ˆi−(2N)ˆj act on a point object. In the given figure which of the six vectors represents →F(1) and →F2 and what is the magnitude of the net forces

4 and 8, sqrt(19)N
3 and 6,5N
5 and 7, √40N
2 and 6,7 N
The correct Answer is:C
→F1=(3N)ˆi−(4N)ˆj
This means (3N) along +x axis and (4N) along negative side of y axis. Hence vector 5 represents it.
→F2=−(1N)ˆi−(2N)ˆj
This means (1N) along negative x-axis. (2N) along negative y-axis.
hence force
|F|2=(2)2+(−6)2=40
∴|F|=√40N
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-II
Explore 112 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-III
Explore 28 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:SWOE
Explore 44 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
Explore 25 Videos
Similar Questions
Two forces →F1=5ˆi+10ˆj−20ˆkand→F2=10ˆi−5ˆj−15ˆk act on a single point. The angle between →F1and→F2 is nearly
View SolutionTwo forces →F1=2ˆi+2ˆjNand→F2=3ˆi+4ˆkN are acting on a particle
(a) Find the resultant force acting on particle
(b) (b) Find the angle between−→F1&−→F2
(c) Find the componant of force −→F1 along force −→F2.
View Solution
Knowledge Check
The resultant of the forces →F1=4ˆi−3ˆj and →F2=6ˆi+8ˆj is
A5√5B10ˆi−5ˆjC125D−2ˆi−3ˆjTwo forces →F1and→F2 are acting at right angles to each other, find their resultant ?
A√F21+F22B√F21−F22CF1+F2DF1−F2Consider two vectors →F1=2ˆi+5ˆk and →F2=3ˆj+4ˆk. The magnitude to thhe scalar product of these vectors is
A20B23C5√33D26
Three forces →F1=(2ˆi+4ˆj)N:→F2=(2ˆj−ˆk)N and →F3=(ˆk−4ˆi−2ˆj)N are applied on an object of mass 1 kg at rest at origin. The position of the object at t = 2s will be
View SolutionIf the four forces as shown are in equilibrium Express →F1&→F2 in unit vector form.
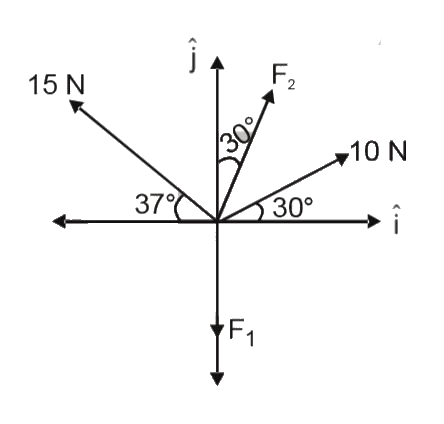
View SolutionWhich of the four arrangements in the figure correctly shows the vector addition of two forces →F1 and →F2 to yield the third force →F3
View SolutionIf force (→F)=4ˆi+4ˆj and displacement (→s)=3ˆi+6ˆk then the work done is
View SolutionThree force F1=(3ˆi+2ˆj−ˆk) →F2 and that the particle may be in equilibrum , the value of 'a' is :
View Solution