If a continous random variable f(x)=kx2+16−∞<x<∞ then the value of k is Solution in Tamil
π4
π2
3π
4PI
The correct Answer is:d
Step by step video, text & image solution for If a continous random variable f(x)=(k)/(x^(2)+16)-inftyltxltinfty then the value of k is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONSBOOK - PREMIERS PUBLISHERSCHAPTER - PROBABILITY DISTRIBUTIONSEXERCISE - EXERCISE 11.6
20 Videos
- ORDINARY DIFFERENTIAL EQUATIONS BOOK - PREMIERS PUBLISHERSCHAPTER - ORDINARY DIFFERENTIAL EQUATIONS EXERCISE - PROBLEMS FOR PRACTICE (ANSWER THE FOLLOWING QUESTIONS )
22 Videos
- THEORY OF EQUATIONSBOOK - PREMIERS PUBLISHERSCHAPTER - THEORY OF EQUATIONSEXERCISE - Problems for practice (Answer the following)
21 Videos
Similar Questions
Let X be random variable with probability density function
f(x)={2x3x≥10x<1
Which of the following statement is correct
View SolutionFind the order and degree of the following differential equation
y''=(2+y')34
View SolutionIf α and β are the roots of 2x2−5x+3=0 form the equation whose roots are α2 and β2
View SolutionLet a random variable X have a binomial distribution with mean 8 and variance 4. If P(X≤2)=k216, then k is equal to :
View SolutionLet a random variable X have a binomial distribution with mean 8 and variance 4. If P(X≤2)=k216, then k is equal to
View SolutionLet X is a continuous random variable with probability density function
f(x)={x6+k0≤x≤30 otherwise
The value of k is equal to
View Solutionc.d.f F(x) of a continous random variable X is defined as .
View Solution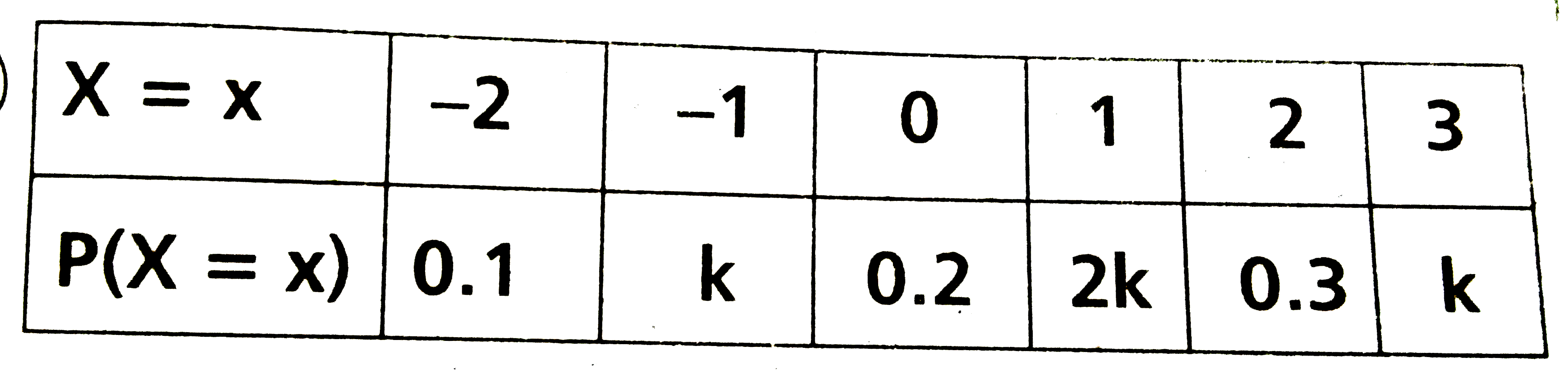
is the probability distribution of a random variable X. Find the value of K and the variance of X.
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5 then the probability that X takes a prime value is
View SolutionFor what value of k will the function f(x) =kx x=1,2,3 ...., n be a probability distribution of a random variable ?
View SolutionF(X)=π2+tan−1xπ−∞<x<∞ is a distribution of a continous random variable X then P(X≤1) is
View SolutionIfF(x)=Aπ=116+x2−∞<x<∞ is a pdf of a continous random variable x then the value of A is
View SolutionIf f(x)=k(2x−x2),0≤x≤1 is probability density function of a continous random variable x then k is
View Solutionमाना एक यादृच्छिक चर x के द्विपद बंटन का माध्य 8 तथा प्रसरण 4 है। यदि p(X≤2)=k216 है, तो k बराबर है
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5, then the probability that X takes a prime value is
View Solution
