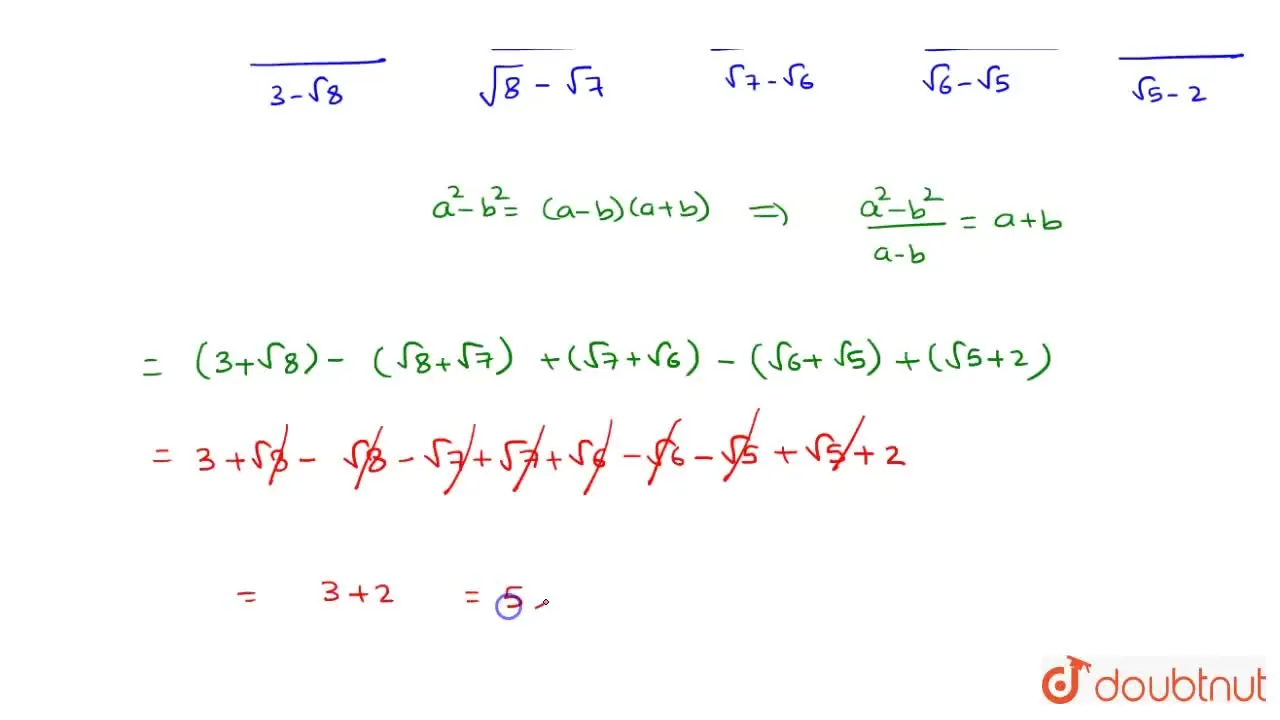
Show that:1(3−√8)−1(√8−√7)+1(√7−√6)−1(√6−√5)+1(√5−2)=5
Video Solution
|
Answer
Step by step video, text & image solution for Show that:1/((3-sqrt8))-1/((sqrt8-sqrt7))+1/((sqrt7-sqrt6))-1/((sqrt6-sqrt5))+1/((sqrt5-2))=5 by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE TEST QUESTION PAPER 1EXERCISE - SECTION-C6videos
PRACTICE TEST QUESTION PAPER 1
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE QUESTION PAPER-2EXERCISE - PART D7videos
PRACTICE QUESTION PAPER-2
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICES QUESTION PAPER -3EXERCISE - PART D5videos
PRACTICES QUESTION PAPER -3
Similar Questions
(13−√8−1√8−√7)
View SolutionProve that: 13−√8−1√8− √7+1√7− √6−1√6− √5+1√5−2=5
View SolutionShow that: 13−√8−1√8−√7+1√7−√6−1√6−√5+1√5−2=5
View Solution13−√8−1√8−√7+1√7−√6−1√6−√5+1√5−2=5
View SolutionProve that: 13−√8−1√8− √7+1√7− √6−1√6− √5+1√5−2=5
View Solution13−√8−1√8−√7+1√7−√6−1√6−√5+1√5−2=
View SolutionProve that : 13−√8−1√8−√7+1√7−√6)−1√6−√5+1√5−2=5
View SolutionLet T=13−√8−1√8−√7+1√7−√6−1√6−√5+1√5+2 then-
View SolutionThe value of [1√9−√8]−[1√8−√7]+[1√7−√6]−[1√6−√5]+[1√5−√4] is
A)6
B)5
C)-7
D)-6View Solution1√9−√8−1√8−√7+1√7−√6−1√6−√5+1√5−√4=?
View Solution