A literacy caompaign was organised by Class IX girl students under NSS. Students made (x-5) row and (3x-4) columns for the rally. Write the total number of students in the form of a polynomial.
The correct Answer is:3x2−19x+20
To find the total number of students in the form of a polynomial, we need to multiply the number of rows by the number of columns.
1. Identify the expressions for rows and columns:
- The number of rows is given as x−5.
- The number of columns is given as 3x−4.
2. Set up the expression for total students:
- The total number of students can be expressed as:
Total Students=Rows×Columns=(x−5)(3x−4)
3. Use the distributive property (FOIL method):
- We will multiply the two binomials:
(x−5)(3x−4)=x⋅3x+x⋅(−4)+(−5)⋅3x+(−5)⋅(−4)
4. Calculate each term:
- First term: x⋅3x=3x2
- Second term: x⋅(−4)=−4x
- Third term: −5⋅3x=−15x
- Fourth term: −5⋅(−4)=20
5. Combine like terms:
- Now combine all the terms:
3x2−4x−15x+20=3x2−19x+20
6. Write the final polynomial:
- Therefore, the total number of students in the form of a polynomial is:
3x2−19x+20
Final Answer:
The total number of students is represented by the polynomial 3x2−19x+20.
- The number of rows is given as x−5.
- The number of columns is given as 3x−4.
- The total number of students can be expressed as:
Total Students=Rows×Columns=(x−5)(3x−4)
- We will multiply the two binomials:
(x−5)(3x−4)=x⋅3x+x⋅(−4)+(−5)⋅3x+(−5)⋅(−4)
- First term: x⋅3x=3x2
- Second term: x⋅(−4)=−4x
- Third term: −5⋅3x=−15x
- Fourth term: −5⋅(−4)=20
- Now combine all the terms:
3x2−4x−15x+20=3x2−19x+20
- Therefore, the total number of students in the form of a polynomial is:
3x2−19x+20
The total number of students is represented by the polynomial 3x2−19x+20.
Topper's Solved these Questions
- POLYNOMIALSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - POLYNOMIALSEXERCISE - Practice Test
8 Videos
- POLYNOMIALSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - POLYNOMIALSEXERCISE - True & False
22 Videos
- NUMBER SYSTEMSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - NUMBER SYSTEMSEXERCISE - Part - D
18 Videos
- PRACTICE QUESTION PAPER-2BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRACTICE QUESTION PAPER-2EXERCISE - PART D
7 Videos
Similar Questions
Knowledge Check
A teacher wants to keep his students an equal number in rows and columns the total number of students is 13 then find the number of students in last row?
A37B33C63D47A total of 1152 students were assembled in rows and colums. If there are n rows and n/2 columns,then find the number of students in each row.
A36B42C48D34There are 'b' boys and 'g' girls in a class. The ratio of the number of boys to the total number of students in the class is:
Abb+gBgb+gCbgDb+gb
There are 25 boys and 25 girls in a class. The ratio of the number of boys to the total number of students is
View SolutionThe given bar graph, shows the number of students in different classes.
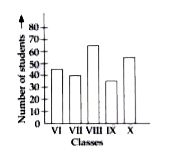
Find the total number of students in the classes VIII to X.
View SolutionThere are ‘b’ boys and ‘g’ girls in a class. The ratio of the number of boys to the total number of students in the class is:
View SolutionIn a class there are 32 girls. If the fraction of girls in the class is (8)/(11) ,find the total number of students in the class.
View SolutionIn a class of 50 students, 15 are girls. 5 of the girls and 27 of the boys were chosen torepresent their class in a game. The total number of students chosen is
View Solution
