Calculate the focal length of concave len in water (μw=43) if the surface have radii equal to 60cm and 20cm. μg=1.5
To calculate the focal length of a concave lens in water, we will use the Lensmaker's formula. The formula is given by:
1f=(μ2μ1−1)(1R1−1R2)
Where:
- f is the focal length of the lens,
- μ1 is the refractive index of the medium in which the lens is placed (water in this case),
- μ2 is the refractive index of the lens material (glass),
- R1 is the radius of curvature of the first surface,
- R2 is the radius of curvature of the second surface.
Given Data:
- Refractive index of water, μw=43
- Refractive index of glass, μg=1.5
- Radius of curvature of the first surface, R1=60cm
- Radius of curvature of the second surface, R2=20cm
Step 1: Identify the values for the formula
In our case:
- μ1=μw=43
- μ2=μg=1.5
- R1=60cm (since it is a concave lens, we take this as negative, so R1=−60cm)
- R2=20cm (this is positive)
Step 2: Substitute the values into the Lensmaker's formula
Now substituting these values into the Lensmaker's formula:
1f=⎛⎝1.543−1⎞⎠(1−60−120)
Step 3: Simplify the equation
Calculating 1.543:
1.543=1.5×34=4.54=98
Now substituting back into the formula:
1f=(98−1)(1−60−120)
Calculating 98−1:
98−1=98−88=18
Now calculating 1−60−120:
1−60−120=−160−360=−460=−115
Step 4: Combine the results
Now substituting these results back into the formula:
1f=(18)(−115)
Calculating this gives:
1f=−1120
Step 5: Find the focal length
Taking the reciprocal gives us the focal length:
f=−120cm
Conclusion
The focal length of the concave lens in water is −120cm.
- f is the focal length of the lens,
- μ1 is the refractive index of the medium in which the lens is placed (water in this case),
- μ2 is the refractive index of the lens material (glass),
- R1 is the radius of curvature of the first surface,
- R2 is the radius of curvature of the second surface.
- Refractive index of water, μw=43
- Refractive index of glass, μg=1.5
- Radius of curvature of the first surface, R1=60cm
- Radius of curvature of the second surface, R2=20cm
In our case:
- μ1=μw=43
- μ2=μg=1.5
- R1=60cm (since it is a concave lens, we take this as negative, so R1=−60cm)
- R2=20cm (this is positive)
Now substituting these values into the Lensmaker's formula:
Calculating 1.543:
Now substituting these results back into the formula:
Taking the reciprocal gives us the focal length:
The focal length of the concave lens in water is −120cm.
Similar Questions
Knowledge Check
What is the focal length of double convex lens for which radius of curvature of either of the surfaces is 30 cm? [aμg=1.5]
A50 cmB30 cmC−30cmD−50cmAssertion : A double convex lens (μ=1.5) has focal length 10cm. When the lens is immersed in water (μ=4/3) its focal length becomes 40cm.
Reason : 1f=μ1−μmμm(1R1−1R2)AIf both the assertion and reason are true and reason explains the assertion.BIf both the assertion and reason are true but reason does not explain the assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true.A concave mirror and a converging lens (glass with μ=1.5) both have a focal length of 3 cm when in air. When they are in water (μ=43), their new focal length are
AfLens=12cm,fMirror=3cmBfLens=3cm,fMirror=12cmCfLens=3cm,fMirror=3cmDfLens=12cm,fMirror=12cm
A thin lens of focal length + 12 cm is immersed in water (μ=1.33). What is its new focal length ?
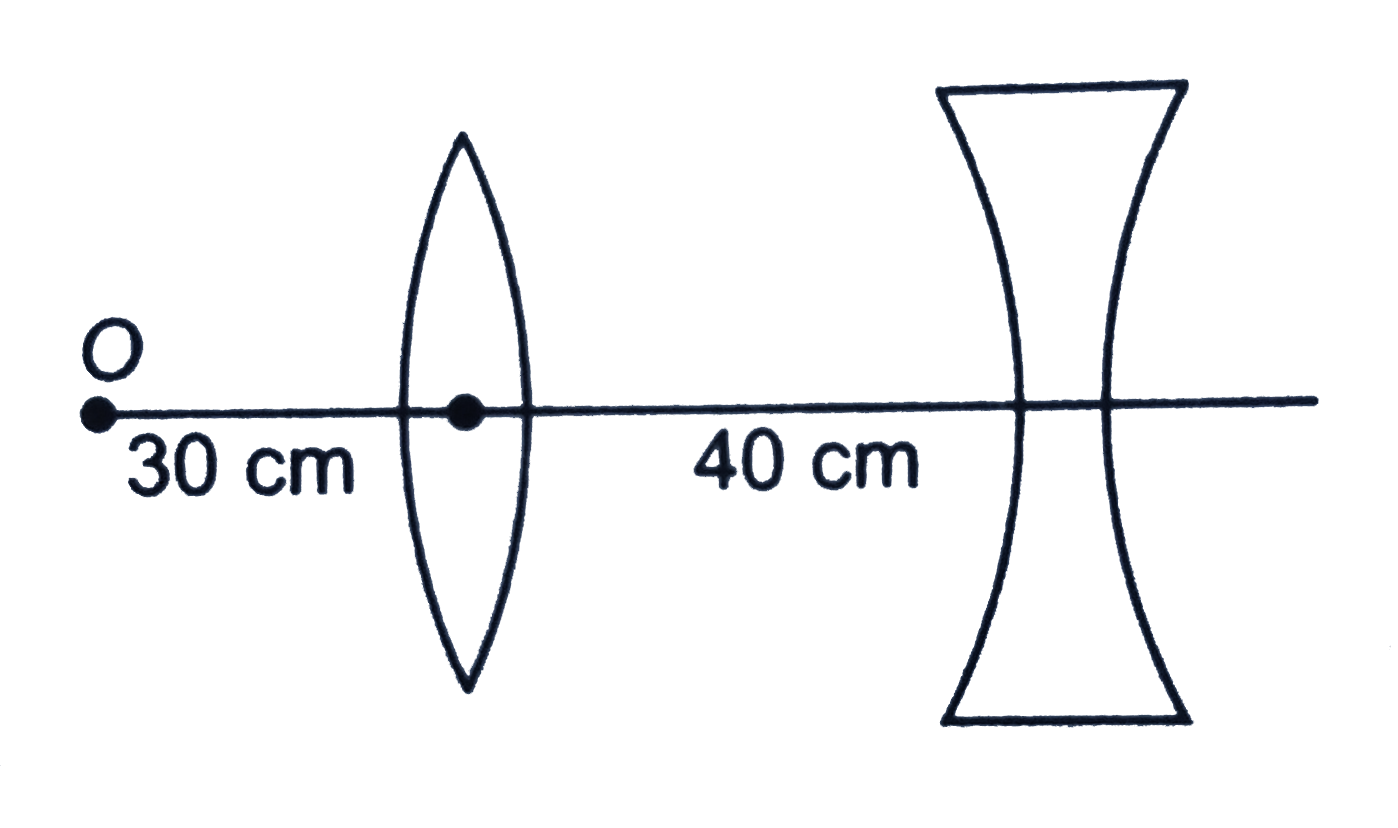
View SolutionFocal length of a convex lens in air is 20cm. and of concave lens 40cm. Find the position of final image.
View SolutionThe two surfaces of a double concave lens are of curvature 10 cm and 40 cm. Find the focal length of the lens in water. The refractive index of water is 4/3 and that of the lens of the material is 3/2.
View SolutionThe focal length of a planoconvex glass lens is 20 cm (μg=1.5). The plane face of it is silvered. An illuminating object is placed at a distance of 60 cm from the lens on its axis along the convex side. Then the distance (in cm) of the image is
View SolutionA biconvex thin lens is prepared from glass of refractive index μ2=32. The two conducting surfaces have equal radii of 20 cm each. One of the the surface is silvered from outside to make it reflecting. It is placed in a medium of refractive index μ1=53. It acts as a
View Solution