In a metre bridge, the length of the wire is 100 cm. At what position will the balance point be obtained if the two resistances are in the ratio 2 : 3?
The correct Answer is:40cm
To solve the problem of finding the balance point on a metre bridge when the two resistances are in the ratio of 2:3, we can follow these steps:
1. Understand the Setup:
A metre bridge consists of a wire of length 100 cm. When two resistances P and Q are connected at either end of the bridge, a jockey is used to find the balance point along the wire.
2. Define the Balance Point:
Let the balance point be at a distance x cm from one end of the bridge. Consequently, the remaining length from the other end will be 100−x cm.
3. Use the Principle of the Meter Bridge:
According to the principle of the metre bridge, the ratio of the resistances is equal to the ratio of the lengths of the wire on either side of the jockey at the balance point:
PQ=x100−x
4. Substitute the Given Ratio:
We know that the resistances are in the ratio PQ=23. Therefore, we can substitute this into our equation:
23=x100−x
5. Cross-Multiply to Solve for x:
Cross-multiplying gives:
2(100−x)=3x
Expanding this:
200−2x=3x
6. Rearrange the Equation:
Combine like terms:
200=3x+2x
200=5x
7. Solve for x:
Divide both sides by 5:
x=2005=40 cm
Final Answer:
The balance point will be obtained at 40 cm from one end of the metre bridge.
A metre bridge consists of a wire of length 100 cm. When two resistances P and Q are connected at either end of the bridge, a jockey is used to find the balance point along the wire.
Let the balance point be at a distance x cm from one end of the bridge. Consequently, the remaining length from the other end will be 100−x cm.
According to the principle of the metre bridge, the ratio of the resistances is equal to the ratio of the lengths of the wire on either side of the jockey at the balance point:
PQ=x100−x
We know that the resistances are in the ratio PQ=23. Therefore, we can substitute this into our equation:
23=x100−x
Cross-multiplying gives:
2(100−x)=3x
Expanding this:
200−2x=3x
Combine like terms:
200=3x+2x
200=5x
Divide both sides by 5:
x=2005=40 cm
The balance point will be obtained at 40 cm from one end of the metre bridge.
Similar Questions
In a meter bridge, the length of the wire is 100 cm. At what position will the balance point be obtained if the two resistances are in the ratio 1:3?
View SolutionIn a metre bridge the length of the wire is 100 cm. At what point will the balance point be obtained if the two resistances are in the ratio of 4:5.
View Solution
Knowledge Check
In a Wheatstone bridge resistance connected the bridge is balanced , when the resistance are in the ratio
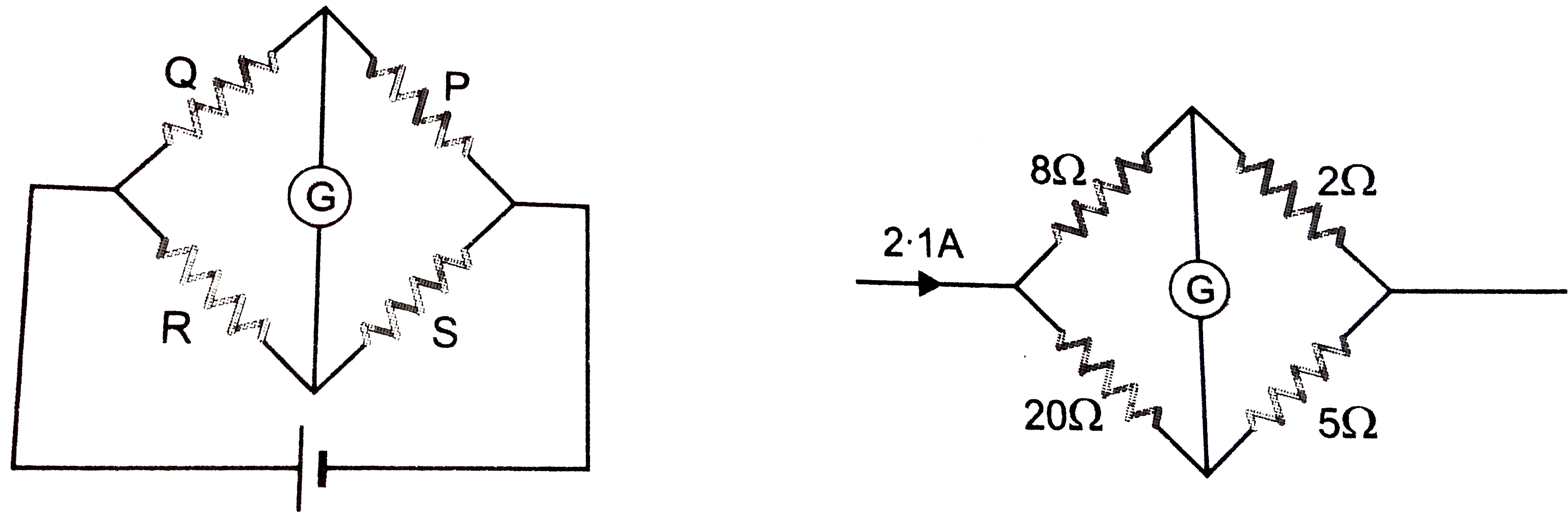 APQ=RSBQP=SRCPQ=RSDPQ=SR
APQ=RSBQP=SRCPQ=RSDPQ=SRA resistance of 2Ω is connected across one gap of a meter bridge (the length of the wire is 100cm) and an unknown resistance, greater than 2Ωis conneted across the other gap. When these resistances are interchanged, the balance point shifts by 20cm. Neglecting any corrections, the unknown resistance is
A3ΩB4ΩC5ΩD6ΩA resistance of 2Ω is connected across one gap of a metre-bridge(the length of the wire is 100 cm) and an unknown resistance, greater than 2Ω, is connected across the other gap. When these resistances are interchanged, the balance points shifts by 20 cm. Neglecting any corrections, the unknown resistance is
A3ΩB4ΩC5ΩD6Ω
In a metre bridge expt, when the resistances in the gaps are interchanged the balance point is increases by 10 cm. The ratio of the resistances is
View SolutionIn a metre bridge, the gaps are closed by two resistance P and Q and the balance point is obtained at 40cm. When Q is shunted by a resistance of 10Ω, the balance point shifts to 50cm. The values of P and Q are.
 .
.
View SolutionIn a metre bridge, the gaps are closed by two resistance P and Q and the balance point is obtained at 40cm. When Q is shunted by a resistance of 10Ω, the balance point shifts to 50cm. The values of P and Q are.
 .
.
View SolutionIn the metre bridge, the balancing length AB = 20 cm. The unknown resistance X is equal to
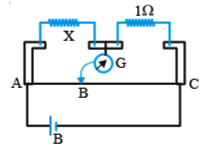
View SolutionIn a meter bridge experiment the ratio of left gap resistance to right gap resistance is 2:3 the balance point from is
View Solution
