An ideal solenoid of cross-sectional area 10−4m2 has 500 turns per metre. At the centre of this solenoid, another coil of 100 turns is wrapped closely around it. If the current in the coil changes from 0 to 2 A in 3.14 ms, the emf developed in the second coil is
1 mv
2 mv
3 mv
4 mv
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Understand the given parameters
- Cross-sectional area of the solenoid, A=10−4m2
- Number of turns per unit length of the solenoid, n=500turns/m
- Number of turns in the coil wrapped around the solenoid, N=100
- Change in current, ΔI=I2−I1=2A−0A=2A
- Time interval for the change in current, Δt=3.14ms=3.14×10−3s
Step 2: Calculate the magnetic field inside the solenoid
The magnetic field B inside an ideal solenoid is given by the formula:
B=μ0nI
Where μ0=4π×10−7T⋅m/A is the permeability of free space.
Step 3: Find the change in magnetic field due to the change in current
The change in magnetic field ΔB when the current changes from 0 to 2A is:
ΔB=μ0nΔI
Step 4: Calculate the magnetic flux Φ through the coil
The magnetic flux Φ linked with the coil is given by:
Φ=N⋅B⋅A
Thus, the change in magnetic flux ΔΦ is:
ΔΦ=N⋅ΔB⋅A
Step 5: Apply Faraday's Law to find the induced EMF
According to Faraday's Law, the induced EMF E is given by:
E=−dΦdt
Since we are interested in the average EMF during the time interval Δt:
E=−ΔΦΔt
Step 6: Substitute the values and calculate
1. Calculate ΔB:
ΔB=μ0nΔI=(4π×10−7)⋅(500)⋅(2)=4π×10−7⋅1000
ΔB=4π×10−4T
2. Calculate ΔΦ:
ΔΦ=N⋅ΔB⋅A=100⋅(4π×10−4)⋅(10−4)=4π×10−6Wb
3. Calculate the induced EMF:
E=−4π×10−63.14×10−3=−4π3.14×10−3V
Since π≈3.14, we find that:
E≈−4×10−3V=−4mV
Final Answer
The induced EMF developed in the second coil is approximately 4mV.
---
- Cross-sectional area of the solenoid, A=10−4m2
- Number of turns per unit length of the solenoid, n=500turns/m
- Number of turns in the coil wrapped around the solenoid, N=100
- Change in current, ΔI=I2−I1=2A−0A=2A
- Time interval for the change in current, Δt=3.14ms=3.14×10−3s
The magnetic field B inside an ideal solenoid is given by the formula:
B=μ0nI
Where μ0=4π×10−7T⋅m/A is the permeability of free space.
The change in magnetic field ΔB when the current changes from 0 to 2A is:
ΔB=μ0nΔI
The magnetic flux Φ linked with the coil is given by:
Φ=N⋅B⋅A
Thus, the change in magnetic flux ΔΦ is:
ΔΦ=N⋅ΔB⋅A
According to Faraday's Law, the induced EMF E is given by:
E=−dΦdt
Since we are interested in the average EMF during the time interval Δt:
E=−ΔΦΔt
1. Calculate ΔB:
ΔB=μ0nΔI=(4π×10−7)⋅(500)⋅(2)=4π×10−7⋅1000
ΔB=4π×10−4T
ΔΦ=N⋅ΔB⋅A=100⋅(4π×10−4)⋅(10−4)=4π×10−6Wb
E=−4π×10−63.14×10−3=−4π3.14×10−3V
Since π≈3.14, we find that:
E≈−4×10−3V=−4mV
The induced EMF developed in the second coil is approximately 4mV.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -C) Previous Years Questions
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION - A) (OBJECTIVE TYPE QUESTIONS)
30 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTECHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION -J(Aakash Challengers Questions)
5 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
A toroidal solenoid with an air core has an average radius of 15 cm, area of cross-section 12cm2 and 1200 turns. Obtain the self inductance of the toroid. Ignore field variations across the cross-section of the toroid.
(b) A second coil of 300 turns is wound closely on the toroid above. If the current in the primary coil is increased from zero to 2.0 A in 0.05 s, obtain the induced e.m.f. in the second coil.
View SolutionA long solenoid of diameter 0.1m has 2×104 turns per metre. At the centre of the solenoid, a 100−turns coil of radius 0.01m is placed with its axis coinciding with the constant rate from +2A to 2A in 0.05s. Find the total charge (in μC) flowing through the coil during this time when the resistance of the coil is 40≠2Ω.
View Solution
Knowledge Check
The inductance of a solenoid 0.5m long of cross-sectional area 20cm2 and with 500 turns is
A12.5mHB1.25mHC15.0mHD0.12mHA solenoid has 2000 turns wound over a length of 0.3m. Its cross-sectional area is equal to 1.2×10−3m2. Around its central cross-section a coil of 300 turns in wound. If an initial current of 2A flowing in the solenoid is reversed in 0.25s, the emf induced in the coil is
A0.6mVB60mVC48mVD0.48mVA long solenoid of diameter 0.1 m has 2×104 turns per meter. At centre of the solenoid is 100 turns coil of radius 0.01 m placed with its axis coinciding with solenoid axis. The current in the solenoid reduce at a constant rate to 0A from 4 a in 0.05 s . If the resistance of the coil is 10π2Ω, the total charge flowing through the coil during this time is
A16μCB32μCC15πμCD32πμC
Find the self-inductance of a toroidal solenoid of radius 20 cm, area of cross section 100cm2 and having 500 turns. Assume that the field variations across the cross section of the toroid are negligible. Further, if a secondary coil of 300 turns is wound on this toroid and the current in the primary coil is increased from 2 A to 5 A in 0.05 s. Find the emf induced in the secondary coil.
View SolutionA long solenoid of diameter 0.1m has 2×104 turns per metre. At the centre of the solenoid, a coil of 100 turns and radius 0.01m is placed with its axis coinciding with the solenoid axis. The current in the solenoid reduces at a constant rate to 0A from 4A in 0.05 .If the resistance of the coil is 10π2ohm, the total charge (in μC ) flowing through the coil during this time is
View SolutionA long solenoid of cross-sectional area 5.0cm2 is wound with 25 turns of wire per centimetre. It is placed in the middle of a closely wrapped coil of 10 turns and radius 25 cm as shown.
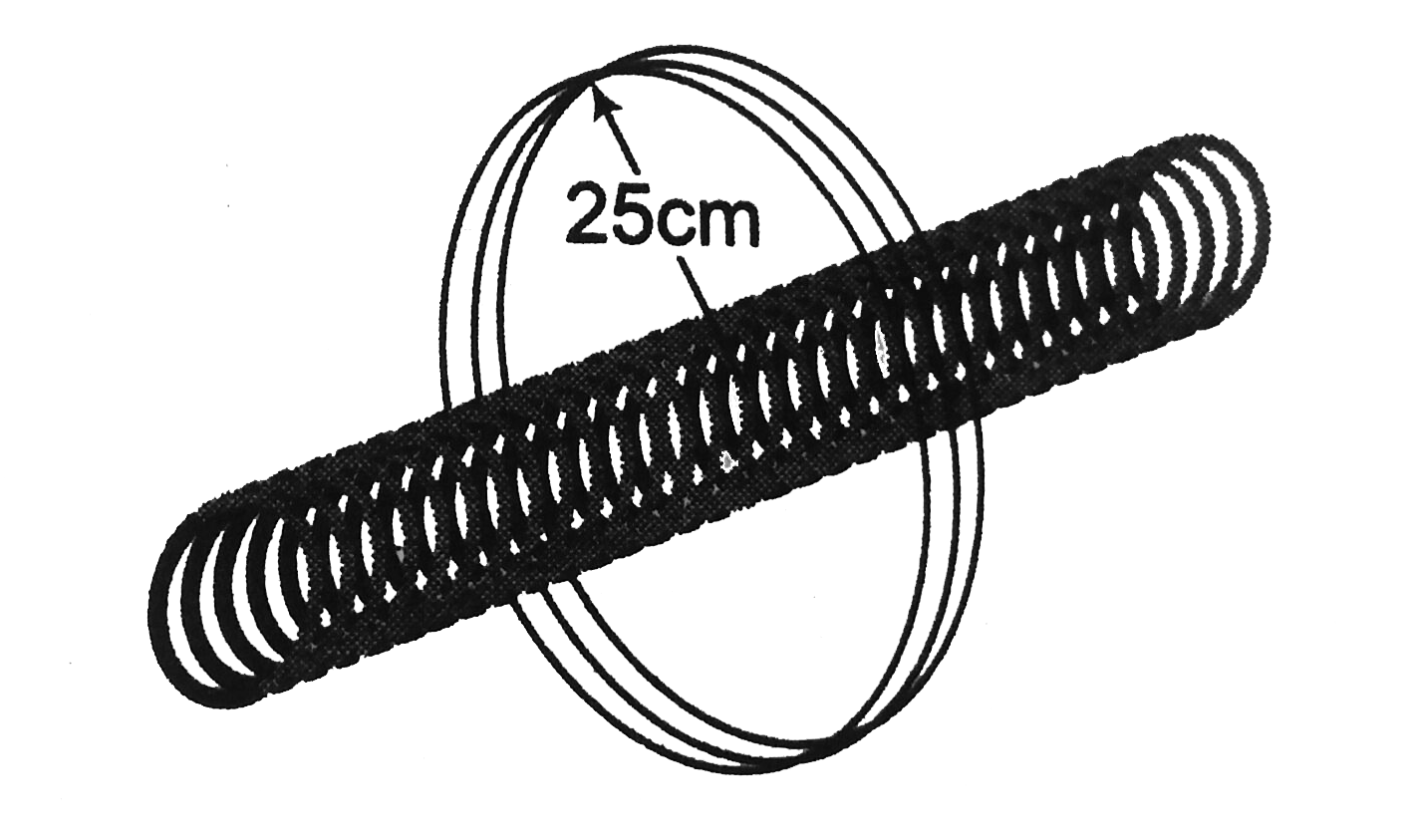
(a) What is the emf induced in the coil when the current through the solenoid is decreasing at a rate −0.20A/s ?
(b) What is the electric field induced in the coil?
View SolutionOver a solenoid of 50 cm length and 2 cm radius having 500 turns. Is wound another wire of 50 turns near the centre. Calculate mutual inductance of the two coils. If currents in primary changes From 0 to 5 a in 0.02 s, what is the emf induced in secondary coil ?
View SolutionA closlly wound coil of 1000 turns and cross sectional area 2×10−4m2 carries a current of 1.0 A.The magnetic moment of the coil is
View Solution
