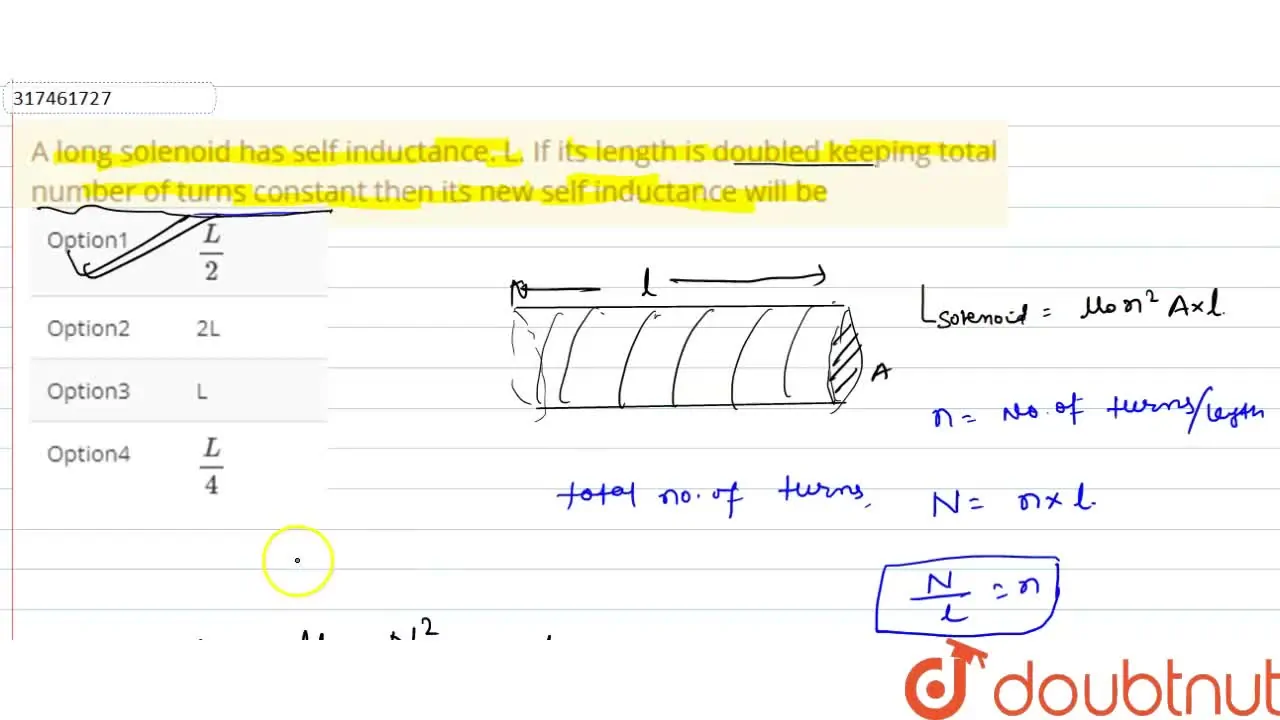
A long solenoid has self inductance. L. If its length is doubled keeping total number of turns constant then its new self inductance will be
L2
2L
L
L4
The correct Answer is:A
Step by step video, text & image solution for A long solenoid has self inductance. L. If its length is doubled keeping total number of turns constant then its new self inductance will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION - B) (OBJECTIVE TYPE QUESTIONS)11videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -C) Previous Years Questions23videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE17videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTECHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION -J(Aakash Challengers Questions)5videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions25videos
ELECTROMAGNETIC WAVES
Similar Questions
A solenoid have self inductance 2H. If length of the solenoid is double having turn density and area constant then new self inductance is :-
View SolutionIf both the number of turns and core length of an inductor is doubled keeping other factors constant, then its self-inductance will be:
View SolutionIf number of turns in a coil is quadrupled, then self-inductance will be:
View Solutionthe dimension of self inductance L are
View Solutionthe dimension of self inductance L are
View SolutionIf both the number of turns and core length of an inductor is doubled keeping other factorsconstant, then its self-inductance will be
View SolutionThe length l of a wire is shaped to form a coil of 1 turn. This coil has self inductance L. if the same length is bend more sharply to form 3 turns, the self inductance will become/remain
View SolutionIf the number of turns in a coil becomes doubled, then its self-inductance will become
View SolutionThe self inductance L of a solenoid depends on the number of turns per unit length 'n' as
View SolutionSelf inductance || L-R Circuit
View Solution