If a small sphere of mass m and charge q is hung from a silk thread at an angle θ with the surface of a vertical charged conducting plate, then for equilibrium of sphere, the surface charge density of the plate is
ε0(mgq)tanθ
ε0(2mgq)tanθ
ε0(mgq)tanθ
ε0(mg3q)tanθ
The correct Answer is:A
To solve the problem of finding the surface charge density (σ) of a vertical charged conducting plate when a small sphere of mass m and charge q is hung from a silk thread at an angle θ, we can follow these steps:
Step 1: Identify the Forces Acting on the Sphere
The forces acting on the sphere include:
- The gravitational force (Fg) acting downwards: Fg=mg
- The tension (T) in the thread acting at an angle θ with the vertical
- The electric force (Fe) due to the electric field (E) created by the charged plate: Fe=qE
Step 2: Resolve the Tension into Components
The tension in the thread can be resolved into two components:
- The vertical component: Tcosθ
- The horizontal component: Tsinθ
Step 3: Apply the Equilibrium Conditions
For the sphere to be in equilibrium, the net force in both the vertical and horizontal directions must be zero.
Vertical Direction:
Tcosθ=mg(1)
Horizontal Direction:
Tsinθ=qE(2)
Step 4: Express the Electric Field in Terms of Surface Charge Density
The electric field (E) due to an infinite charged plate with surface charge density σ is given by:
E=σ2ϵ0(3)
Step 5: Substitute Equation (3) into Equation (2)
Substituting the expression for E from Equation (3) into Equation (2):
Tsinθ=q(σ2ϵ0)(4)
Step 6: Solve for Tension T
From Equation (1), we can express T:
T=mgcosθ(5)
Step 7: Substitute T from Equation (5) into Equation (4)
Substituting Equation (5) into Equation (4):
mgcosθsinθ=q(σ2ϵ0)
Step 8: Rearrange to Solve for Surface Charge Density σ
Rearranging the equation gives:
σ=2mgtanθqϵ0(6)
Final Answer
Thus, the surface charge density (σ) of the plate is:
σ=2mgqϵ0tanθ
The forces acting on the sphere include:
- The gravitational force (Fg) acting downwards: Fg=mg
- The tension (T) in the thread acting at an angle θ with the vertical
- The electric force (Fe) due to the electric field (E) created by the charged plate: Fe=qE
The tension in the thread can be resolved into two components:
- The vertical component: Tcosθ
- The horizontal component: Tsinθ
For the sphere to be in equilibrium, the net force in both the vertical and horizontal directions must be zero.
Tcosθ=mg(1)
Tsinθ=qE(2)
The electric field (E) due to an infinite charged plate with surface charge density σ is given by:
E=σ2ϵ0(3)
Substituting the expression for E from Equation (3) into Equation (2):
Tsinθ=q(σ2ϵ0)(4)
From Equation (1), we can express T:
T=mgcosθ(5)
Substituting Equation (5) into Equation (4):
mgcosθsinθ=q(σ2ϵ0)
Rearranging the equation gives:
σ=2mgtanθqϵ0(6)
Thus, the surface charge density (σ) of the plate is:
σ=2mgqϵ0tanθ
Similar Questions
A small non-conducting ball of mass m=1mg and charge q=2×10−8C hangs from an insulating thread that makes an angle θ=30∘ with vertical uniformly charged non-conducting sheet.Considering the gravitational force on the ball and assuming that the sheet extends for vertically and into out of the page.Calculate surface charge density of sheet.
View SolutionA charged ball B hangs from a silk thread S, which makes an angle θ with a large charged conducting sheet P, as shown in the figure. The surface charge density σ of the sheet is proportional to
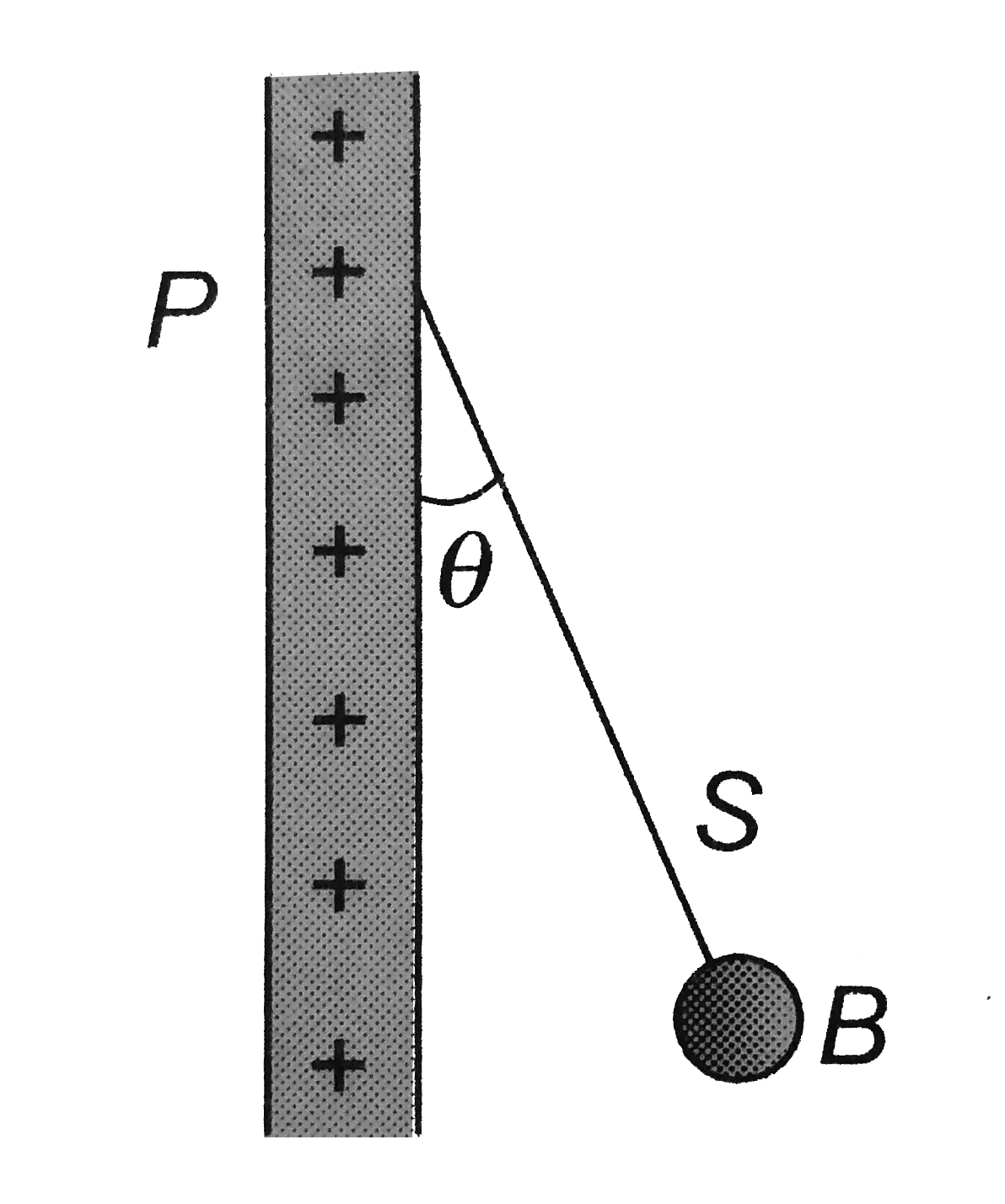
View Solution
Knowledge Check
If a small sphere of mass m and charge q is hanged from a silk thread at an angle θ with the surface of a vectical charged conductinb plate, then for equilibrium of sphere the surface charge density of the plate -
Aε0mgtanθqB(2ε0mgtanθ)/qCε0mgqtanθDε0mgtanθ3qA point object of mass 1mg and charge 1μC is hung with a silk thread at an angle 45 with the surface of a vertical uniformly charged conducting plate. For equilibrium of object , the surface charge density (in SI unit) of the plate is (g= 10m/s2)
A104ε0√3B104ε0C10ε0√3D10ε0A charged ball B hangs from a silk thread S, which makes an angle θ with a large charged conducting sheet P, as shown in figure. The surface charge density σ of the sheet is proportional to
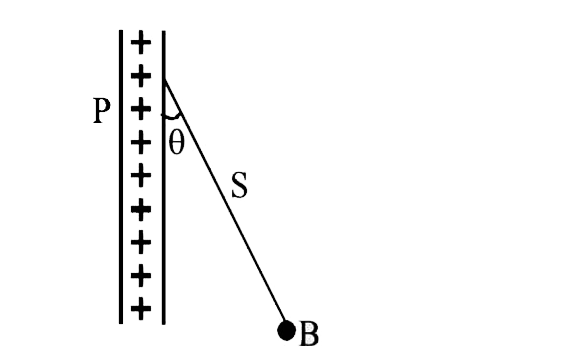 A(a) cotθB(b) cosθC(c) tanθD(d) sinθ
A(a) cotθB(b) cosθC(c) tanθD(d) sinθ
In going from the surface of a charged conducting sphere towards the centre of the sphere the electric field
View SolutionA sphere has surface charge density σ. It is surrounded by a spherical shell. The surface charge density on the spherical shell is
View SolutionA circular metal plate of radius 10 cm is given a charge of 20μC on its surface. The charge density of the plate is
View SolutionA uniformly charged conducting sphere of 4.4 m diameter has a surface change density of 60μCm−2. The charge on the sphere is
View SolutionThe electric field intensity in free space at a distance 'r' outside a charged conducting sphere of radius 'R' in terms of surface charge density σ is
View Solution
