A pipe of varying area of cross-section is held such that its axis is horizontal. At two cross sections A and B, its radii are 8 cm and 4 cm. If velocity of water at A through the pipe is 16 cm s−1 and pressure at A is 10−6 dyne/cm2 , find the pressure at cross-section B.
To find the pressure at cross-section B of the pipe, we will use the principles of fluid dynamics, specifically the continuity equation and Bernoulli's equation. Here’s the step-by-step solution:
Step 1: Identify the given data
- Radius at cross-section A, r1=8 cm
- Radius at cross-section B, r2=4 cm
- Velocity at cross-section A, V1=16 cm/s
- Pressure at cross-section A, P1=10−6 dyne/cm²
Step 2: Calculate the areas at cross-sections A and B
The area A of a circle is given by the formula:
A=πr2
- Area at A:
A1=π(r21)=π(82)=64π cm2
- Area at B:
A2=π(r22)=π(42)=16π cm2
Step 3: Apply the continuity equation
The continuity equation states that the product of area and velocity is constant along the flow:
A1V1=A2V2
Substituting the known values:
64π×16=16π×V2
Cancelling π from both sides:
64×16=16×V2
Dividing both sides by 16:
V2=64 cm/s
Step 4: Apply Bernoulli's equation
Bernoulli's equation for horizontal flow states:
P1+12ρV21=P2+12ρV22
Rearranging to find P2:
P2=P1+12ρV21−12ρV22
Step 5: Substitute the values into Bernoulli's equation
We need to express the density ρ in dyne/cm³. The density of water is approximately 1 g/cm3=1000 kg/m3, which is equivalent to 1000 g/cm3 in CGS units. Thus, ρ=1 g/cm3.
Substituting the values:
- P1=10−6 dyne/cm²
- V1=16 cm/s
- V2=64 cm/s
Now substituting into the equation:
P2=10−6+12⋅1⋅(162)−12⋅1⋅(642)
Calculating the kinetic energy terms:
=10−6+12⋅(256)−12⋅(4096)
=10−6+128−2048
=10−6−1919.999
Step 6: Final calculation
Since 10−6 is negligible compared to 1919.999, we can approximate:
P2≈−1919.999 dyne/cm2
This negative pressure indicates that the assumptions may need to be revisited, as physically, pressure cannot be negative. However, this is a theoretical calculation based on the given velocities and pressures.
Conclusion
The pressure at cross-section B is approximately −1919.999 dyne/cm2, indicating a high velocity leading to a significant drop in pressure.
- Radius at cross-section A, r1=8 cm
- Radius at cross-section B, r2=4 cm
- Velocity at cross-section A, V1=16 cm/s
- Pressure at cross-section A, P1=10−6 dyne/cm²
The area A of a circle is given by the formula:
A=πr2
- Area at A:
A1=π(r21)=π(82)=64π cm2
- Area at B:
A2=π(r22)=π(42)=16π cm2
The continuity equation states that the product of area and velocity is constant along the flow:
A1V1=A2V2
Substituting the known values:
64π×16=16π×V2
Cancelling π from both sides:
64×16=16×V2
Dividing both sides by 16:
V2=64 cm/s
Bernoulli's equation for horizontal flow states:
P1+12ρV21=P2+12ρV22
Rearranging to find P2:
P2=P1+12ρV21−12ρV22
We need to express the density ρ in dyne/cm³. The density of water is approximately 1 g/cm3=1000 kg/m3, which is equivalent to 1000 g/cm3 in CGS units. Thus, ρ=1 g/cm3.
- P1=10−6 dyne/cm²
- V1=16 cm/s
- V2=64 cm/s
P2=10−6+12⋅1⋅(162)−12⋅1⋅(642)
Calculating the kinetic energy terms:
=10−6+12⋅(256)−12⋅(4096)
=10−6+128−2048
=10−6−1919.999
Since 10−6 is negligible compared to 1919.999, we can approximate:
P2≈−1919.999 dyne/cm2
The pressure at cross-section B is approximately −1919.999 dyne/cm2, indicating a high velocity leading to a significant drop in pressure.
Similar Questions
Water flows through a horizontal pipe of varying cross-section at the rate of 20 litres per minuts , determine the velocity of water at a point where diameter is 4cm
View SolutionWater flow through a horizontal pipe. The area of cross-second at one place A1=10cm2, velocity of water flow is 1ms−1 and pressure is 2000 Pa. At another place area A2=5cm2. Whate is the pressure at area A2 ?
View Solution
Knowledge Check
A horizontal pipe of cross-section diameter 5cm carries water at a speed of 4ms−1. The pipe is connected to a smaller pipe with a cross-sectional diameter 4cm. The velocity of water through the smaller pipe is
A6.25ms−1B5.0ms−1C3.2ms−1D2.56ms−1A horizontal pipe of cross sectional diameter 5 cm carries water at a velocity of 4m/s. The pipe is connected to a smaller pipe with a cross-sectional diameter 4 cm. The velocity of water through the smaller pipe is:
A6.25m/sB5.0m/sC3.2m/sD2.56m/sA horizontal pipe of cross sectional diameter 5 cm carries water at a velocity of 4m/s. The pipe is connected to a smaller pipe with a cross-sectional diameter 4 cm. The velocity of water through the smaller pipe is:
A6.25m/sB5.0m/sC3.2m/sD2.56m/s
Water flows through a horizontal pipe of varying area of cross-section at the rate of 10 cubic metre per minute. Determine the velocity of water at a point where radius of pipe is 10cm.
View SolutionWater flows through a horizontal pipe of varying area of cross-section at the rate 5 cubic metre per minute. What is the velocity of water at a point where pipe radius is 10 cm?
View SolutionThe rate of flow of water through a horizontal pipe of changing cross section is 100 litres per hour. Calculate the velocity of water flow at a point where diameter of the tube is 4 cm?
View SolutionA horizontal pipe of varying cross-sectional area carries water flowing at a constant rate. The pressure at sections (1) and (2) are 50cm and 20cm of Hg. If the velocity of section (1) is 5m/s, what is the velocity of section (2). Also determine the ratio of 4 areas at sections (1) and (2) (as shown in Fig.
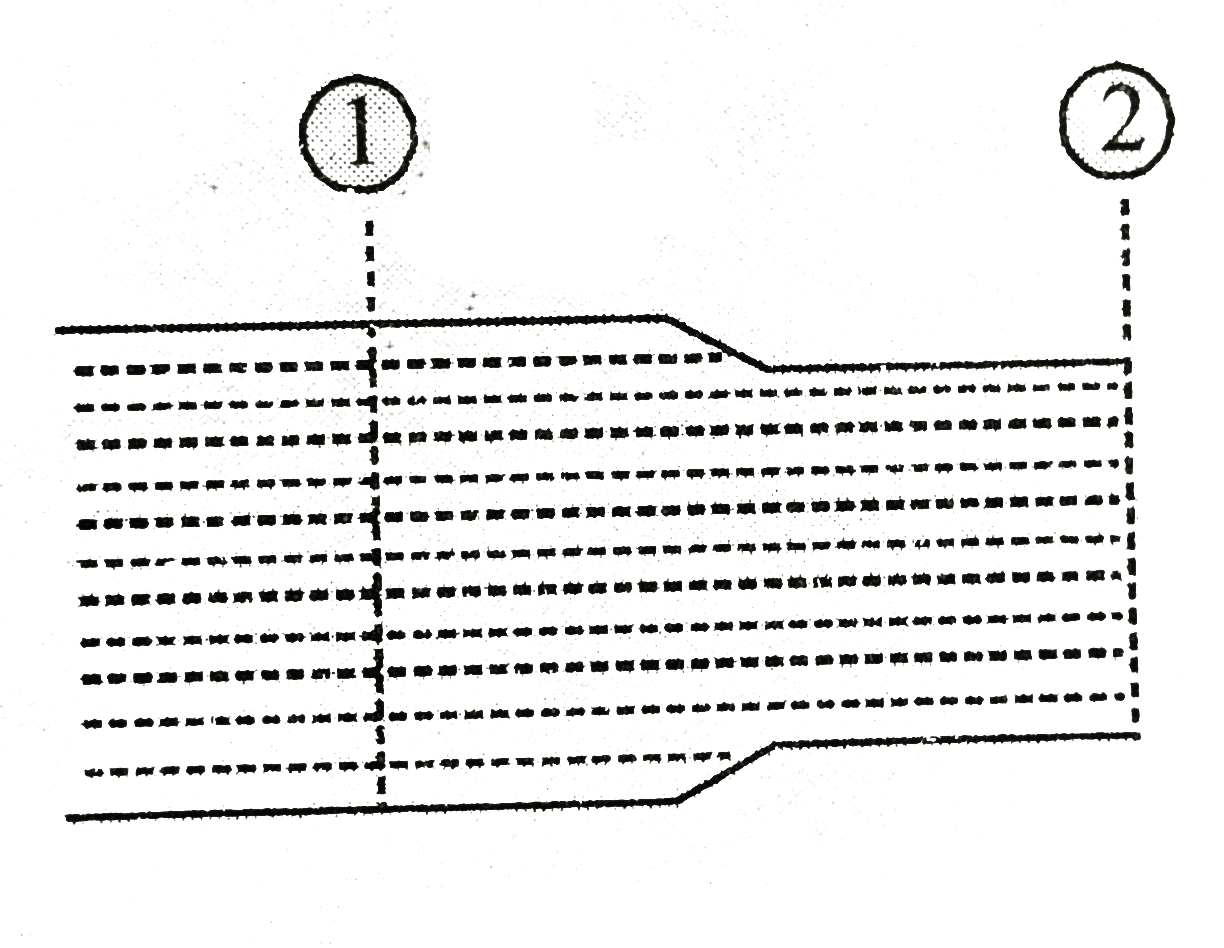
View SolutionA horizontal pipe of area of cross-section a and 3a respectively, then the ratio of velocity of flow at two different cross-section (If flow is streamline) is
View Solution
