A manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the tune (in minutes) required for each toy on the machines is given below: Each machine is available for a maximum of 6 hours per day. If the profit
The correct Answer is:NA
NA
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12.1
10 Videos
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12.2
11 Videos
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exercise 12 A
8 Videos
- INVERES TRIGONOMETRIC FUNCTIONSBOOK - NAGEEN PRAKASHANCHAPTER - INVERES TRIGONOMETRIC FUNCTIONSEXERCISE - Miscellaneous Exercise (prove That )
9 Videos
- MATRICESBOOK - NAGEEN PRAKASHANCHAPTER - MATRICESEXERCISE - Miscellaneous Exerice
15 Videos
Similar Questions
A manufacturer makes two types of toys A and B.Three machines are needed for this purpose and the time (in minutes) required for each toy on the machines is given below:
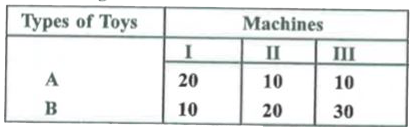
The machine I, II and III are available for a maximum of 3 hours, 2 hours and 2 hours 30 minutes respectively.The profit on each toy of type A is ₹ 50 and that of type B is ₹ 60.Formulate the above problem as a LPP and solve graphically to maximize profit.
View SolutionA manufacture makes two types of cups A and B. Three machines are required to manufacture the cups and the time in minutes required by each in as given below:

Each machine is available for a maximum period of 6 hours per day. If the profit on each cup A is 75 paisa and on B is 50 paisa, find how many cups of each type should be manufactures to maximise the profit per day.
View Solution
Knowledge Check
A company manufactures two types of telephone sets A and B . The A type telephone requires 2 hour and B type telephone requires 2 hour and B type telephone requires 4 hours to make . The company has 800 work hours per day . 300 telephones can pack in a day . The selling prices of A and B type telephones are Rs.300 and 400 respectively . For maximum profits company produces x telephones of a type and y telephones of B types . Then except x≥0andy≥0 , linear constraints and the probable region of the LPP is of the type .
Ax+2y≤400,x+y≤300,
Max z = 300x +400y, boundedB2x+y≤400,x+y≥300,
Max z = 400x +300y, unboundedC2x+y≥400,x+y≥300,
Max z = 300x +400y, parallelogramD2x+y≤400,x+y≥300,
Max z = 300x +400y, squareA manufacturer produces two types of soaps using two machines A and B . A is operated for 2 minutes and B for 3 minutes to manufacture first type , while it takes 3 minutes on machine A and 5 minutes on machine B to manufature second type . Each machine can be operated at the most for 8 hours per day . The two types of soap are sold at a profit of Rs 0.25 and Rs 0.05 each respectively . Assuming that the manufactured can sell all the soaps he can manufacture , how many soaps of each type should be manufature per day so as to maximize his profit .
A50 soaps of type I , 20 soaps of types IIB96 soaps of type IIC45 soaps of type ID55 soaps of type I
A manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the time (in minutes) required for each purpose and the time (in minutes) required for each toy on the machines is given below:
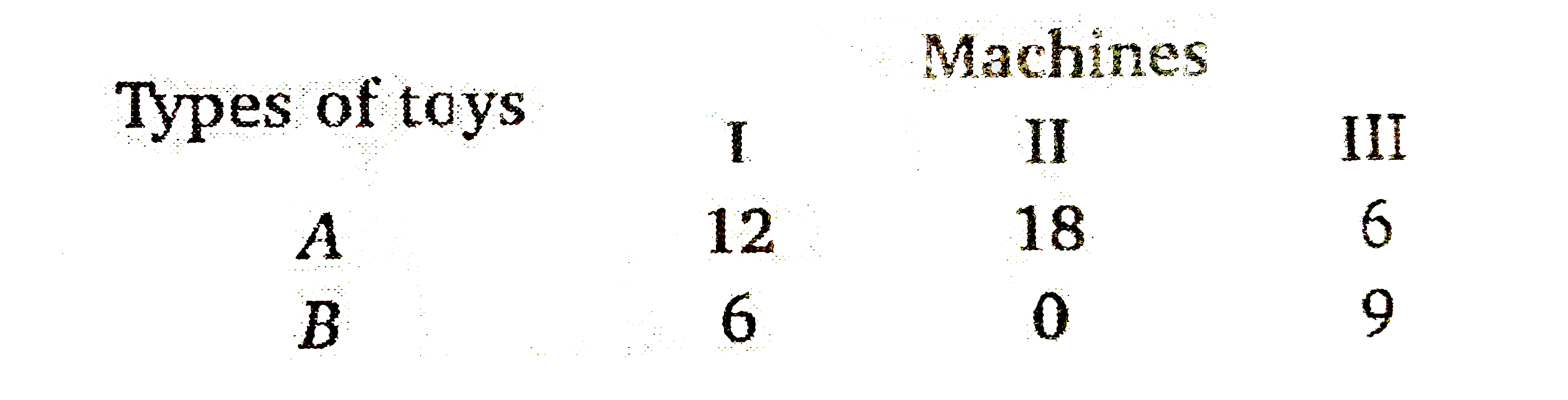
Each machine is available for a maximum of 6 hours per day. If the profit on each toy of type A is Rs. 7.50 and that on each toy of type B is Rs. 5, show that 15 toys of type A and 30 of type B should be manufactured in a day to get maximum profit.
View SolutionA company manufactures two types of toys A and B. Type A requires 5 minutes each for cutting and 10 minutes each for assembling. Type B requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours available for cutting and 4 hours available for assembling in a day. He earns a profit of Rs.50 each on type A and Rs.60 each on type. B. How many toys of each type should the company manufacture in a day to maximize the profit ?
View SolutionA copany manufactures two types of toys, A and B. Type A requires 5 minutes each for cutting and 10 minutes each for assembling. Type B requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours available for cutting and 4 hours available for assembling in a day. The profit is Rs.50 each on type A and Rs.60 each on type B. How many toys of each type should the company manufacture in a day to maximize the profit?
View SolutionA small firm manufactures gold rings and chains. The total number of rings and chains manufactured per day is atmost 24. It takes 1 hour to make a ring and 30 minutes to make a chain. The maximum number of hours available per day is 16. If the profit on a ring is Rs. 300 and that on a chain is Rs. 190, find the number of rings and chains that should be manufactured per day, so as to earn the maximum profit. Make it as an L.P.P. and solve it graphically.
View SolutionA manufacturer produces two products A and B. Both the products are processed on two different machines. The available capacity of first machine is 12 hours and that of second machine is 9 hours per day. Each unit of product A requires 3 hours on both machines and each unit of product B requires 2 hours on first machine and 1 hour on second machine. Each unit of product A is sold at Rs. 7 profit and that of B at a profit of Rs. 4. Find the production level per day for maximum profit graphically.
View SolutionA manufacture produceds two types of steel trunks. He has two machines, A and B. The first type of book case requires 3 hours on machine A and 3 hours on machine B for completion whereas the second type required 3 hours on machine A and 2 hours n machine B. Machines A and B can work at most for 18 hours and 15 hours per day respectively. He earns a profit of Rs.30 and Rs.25 per trunk of the first type and second type respectively. How many trunks of each type must he make each day to make the maximum profit?
View Solution
