Question

$x\ge 3,y\ge 2$

Answer

N/a

Explanation

First inequation : `x ge 3`
Corresponding equation : `x = 3`
This line is parallel to Y-axis, at a distence of 3 units from it and passes through the point A (3, 0) . At point (0, 0) from the inquation, `0ge3` (False)
Its solution is on right side of the line .
Second inequation : `yge2`
Corresponding equation : y = 2
This line is parallel to X-axis, at a distence of 2 units from it and passes through the point B (0, 2).
At point (0 , 0) , from the inequation , 0 ge 2 (False)
Its solution is above the line .

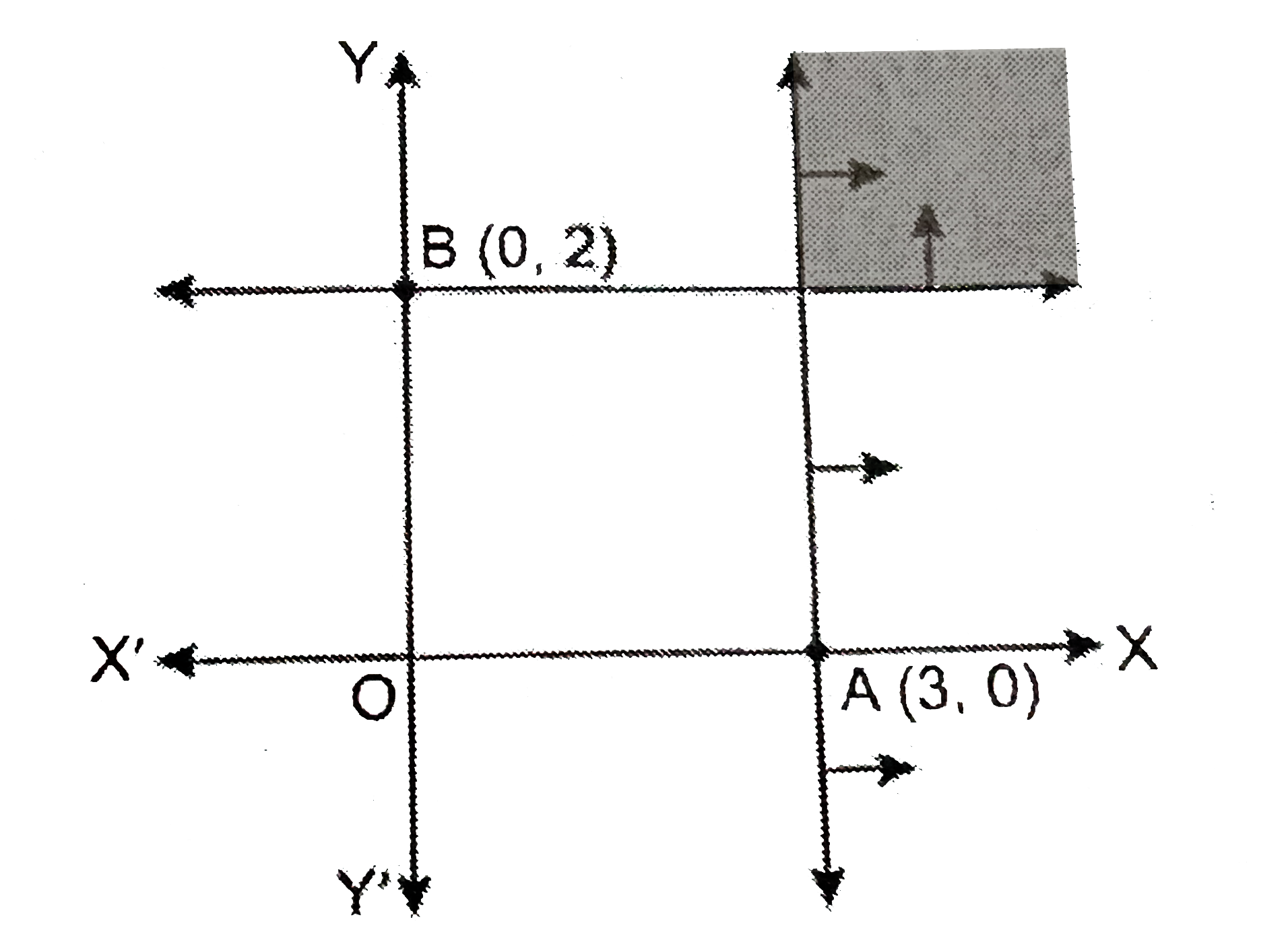
The common solution of two inequations is shown in the shaded region.