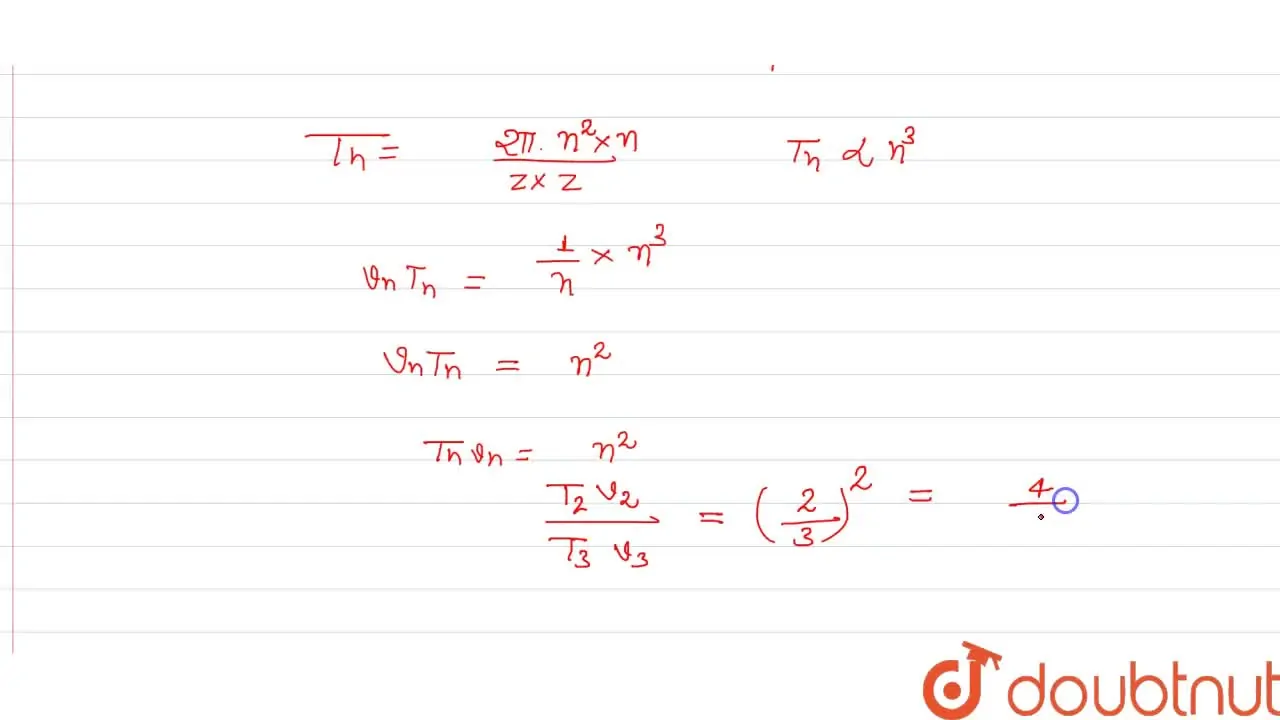
Find the ratio of product of velocity and time period of electron orbiting in 2nd and 3nd stable orbits.
We know that,time period Tn∝n3 and velocity vn∝1/n
⇒ Time period (Tn)×velocity (vn)∝n2
∴ T2v2T3v3=2233=49
Related Playlists
- BOOK - DC PANDEYCHAPTER - ATOMSEXERCISE - Check point 12.114videos
ATOMS
- BOOK - DC PANDEYCHAPTER - ATOMSEXERCISE - Check point 12.216videos
ATOMS
- BOOK - DC PANDEYCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN63videos
ALTERNATING CURRENT
- BOOK - DC PANDEYCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
Similar Questions
What would be the ratio of product of velocity and time period of electron orbiting in 2nd and 3rd stable orbits ?
View SolutionProduct of velocity and time period of electron orbiting in nth stable orbit is proportional to
View SolutionWhat would be the ratio of product of speed and time period of electron orbiting 3rd and 4th stable orbit in Bohr's atomic model?
View SolutionRatio of time period of electron in first and second orbit of H-atom would be -
View SolutionFind the ratio of velocities of electron in 2nd and 4th orbit of hydrogen atom.
View SolutionThe ratio of radius of 2nd and 3rd Bohr orbit is
View SolutionThe ratio of the velocity of electron in 3rd and 5th orbit of hydrogen atom is
View SolutionWhat is the ratio of radius of the 1st orbit to 2nd orbit, if the velocity of the electron in the 1st orbit is twice that of the 2nd orbit.
View SolutionCalculate the number of waves made by a Bohr electron in one complete revolution in nth orbit of He+ ion, if ratio of de-Broglie wavelength associated with electron moving in nth orbit and 2nd orbit is 2.0 :-
View SolutionThe ratio of the velocity of the electron in the first orbit to that in the second orbit is
View Solution