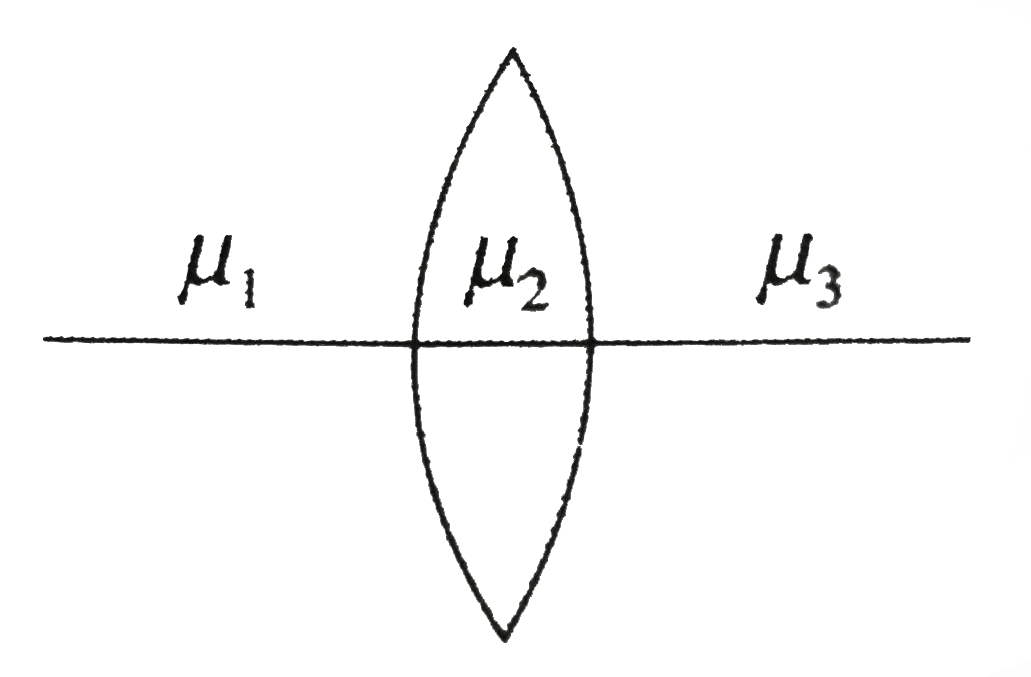The figure shows an equi-convex lens. What should be the condition of the refractive indices so that the lens becomes diverging? 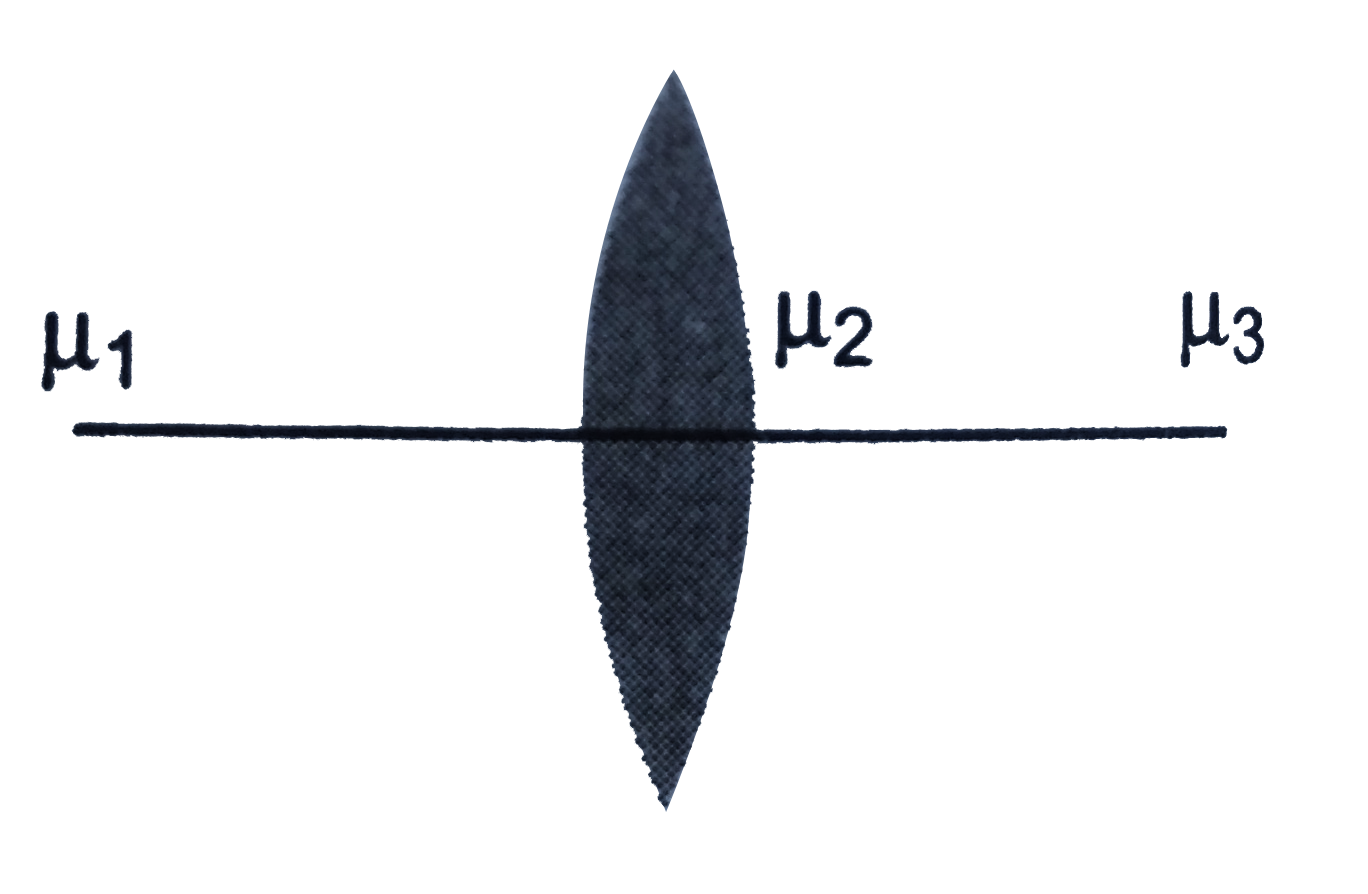
A
B
C
D
None of the above
Video Solution
Text Solution
The correct Answer is:B
(b) We have,
and
From Eqs. (i) and (ii) we get
Lens will become diverging, if f is negative
i.e.,
|
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question
25 Videos
- RAY OPTICSBOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Medical entrance gallary
76 Videos
- RAY OPTICSBOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.7
10 Videos
- NUCLEIBOOK - DC PANDEYCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
Knowledge Check
Figure, shows a concavo-convex lens μ2. What is the condition on the refractive indiecs so the at the lens is diverging?
 A2μ3<μ1+μ2B2μ3>μ1+μ2Cμ3>2(μ1−μ2)DNone of these
A2μ3<μ1+μ2B2μ3>μ1+μ2Cμ3>2(μ1−μ2)DNone of theseThe power in dioptre of an equi-convex lens with radii of curvature of 10cm and refractive index 1.6 is
A+12B+18C+1.2D+1.8The radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
A30cmB50cmC20cmD40cm
A convex lens is a diverging lens.
View SolutionA lens behaves as converging lens in air and a diverging lens in water (n=43) the condition on the value of refractive index n of the material of the lens ?
View SolutionWhat is the other name of a diverging lens ?
View SolutionThe radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
View SolutionA plano-convex lens is made of refractive index of 1.6. The focal length of the lens is
View Solution