A convex mirror is formed from a spherical surface of radius 20 cm. Find the power of the mirror.
To find the power of a convex mirror formed from a spherical surface of radius 20 cm, we can follow these steps:
Step 1: Identify the radius of curvature
The radius of curvature (R) of the convex mirror is given as 20 cm.
Step 2: Calculate the focal length (f)
The focal length (f) of a mirror is related to the radius of curvature (R) by the formula:
f=R2
Since the mirror is convex, the focal length will be positive. Thus, we calculate:
f=20cm2=10cm
Step 3: Convert the focal length to meters
To use the standard SI unit, we convert the focal length from centimeters to meters:
f=10cm=10×10−2m=0.10m
Step 4: Calculate the power (P) of the mirror
The power (P) of a lens or mirror is given by the formula:
P=1f
where f is in meters. Substituting the value of f we calculated:
P=10.10m=10diopters
Step 5: Determine the sign of the power
Since the focal length of a convex mirror is positive, the power will also be positive:
P=+10diopters
Final Answer
The power of the convex mirror is +10diopters.
---
The radius of curvature (R) of the convex mirror is given as 20 cm.
The focal length (f) of a mirror is related to the radius of curvature (R) by the formula:
f=R2
f=20cm2=10cm
To use the standard SI unit, we convert the focal length from centimeters to meters:
f=10cm=10×10−2m=0.10m
The power (P) of a lens or mirror is given by the formula:
P=1f
where f is in meters. Substituting the value of f we calculated:
P=10.10m=10diopters
Since the focal length of a convex mirror is positive, the power will also be positive:
P=+10diopters
The power of the convex mirror is +10diopters.
Topper's Solved these Questions
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Checkpoint 9.1
Explore 10 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Checkpoint 9.2
Explore 10 Videos
- NUCLEIBook:DC PANDEYChapter:NUCLEIExercise:C MADICAL ENTRANCES GALLERY
Explore 46 Videos
- REFLECTION OF LIGHTBook:DC PANDEYChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
Similar Questions
A concave mirror is formed from a sphere of radius 20 cm. The image of an object at an infinite distance is formed at a distance of _____ cm from the mirror.
View SolutionA concave mirror is formed from a sphere of radius 20 cm. The image of an object at an infinite distance is formed at a distance of _____ cm from the mirror.
View Solution
Knowledge Check
An object is placed at 20 cm from a convex mirror of focal length 20 cm. The distance of the image from the pole of the mirror is
AinfinityB10 cmC15 cmD40 cmAssertion : Radius of curvature of a convex mirror is 20 cm. If a real object is placed at 10 cm from pole of the mirror, image is formed at infinity.
Reason : When object is placed at focus, its image is formed at infinityAIf both assertion and reason are true and\ reason is a correct explanation of the assertionBIf both assertion and reason are true but the reason is not a correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is trueAn object of height 1cm is kept perpendicular to the principle axis of a convex mirror of radius of curvature 20cm. IF the distance of the object form the mirror is 20cm then the distance (in cm) between heads of the image and the object will be:
A√64049B√64149C403Dnone of these
A convex mirror is made by cutting a hollow sphare of radius of curvature 20 cm. Find the focal length of the mirror.
View SolutionThere is a convex mirror of radius 50 cm. The image of a point at a distance 50 cm from the pole of mirror on its axis will be formed at :
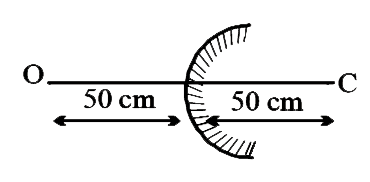
View SolutionAn object of height 5 cm is placed 20 cm away from a convex mirror. Its image is formed at a distance of 12 cm behind the mirror. Calculate the focal length of the mirror using mirror formula. Also draw a scale diagram to locate the image. From the diagram, find out the length of the image formed.
View SolutionA convex lens of focal length 15cm is placed in front of a convex mirror. Both are coaxial and the lens is 5cm from the apex of the mirror. When an object is placed on the axis at a distance of 20cm from the lens, its is found that image coincides with the object. Calculate the radius of curvature of mirror.
[55cm]
View SolutionA 0.5 cm high object is placed at 30 cm from a convex mirror whose focal length is 20 cm. Find the position and size of the image.
View Solution