The frequency for which a 5μF capacitor has a reactance of 11000Ωis given by
100π MHz
1000π MHz
11000Hz
1000 Hz
The correct Answer is:A
To find the frequency for which a 5 µF capacitor has a reactance of 11000Ω, we can use the formula for capacitive reactance:
XC=1ωC
where:
- XC is the capacitive reactance,
- ω=2πf (angular frequency),
- C is the capacitance.
Step 1: Write the equation for capacitive reactance
We start with the formula for capacitive reactance:
XC=1ωC
Step 2: Substitute the values
We know that XC=11000Ω and C=5μF=5×10−6F. Substituting these values into the equation gives:
11000=1ω⋅5×10−6
Step 3: Rearranging the equation
Rearranging the equation to solve for ω:
ω⋅5×10−6=1000
ω=10005×10−6
Step 4: Calculate ω
Calculating ω:
ω=10005×10−6=200000000rad/s
Step 5: Convert ω to frequency f
Now, we convert ω to frequency f using the relation ω=2πf:
f=ω2π=2000000002π
Step 6: Calculate the frequency f
Calculating f:
f=2000000002×3.14≈2000000006.28≈31830988.18Hz
Step 7: Convert to MHz
To convert Hz to MHz:
f≈31.83MHz
Final Answer
Thus, the frequency for which a 5 µF capacitor has a reactance of 11000Ω is approximately:
f≈31.83MHz
- XC is the capacitive reactance,
- ω=2πf (angular frequency),
- C is the capacitance.
We start with the formula for capacitive reactance:
We know that XC=11000Ω and C=5μF=5×10−6F. Substituting these values into the equation gives:
Rearranging the equation to solve for ω:
Calculating ω:
Now, we convert ω to frequency f using the relation ω=2πf:
Calculating f:
To convert Hz to MHz:
Thus, the frequency for which a 5 µF capacitor has a reactance of 11000Ω is approximately:
Similar Questions
Knowledge Check
The frequency for which a 5.0 muF capacitor has a reactance of 1000Ω is given by
A(1000)/(pi) cycles//secB(100)/(pi) cycles//secC200 cycle //secD5000 cycles //secThe frequency for which a 5μF capacitor has a reactance of 1100 ohm is given by
A100πMHzB1000πHzC11000HzD1000HzThe frequency at which 9.0mh inductor and 10μF capacitor will have same reactance is
A0.53kHzB50 kHzC0.33kHzD5.3kHz
A 2μF capacitor is connected across an AC power supply . Calculate the frequency of the supply if the capacitive reactance of the capacitor is 10 Ω .
View Solution(a) At what frequency would a 12 mH inductor and a 10 μF capacitor have the same reactance? (b) What would the reactance be? (c) Show that this frequency would be the natural frequency of an oscillating circuit with the same L and C.
View Solution(a) What is the reactance of a 2.00H inductor at a frequency of 50.0Hz?
(b) What is the inductance of an inductor whose reactance is 2.00Ω at 50.0Hz?
(c) What is the reactance of a 2.00μF capacitor at a frequency of 50.0Hz?
(d) What is the capacitance of a capacitor whose reactance is 2.00Ω at 50.0Hz?
View SolutionThe reactance of a 25μF capacitor at the AC frequency of 4000Hz is
View SolutionA generator with an adjustable frequency of oscillaton is connected to resistance, R=1000Ω, inductance, L1=1.7mH and L2=2.3mH and capacitance, C1=4μF,C2=2.5μF and C3=3.5μF. The resonant angular frequency of the circuit is
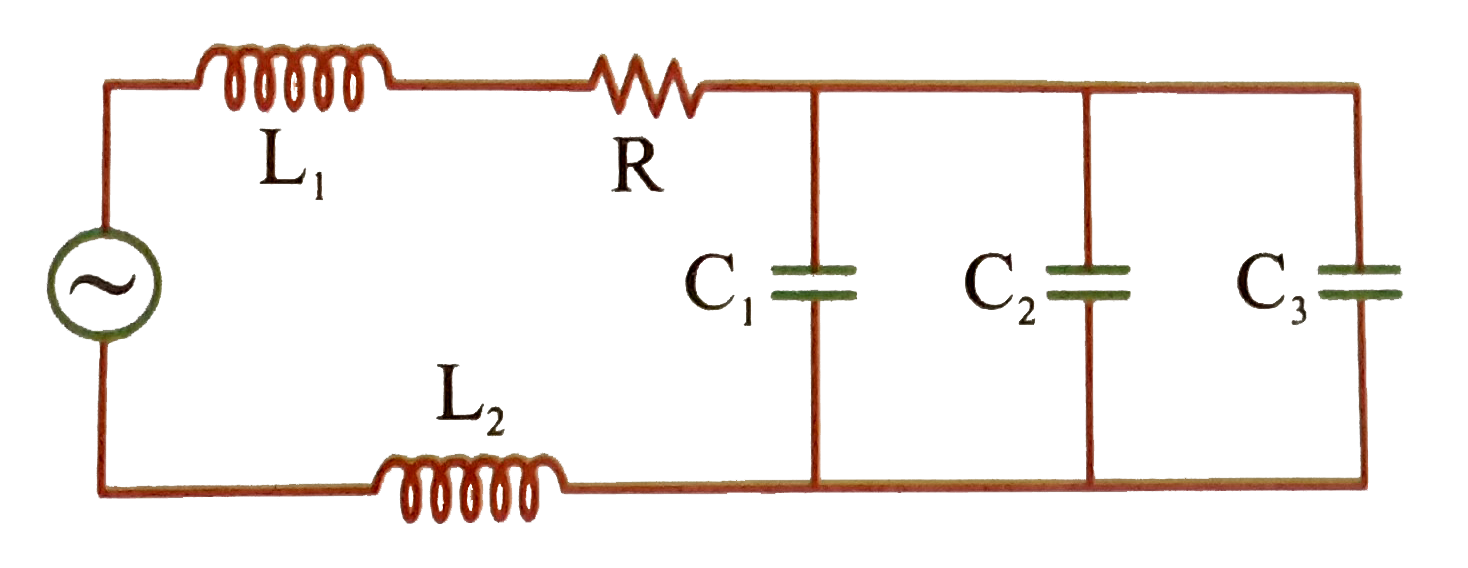
View Solution