Question

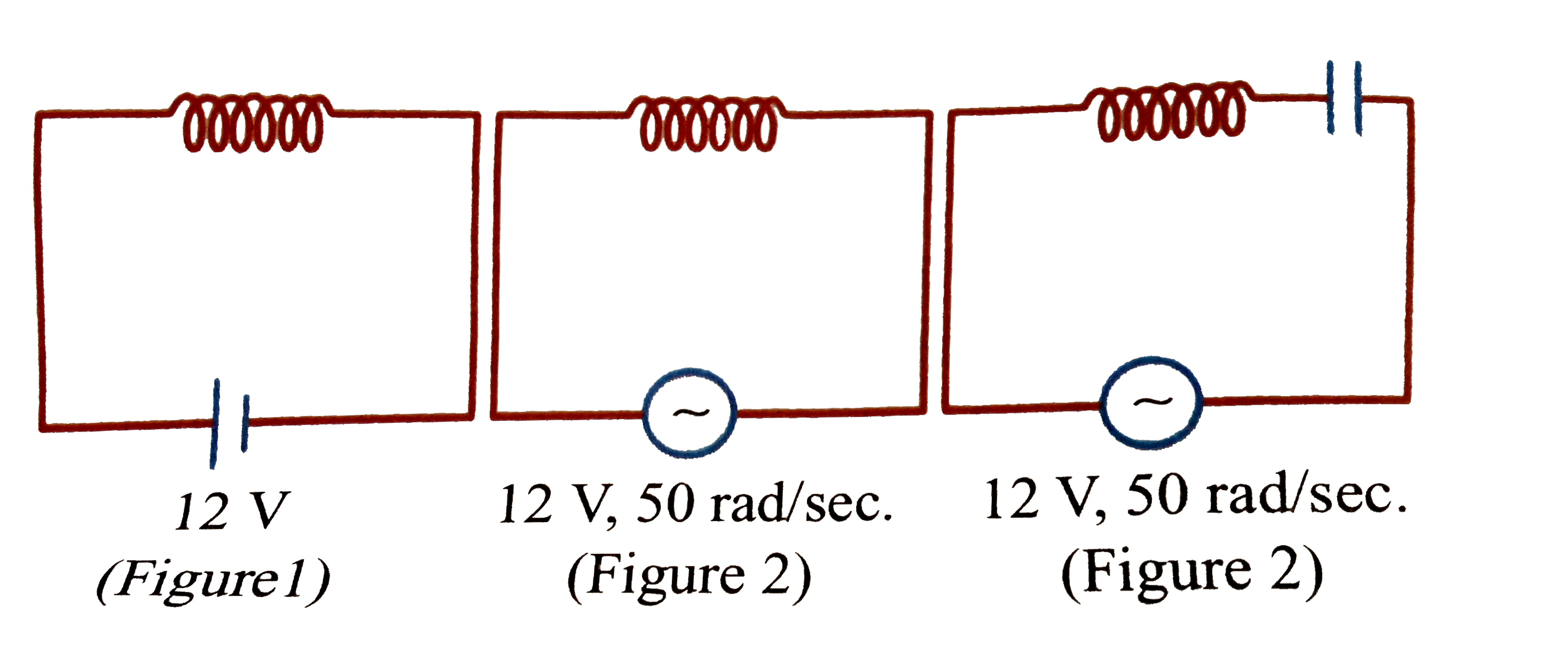
A long solenoid connected to a 12V DC source passes a steady current of 2 A. When the solenoid is connected to an AC source of 12V at 50 Hz, the current flowing is 1A. Calculate inductance of the solenoid.

Explanation

To calculate the inductance of the solenoid, we will follow these steps:

Step 1: Calculate the Resistance of the Solenoid
When the solenoid is connected to a DC source, the voltage (V) is 12V and the current (I) is 2A. We can use Ohm's law to find the resistance (R) of the solenoid.

\( R = \frac{V}{I} = \frac{12V}{2A} = 6 \, \Omega \)

Step 2: Set Up the AC Circuit Equation
When the solenoid is connected to an AC source, the voltage is still 12V, but the current (I) is now 1A. The total impedance (Z) in the AC circuit can be expressed as:

\( Z = \frac{V}{I} = \frac{12V}{1A} = 12 \, \Omega \)

Step 3: Relate Impedance to Resistance and Inductive Reactance
The impedance (Z) in an AC circuit with resistance (R) and inductive reactance (X_L) is given by:

\( Z = \sqrt{R^2 + X_L^2} \)

Where \(X_L = \omega L\) and \(\omega = 2\pi f\). Given that the frequency (f) is 50 Hz:

\( \omega = 2\pi \times 50 = 100\pi \, \text{rad/s} \)

Substituting \(R\) and \(Z\) into the impedance equation:

\( 12 = \sqrt{6^2 + (100\pi L)^2} \)

Step 4: Square Both Sides
Squaring both sides to eliminate the square root gives:

\( 12^2 = 6^2 + (100\pi L)^2 \)

This simplifies to:

\( 144 = 36 + (100\pi L)^2 \)

Step 5: Solve for Inductive Reactance
Rearranging the equation to isolate the inductive reactance term:

\( (100\pi L)^2 = 144 - 36 \)
\( (100\pi L)^2 = 108 \)

Step 6: Solve for L
Taking the square root of both sides:

\( 100\pi L = \sqrt{108} \)
\( L = \frac{\sqrt{108}}{100\pi} \)

Calculating \(\sqrt{108}\):

\( \sqrt{108} = 6\sqrt{3} \)

Thus:

\( L = \frac{6\sqrt{3}}{100\pi} \)

Step 7: Calculate the Final Value of L
Now, calculating the numerical value:

\( L \approx \frac{6 \times 1.732}{100 \times 3.14} \approx \frac{10.392}{314} \approx 0.0331 \, \text{H} \approx 33.1 \, \text{mH} \)

Final Answer
The inductance of the solenoid is approximately 33.1 mH.

---