The length of a magnetised steel wire is l is the magnetic momemt is M. It is bent the shape of L with two sides equal. What will be the new magnetic moment?
To solve the problem, we need to determine the new magnetic moment of a magnetized steel wire after it has been bent into the shape of an "L".
1. Understanding the Initial Conditions:
- The length of the magnetized steel wire is L.
- The magnetic moment of the wire before bending is M.
2. Bending the Wire:
- When the wire is bent into the shape of an "L", it forms two equal sides. Each side of the "L" will have a length of L2.
3. Magnetic Moment Calculation:
- The magnetic moment M is defined as the product of the magnetic pole strength m and the distance d between the poles.
- Initially, the magnetic moment can be expressed as:
M=m⋅L
4. New Configuration:
- After bending, we have two segments of the wire, each of length L2.
- The magnetic moments of these two segments can be considered as being positioned at the ends of the "L".
5. Distance Between the Magnetic Poles:
- The distance between the two ends of the "L" shape can be calculated using the Pythagorean theorem:
d=√(L2)2+(L2)2=√L24+L24=√L22=L√2
6. New Magnetic Moment:
- The new magnetic moment M′ after bending can be calculated as:
M′=m⋅d=m⋅L√2
- Since m=ML (from the initial magnetic moment), we can substitute this into the equation:
M′=(ML)⋅L√2=M√2
Final Answer:
The new magnetic moment M′ after bending the wire into the shape of an "L" is:
M′=M√2
- The length of the magnetized steel wire is L.
- The magnetic moment of the wire before bending is M.
- When the wire is bent into the shape of an "L", it forms two equal sides. Each side of the "L" will have a length of L2.
- The magnetic moment M is defined as the product of the magnetic pole strength m and the distance d between the poles.
- Initially, the magnetic moment can be expressed as:
M=m⋅L
- After bending, we have two segments of the wire, each of length L2.
- The magnetic moments of these two segments can be considered as being positioned at the ends of the "L".
- The distance between the two ends of the "L" shape can be calculated using the Pythagorean theorem:
d=√(L2)2+(L2)2=√L24+L24=√L22=L√2
- The new magnetic moment M′ after bending can be calculated as:
M′=m⋅d=m⋅L√2
- Since m=ML (from the initial magnetic moment), we can substitute this into the equation:
M′=(ML)⋅L√2=M√2
The new magnetic moment M′ after bending the wire into the shape of an "L" is:
M′=M√2
Topper's Solved these Questions
- MAGNETISM AND MATTERBook:DC PANDEYChapter:MAGNETISM AND MATTERExercise:5.1
Explore 14 Videos
- MAGNETISM AND MATTERBook:DC PANDEYChapter:MAGNETISM AND MATTERExercise:5.2
Explore 14 Videos
- MAGNETICSBook:DC PANDEYChapter:MAGNETICSExercise:MCQ_TYPE
Explore 1 Video
- MODERN PHYSICSBook:DC PANDEYChapter:MODERN PHYSICSExercise:Integer Type Questions
Explore 17 Videos
Similar Questions
Knowledge Check
A steel wire of length 'l' has a magnetic moment 'M'. It is bent in 'L' shape having equal size of arm. The new magnetic moment is
AM2BM√2CMD2MA steel wire of length l has a magnetic moment M. It is bent into L shape from the middle. The new magnetic moment is :
AMBM√2CM2D2MA bar magnet of magnetic moment M is bent in
 shape such that all the parts are of equal lengths. Then new magnetic moment is AM/3B2MC√3MD3√3M
shape such that all the parts are of equal lengths. Then new magnetic moment is AM/3B2MC√3MD3√3M
A steel wire of length l has a magnetic moment M. It is bent in shape at its midpoint. The new magnetic moment is
View SolutionA bar magnet of moment 'M' is bent into a shape
 . If the length of the each part is same, its new magnetic moment will be
. If the length of the each part is same, its new magnetic moment will be
View SolutionA bar magnet of magnetic moment M is bent in
 shape with equal arm lengths.The new magnet moment is
shape with equal arm lengths.The new magnet moment is
View SolutionA bar magnet of magnetic moment M is bent in the form of quadrant of circular arc . The new magnetic moment is
View SolutionA steel wire of length l has a magnetic moment M. It is then bent into a semicircular arc. The new magnetic moment is
View Solution

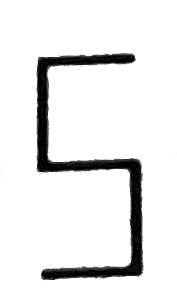 with all segments of equal length the new magnetic moment is
with all segments of equal length the new magnetic moment is