A solid ball of density half that of water falls freely under gravity from a height of 19.6 m and then enters water. Up to what depth will the ball go. How much time will it take to come again to the water surface? Neglect air resistance and viscosity effects in water. (Take g=9.8m/S2).
4 s
8s
6s
2s
The correct Answer is:A
v=√2gh=√2×9.8×19.6=19.6ms−1
Let ρ be the density of ball and 2ρ the density of water. Net retardation inside the water,
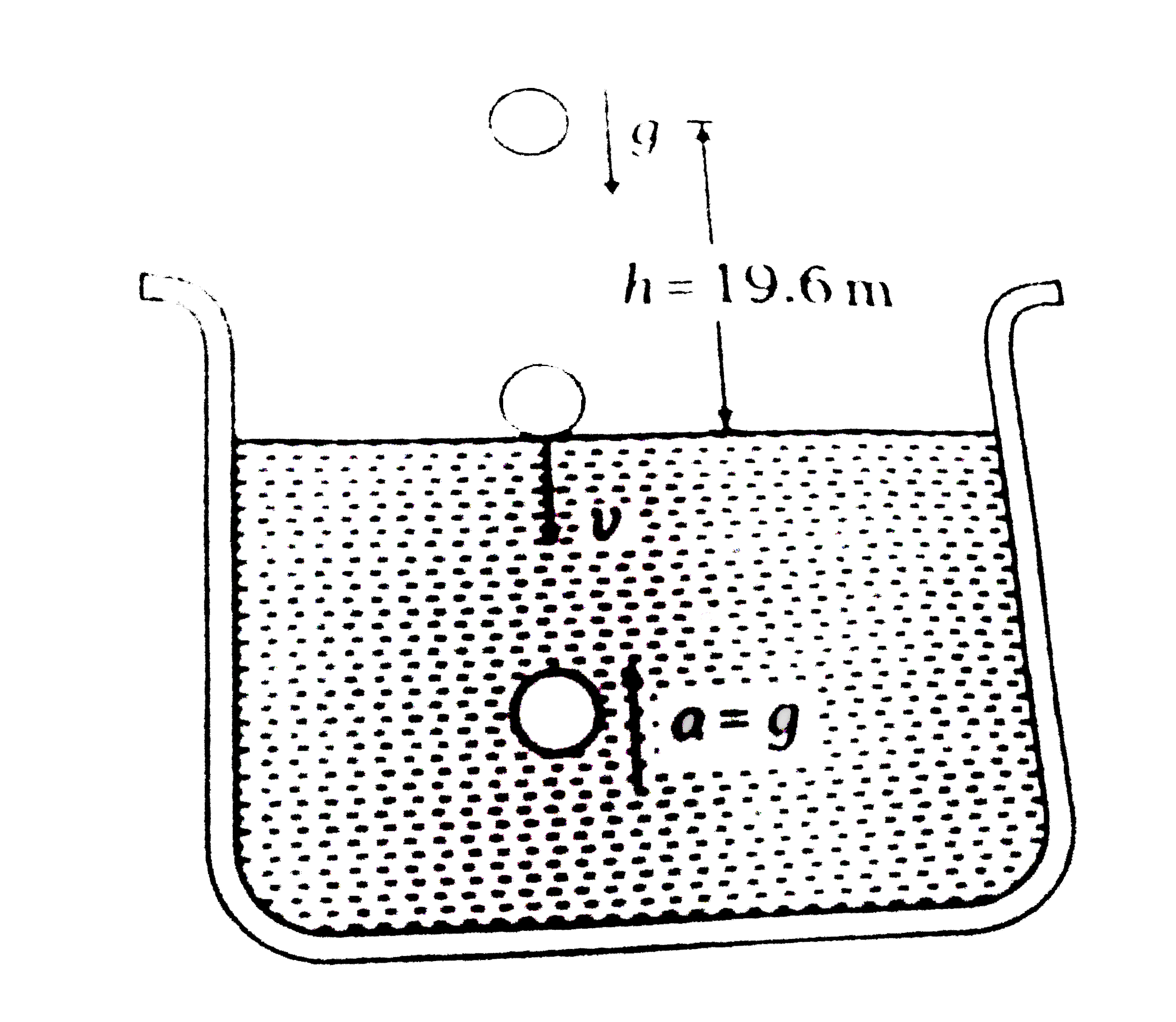
a=upthrust-weightmass
=V(2ρ)g−V(ρ)(g)V(ρ)g (V=volume of ball)
=g=9.8ms−2
Hence, the ball will go upto the same depth 19.6 m below the water surface. Further, time taken by the abll to come back to water surface is, t=2(va)=2(19.69.8)=4s
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - B) Medical entrance special format question
19 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Match the columns
6 Videos
- FLUID MECHANICSBOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Check point 13.4
10 Videos
- EXPERIMENTSBOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A soild ball of density half that of water falls freely under gravity from a height of 19.6 m and then enters water. Upto what depth will the ball go. How much time will it take to come again to the water surface? Neglect air resistandce and viscosity effects in water. (Take g=9.8m/S2).
View SolutionA solid ball of density half that of water falls freely under gravity from a height of 20m and then enters water. Up to what depth will the ball go ? How much time will it take to come again to the wate surface ? Neglect air resistance and viscosity effect in water.
View Solution
Knowledge Check
A solid ball of density half that of water falls freely under gravity from a height of 19.6 m and then enters water. Neglecting air resistance and viscosity effect in water, the depth up to which the ball will go is (g=9.8m/s2)
A19.6 mB9.8 mC14.7 mD12.7 mA ball whose density is 0.4×103kg/m3 falls into water from a height of 9 cm. To what depth does the ball sink ?
A9 cmB6 cmC4.5 cmD2.25 cm
A solid ball of density half that of water falls freely under gravity from a height of 19.6 m and then enters water. Upto what depth will the ball go? How much time will take to come again to the water surface? Neglect air resistance and viscosity effects in water (g=9.8m/s2)
View SolutionConsider a ball of density 0.5×103kg/m3 falling freely into the water from a height of 10 cm. Now, find the depth (in centimetres) to which the ball will sink:
View SolutionA wooden ball of density D is immersed in water of density d to a depth h//2 below the surface of water and then relased. To what height will the ball jump out of water ?
View SolutionA metal ball of mass 5 kg falls from a height of 490 m. How much time will it take to reach the ground ?
View SolutionA ball of relative density 0.8 falls into water from a height of 2 m. find the depth to which the ball will sink (neglect viscous forces)
View SolutionA ball of radius r and density r falls freely under gravity through a distance h before entering water. Velocity of ball does not change even on entering water. If viscosity of water is η the value of h is given by
View Solution
