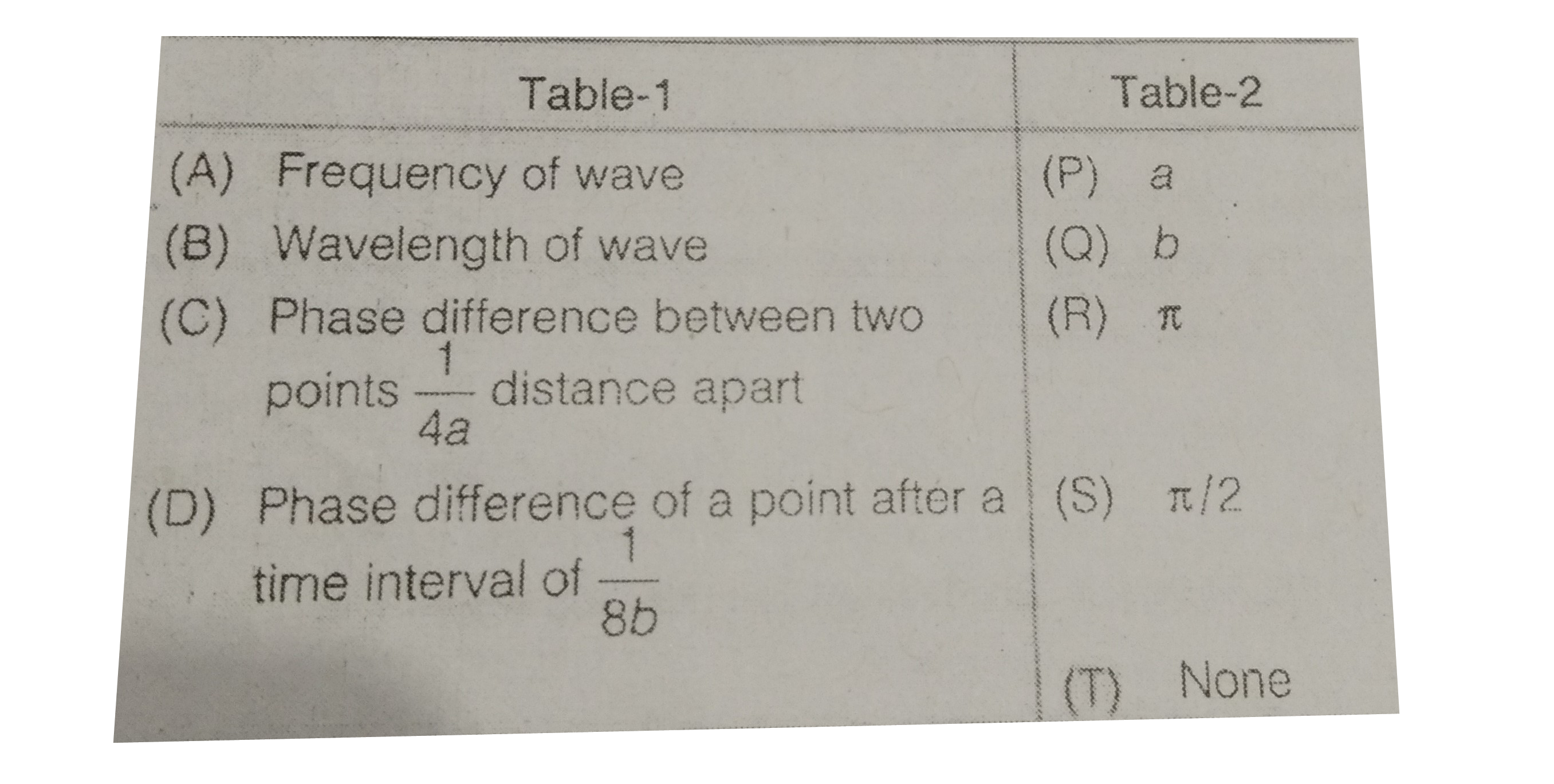
In the equation $y = A sin (ω t + \frac{π}{4})$ match the following for x= $\frac{A}{2}$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(A \to q, B \to s, C \to p)$

Updated on:21/07/2023

In the wave equation,
$y = A \frac{sin (2 π)}{a} (x - b t)$
Aspeed of wave is $a$
Bspeed of wave is $b$
Cwavelength of wave is $a / b$
Dwavelength of wave is $a$
A particle excuting SHM has equation $y = A sin (ω t + \frac{π}{6})$ where $ω = \frac{2 π}{T}$ , then find the possible time t when it is at $y = - \frac{A}{2}$
A $\frac{T}{2}$
B $\frac{5 T}{6}$
C $\frac{3 T}{2}$
D $\frac{11 T}{6}$
The equation y=Asin^2(kx-omegat) represents a wave motion with
Aamplitude A, frequency $ω / 2 π$
Bamplitude $A / 2$ , frequency of $\frac{ω}{π}$
Camplitude 2A, frequency $ω / 4 π$
Dit does not represent a wave motion