A particle of mass m is dropped from a great height h above the hole in the earth dug along its diameter.
The motion of the particle is simple harmonic
The motion of the particle is periodic
The speed of the particle at the centre of earth equals √2GM(R+h) , where R and M are the radius and mass of the earth respectively
The speed of the particle at the centre of earth equals √GM(R+3h)R(R+h), where R and M are the radius and mass of the earth respectively
The correct Answer is:D
(d) The motion is simple harmonic only inside earth. Further
12mv2=−GMm(R+h)+32GMmR
⇒v=√GM(R−h)R(R+h)
Similar Questions
A particle of mass 2m is dropped from a height 80 m above the ground. At the same time another particle of mass m is thrown vertically upwards from the ground with a speed of 40 m/s. If they collide head-on completely inelastically, the time taken for the combined mass to reach the ground, in units of second is:
View SolutionA particle of mass 2 is dropped from a height 80 m above the ground. At the same time another particle of mass m is thrown vertically upwards from the ground with a speed of 40 m/s. If they collide head-on completely inelastically, the time taken for the combined mass to reach the ground, in units of second is:
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m is dropped from a height h above the ground. At the same time another particle of mass 2m is thrown vertically upwards from the ground with a speed of √gh . If they collide head-on completely inelastically, the time taken for the combined mass to reach the ground, in units of √hg is:
A√40−26B√30−37C√20−25D√32 - Question 2 - Select One
A particle of mass m is dropped from a height h above the ground. At the same time another particle of mass 2m is thrown vertically upwards from the ground with a speed of √gh . If they collide head-on completely inelastically, the time taken for the combined mass to reach the ground, in units of √hg is :
A√40−26B√30−37C√20−25D√32 - Question 3 - Select One
A particle of mass m is dropped from a height h above the ground. Simultaneously another particle of the same mass is thrown vertically upwards from the ground with a speed of√2gh. If they collide head-on completely inelastically, then the time taken for the combined mass to reach the ground is
A√3h4gB√3h2gC√h2gD√h4g
A small particle is dropped from a height R in front of a narow tunnel dug inside the earth (along a diameter). Let M be the mass of earth, R be radius of earrth. Let v0,T be speed of particle when it reaches A and time taken by particle to go from A to B respectively. Assuming mass of particle to be negligible as compare to mass of earth, pick the correct option(s)
View Solution_S01_036_Q01.png)
A solid sphere of mass M and radius R is surrounded by a spherical shell of same mass and radius 2R as shown. A small particle of mass m is relased from rest from a height h(<<R) above the shell. There is a hole in the shell.
Q. In what time will it enter the hole at AView SolutionA solid sphere of mass M and radius R is surrounded by a spherical shell of same mass M and radius 2R as shown. A small particle of mass m is released from rest from a height h(<<R) above the shell. There is a hole in the shell.
What time will it take to move from A to B?View SolutionA solid sphere of mass M and radius R is surrounded by a spherical shell of same mass M and radius 2R as shown. A small particle of mass m is released from rest from a height h(<<R) above the shell. There is a hole in the shell.
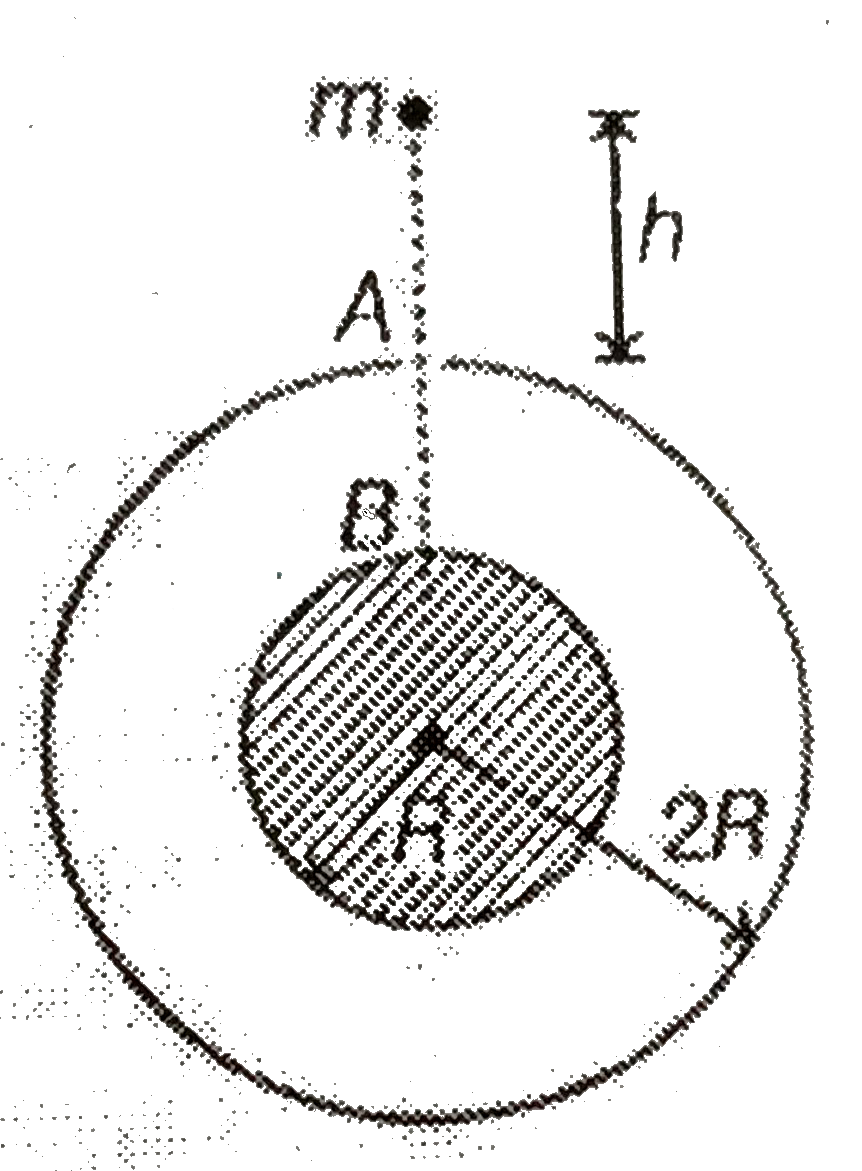
What speed will it collide at B?View SolutionA ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
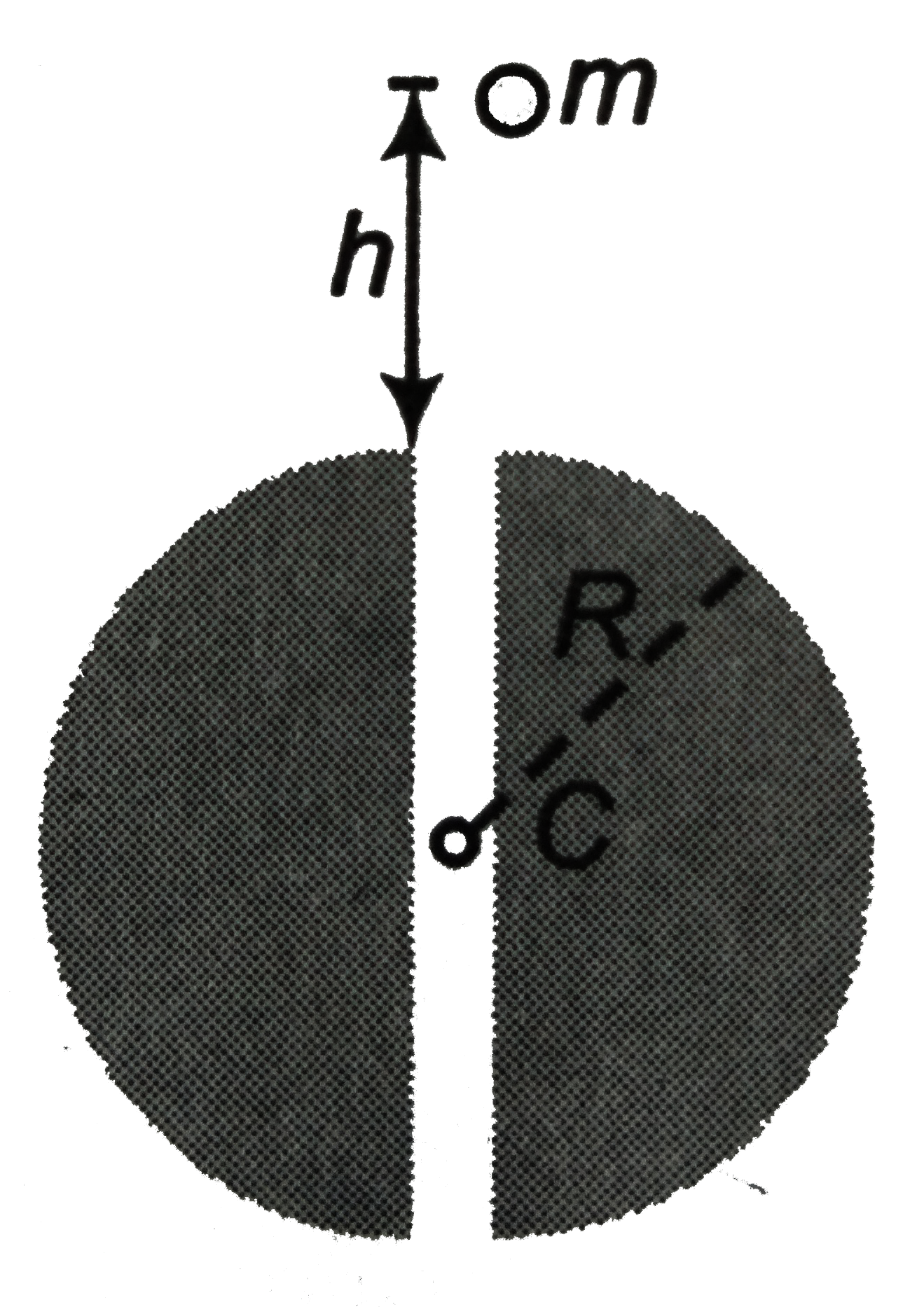View Solution