Determine the period of small oscillations of a mathematical pendulum, that is a ball suspended by a thread l=20cm in length, if it is located in a liquid whose density is η=3.0 times less than that of the ball. The resistance of the liquid is to be neglected.
Let, V=volume of bob
ρ =density of material of the bob
g'=effective value of acceleration due to gravity
Now , effective weight=weight of bob- upthrust of liquid
Vρg'=Vρg−Vρηg
g'=(1−1η)gorg'=η−1ηg
Again, T=2π√lg' or T=2π
⎷lη−1ηg
or T=2π√ηl(η−1)g
Substituting values, we get
T=2×227√3×20×10−2(3−1)9.8s=447×0.175s=1.1s
Similar Questions
A simple pendulum has time period 2s in air. If the whole arrangement is placed in a non viscous liquid whose density is 1/2 times the density of bob, find the time period of oscillation in liquid.
View SolutionA solid cube of sides 2 cm each is suspended by a thread and completely immersed in a liquid of density 0.85×103 kg/m3. What will be the tension in the thread if relative density of solid is 5?
View Solution
Knowledge Check
The time period of a simple pendulum gets increased, it it is made to oscillates in a liquid whose density is
Aless than the density of the material of the bobBgreater than the density of the material of the bobCequal to the density of the material of the bobDless than or equal to density of the material at the bobA small ball is dropped from a certain height on the surface of a non-viscous liquid of density less than the density of ball. The motion of the ball is
ASHMBperiodic but not SHMCnot periodicDSHM for half a period and non-periodic for rest half of the periodThe periodic time of a simple pendulum, oscillating in air is T. But if the bob of the simple pendulum is completely immersed in a nonviscous liquid, whose density is 120 th of the material of the bob, then its new period will be
ATB1920TC2019TD√2019T
Derive an expression for the angular frequency of small oscillation of the bob of a simple pendulum when it is immersed in a liquid of density ρ. Assume the density of the bob as σ and length of the string as l.
View SolutionFind the period of small oscillations of a mathematical pendulum of length l if its point of suspension O moves relative to the Earth's surface in an arbitrary directio with a constant acceleration w (figure). Calculate that period if l=21cm,w=g/2, and the angle between the vectors w and g equals beat=120∘.
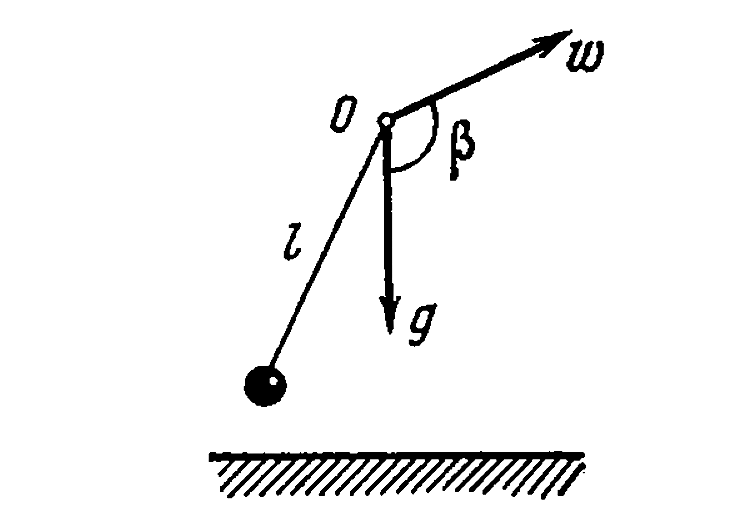
View SolutionA metal cube of 5cm side and relative density 9 is suspended by thread so as to be dompletely immersed in a liquid of density 1.2×103kgm−3. Find the tension in the thread.
View SolutionA solid copper sphere is suspended from a massless spring. The time period of oscillation of the system is 4 s. The sphere is now completely immersed in a liquid whose density is 1/8the that of brass. The sphere remains in liquid during oscillation. Now, the time period is
View SolutionA simple pendulum oscillating in air has a time period of √3s. Now the bob of the pendulum is completely immersed in a non-viscous liquid whose density is equal to 14th that of the material of the bob. The new time period of simple pendulum will be
View Solution