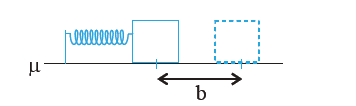
A mass-spring system oscillates such that the mass moves on a rough surface having coefficient of friction $μ$ . It is compressed by a distance a from its normal length and, on being released, it moves to a distance b from its equilibrium position. The decrease in anplitude for one half-cycle (-a to b) is

A

$\frac{μ m g}{k}$

B

$\frac{2 μ m g}{k}$

C

$\frac{μ g}{k}$

D

$\frac{k}{μ m g}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

(b) From -a to b decrease in elastic potential energy
= work done against friction.
$∴ \frac{1}{2} k a^{2} - \frac{1}{2} k b^{2} = μ m g (a + b)$
or $(a - b) = \frac{2 μ m g}{k}$
$∴ Decrease in amplitude = \frac{2 μ m g}{k}$

|

Updated on:21/07/2023

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A body of mass m moves with a velocity v on a surface whose friction coefficient is $μ$ . If the body covers a distance s, before it comes to rest, then v will be $:$
A $\sqrt{2 μ g s}$
B $\sqrt{μ g s}$
C $\sqrt{μ g s / 2}$
D $\sqrt{3 μ g s}$
Question 2 - Select One
A vehicle of mass $M$ is moving on a rough horizontal road with a momentum P If the coefficient of friction between the tyres and the road is $μ$ is then the stopping distance is .
A $\frac{P}{2 μ M g}$
B $\frac{P^{2}}{2 μ M g}$
C $\frac{P^{2}}{2 μ M^{2} g}$
D $\frac{P^{2}}{2 μ M^{2} g}$
Question 3 - Select One
A block of mass $m = 0.1 k g$ is connceted to a spring of unknown spring constant k. It is compressed to a distance x from its equilibrium position and released from rest. After approaching half the distance $(\frac{x}{2})$ from the euilibrium position, it hits another block and comes to rest momentarily, while the other block moves with velocity $3 m s^{- 1}$ . The total initial energy of the spring is :
A0.6 J
B0.8 J
C1.5 J
D0.3 J

DC PANDEY-WORK, ENERGY AND POWER-EXERCISES(TAKING IT TOGETHER)

Home
Ask Doubt
Profile