Let us consider the Example 3 of Section 3.2. Will the rails cross each other?
To determine whether the two rails represented by the equations x+2y−4=0 and 2x+4y−12=0 cross each other, we need to analyze the equations step by step.
Step 1: Write down the equations
We have two equations:
1. x+2y−4=0 (Equation 1)
2. 2x+4y−12=0 (Equation 2)
Step 2: Solve Equation 1 for x
From Equation 1, we can express x in terms of y:
x=4−2y
Step 3: Substitute x in Equation 2
Now, we substitute the expression for x into Equation 2:
2(4−2y)+4y−12=0
Step 4: Simplify the equation
Expanding the equation gives:
8−4y+4y−12=0
Combining like terms:
8−12=0
This simplifies to:
−4=0
Step 5: Analyze the result
The equation −4=0 is a contradiction; it is never true. This indicates that there are no common solutions to the two equations.
Conclusion
Since there are no common solutions, the two rails represented by the equations do not cross each other.
Final Answer
No, the rails will not cross.
---
We have two equations:
1. x+2y−4=0 (Equation 1)
2. 2x+4y−12=0 (Equation 2)
From Equation 1, we can express x in terms of y:
x=4−2y
Now, we substitute the expression for x into Equation 2:
2(4−2y)+4y−12=0
Expanding the equation gives:
8−4y+4y−12=0
Combining like terms:
8−12=0
This simplifies to:
−4=0
The equation −4=0 is a contradiction; it is never true. This indicates that there are no common solutions to the two equations.
Since there are no common solutions, the two rails represented by the equations do not cross each other.
No, the rails will not cross.
Topper's Solved these Questions
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:EXERCISE 3.3
Explore 3 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:EXERCISE 3.6
Explore 2 Videos
- PAIR OF LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:PAIR OF LINEAR EQUATIONS IN TWO VARIABLESExercise:Exercise 3.2
Explore 7 Videos
- NCERT THEOREMSBook:NCERTChapter:NCERT THEOREMSExercise:THEOREM 10.1
Explore 2 Videos
- POLYNOMIALSBook:NCERTChapter:POLYNOMIALSExercise:EXERCISE 2.4
Explore 5 Videos
Similar Questions
Let us consider Example 2 in Section 3.3, i.e., the cost of 2 pencils and 3 erasers is Rs 9 and the cost of 4 pencils and 6 erasers is Rs 18. Find the cost of each pencil and each eraser.
View SolutionTwo trains whose speed is in the ratio of 3: 4 are going in opposite directions on parallel tracks. If each train takes 3 seconds to cross a pole, then in how much time will they cross each other?
View Solution
Knowledge Check
Two trains of equal lengths take 3 seconds and 4 seconds in crossing a telegraph pole. In how much time will these trains cross each other while moving in opposite direction?
A1 secB7 secCsqrt 12 secD3 (3)/7 secTwo trains one 160 m and the other 140 m long are running in opposite directions on parallel rails, the first at 77 km an hour and the other at 67 km an hour. How long will they take to cross each other?
A7 secondsB712 secondsC6 secondsD10 secondsTwo trains, one 160m and the other 140m long are running in opposite directions on parallel rails, the first at 77km an hour and the other at 67km an hour. How long will they take to cross each other?
A7 secondsB712 secondsC6 secondsD10 seconds
Let us factorise the foll x3−3x+2
View SolutionTwo trains 160 m and 140 m long are running in opposite directions on parallel rails, the first at 77 km/hr and the other at 67 km/hr. How long will they take to cross each other?
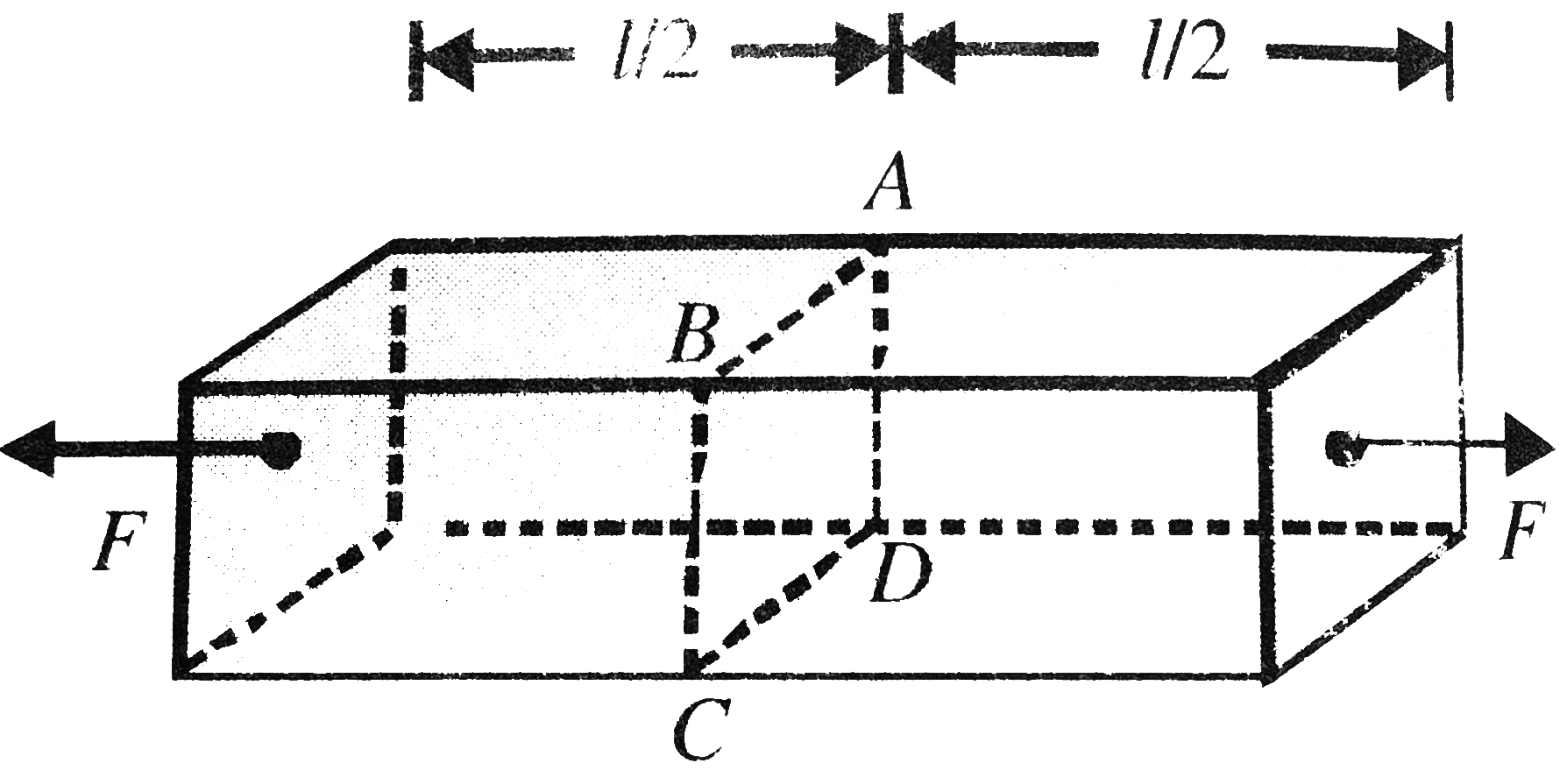
View SolutionTwo equal and opposite point forces applied at mid- points of the ends of a rod of square cross shown. Consider the dotted section ABCD. If the rod is cut across this Gloss section, the force exerted by the right part of the rod on left part across this cross section is
View SolutionIn the previous problem, the height at which the two stones will cross each other is
View SolutionLet us consider an esterification of isotopically labelled carboxylic acid:
CH3−O∣∣C−18OH+CH3CH2OhH+−−→(X)and(Y)
(X) and (Y) respectively are:
View Solution