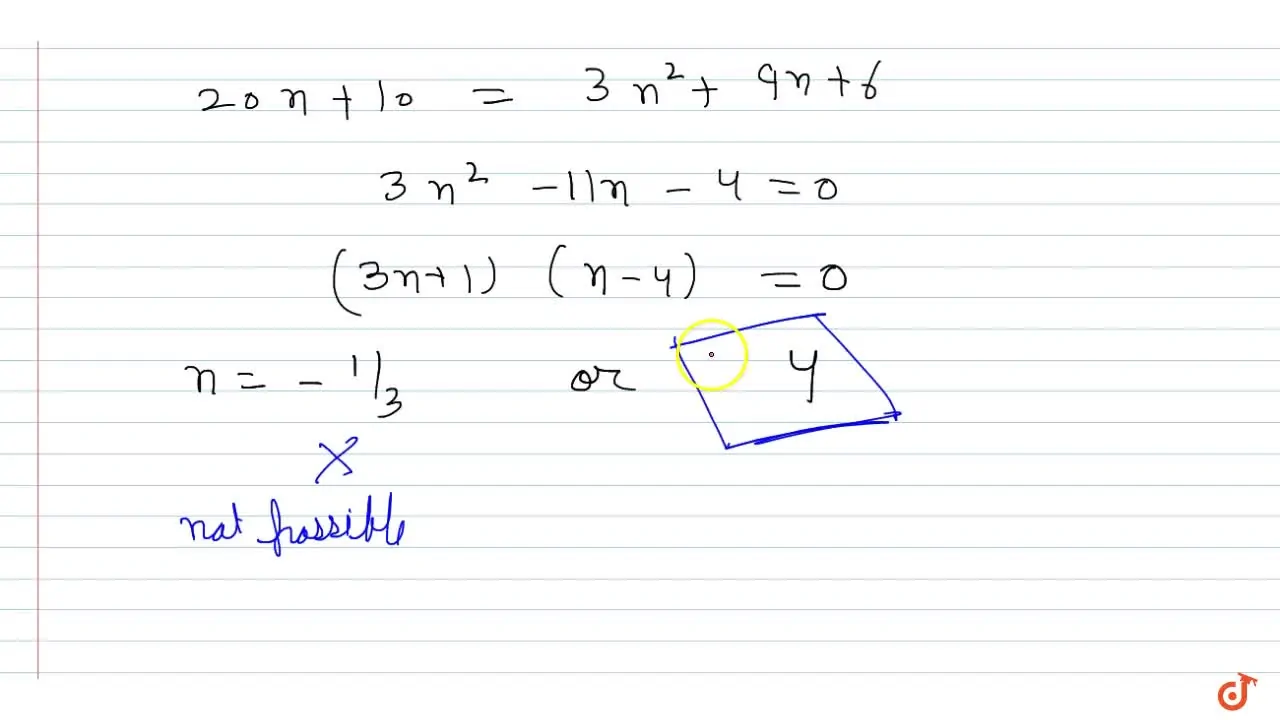
If 2n+1Pn−1:2n−1Pn=3:5, then find the value of n.
Video Solution
|
Answer
Step by step video & image solution for If "^(2n+1)P_(n-1):^(2n-1)P_n=3:5, then find the value of ndot by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Similar Questions
If ^(2n+1)Pn−1:2n−1Pn=3:5, then the value of n is
View SolutionIf (2n+1)Pn−1:2n−1Pn=3:5,find n.
View SolutionIF 2n+1Pn−1:2n−1 Pn=3:5 then n is equal to
View SolutionIF 2n+1Pn−1:2n−1 Pn=3:5 then n is equal to
View SolutionIf 2n+1Pn−1:2n−1Pn=3:5the possible value of n will be :
View SolutionIf 2n−1Pn:2n+1Pn−1=22:7, find n.
View SolutionIf 25n^(−1)+100=52n^(−1), find the value of n.
View SolutionIf .nP4:.nP5=1:2, find the value of n.
View SolutionIf 2n+1Pn−1:2n−1Pn=3:5, then n = ___________.
View Solutionif .2n+1Pn−1:2n−1Pn=7:10, then .nP3 equals
View Solution