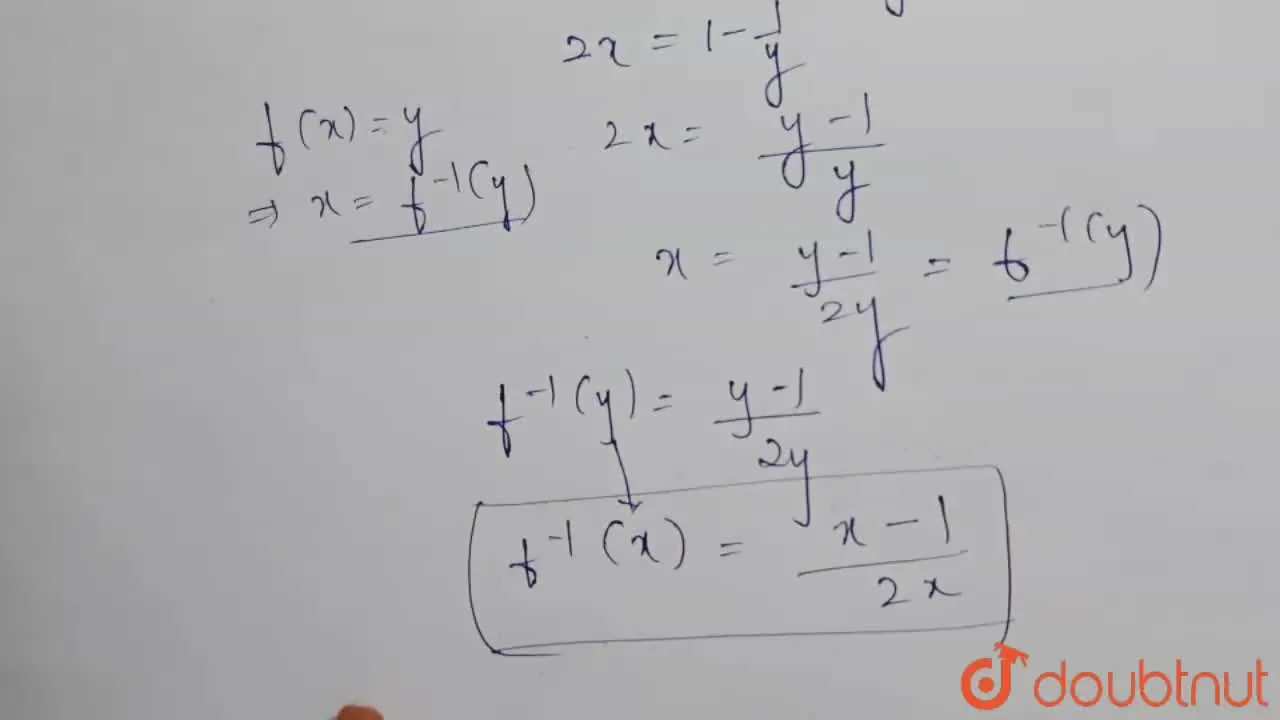Let x≠0,|x|<12 and f(x)=1+2x+4x2+8x3+…… then , f−1(x)= Solution in Telugu
x−12x
x−12
x+12
1−2x
The correct Answer is:A
Step by step video, text & image solution for Let x ne 0 , |x| lt 1/2 and f(x) = 1 + 2x + 4 x^2 + 8x^3 + …… then , f^(-1) (x) = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Match the following

The correct answer isView SolutionAn equation of a line whose segment between the coordinates axes is divided by the point (12,13) in the ratio 2:3 is
09:13View SolutionLet f(x)={2x+a,x≥−1bx2+3,x<−1
and g(x)={x+4,0≤x≤4−3x−2,−2<x<0
If the domain of g(f(x)) is [−1,4], then02:12View SolutionLet f(x)={2x+a,x≥−1bx2+3,x<−1
and g(x)={x+4,0≤x≤4−3x−2,−2<x<0
If a=2andb=3, then the range of g(f(x)) is02:38View SolutionIf f(x)=(25−x4)1/4for 0<x<√5,thenf(f(12))=
01:21View SolutionLet f(x+1x)=x2+1x2,(x≠0) then f(x) equals
01:19View SolutionLet f(x+1x)=x2+1x2,x≠0, then the value of f(x) is-
02:12View SolutionIf f(x)=1x−1 where x≠0, then: f(x)−2.f(2x)=
04:35View SolutionIf f(x)=(25−x4)1/4 for 0<x<√5 then f(f(12))=
01:17View SolutionLet f(x+1x)=x2+1x2,x≠0, then f(x)=?
01:37View SolutionLet f(x)={2x+a,x≥−1bx2+3,x<−1
and g(x)={x+4,0≤x≤4−3x−2,−2<x<0
If the domain of g(f(x)) is [−1,4], then04:31View SolutionLet f(x)={2x+a,x≥−1bx2+3,x<−1
and g(x)={x+4,0≤x≤4−3x−2,−2<x<0
If a=2andb=3, then the range of g(f(x)) isView SolutionIf f(x)=(25-x^(4))^(1//4) for 0 lt x lt sqrt5 then f(f((1)/(2)))=
View SolutionLet f(x){2x+a,x≥−1bx2+3x<−1 and g(x)={x+4,0≤x≤4−3x−2−2<x<0
If the domain of g(f(x)) is [-1, 4], then01:28View SolutionLet f(x){2x+a,x≥−1bx2+3x<−1 and g(x)={x+4,0≤x≤4−3x−2−2<x<0
If a=2 and b=3, then the range of g(f(x)) is02:53View Solution