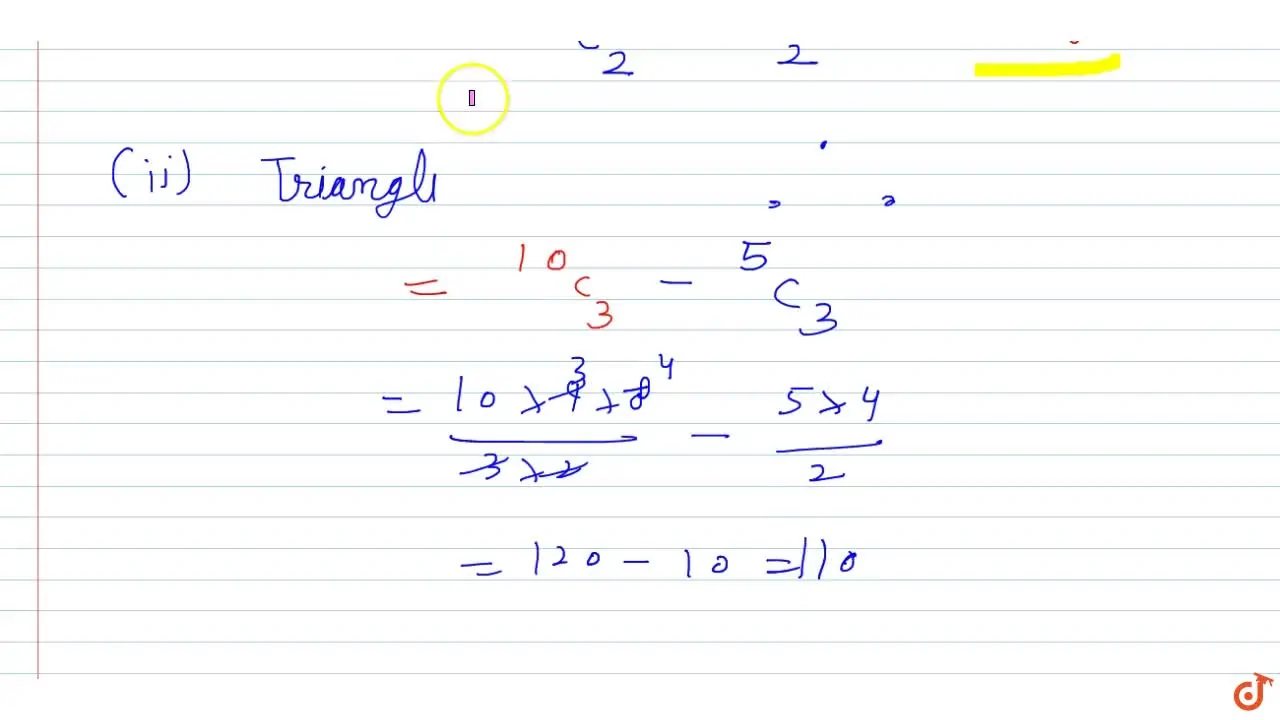
There are 10 points on a plane of which 5 points are collinear. Also, no three of the remaining 5 points are collinear. Then find (i) the number of straight lines joining these points: (ii) the number of triangles, formed by joining these points.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:(i) 36 (ii) 110

Updated on:21/07/2023
|
Answer

Step by step video, text & image solution for There are 10 points on a plane of which 5 points are collinear. Also, no three of the remaining 5 points are collinear. Then find (i) the number of straight lines joining these points: (ii) the number of triangles, formed by joining these points. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023
Class 11MATHSPERMUTATIONS AND COMBINATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • There are 10 points in a plane, out of which 5 are collinear. Find the number of straight lines formed by joining them.

    A36
    B45
    C30
    D35

  • There are 14 points in a plane, out of which 4 are collinear. Find the number of triangles made by these points.

    A364
    B360
    C368
    D365

  • There are 12 points in a plane of which 5 are collinear. Except these five points no three are collinear, then

    AThe number of quadrilaterals one can form using these points is 420
    Bthe number of Deltas one can form using these points is 210
    Cthe number of lines one can form using these points is 57
    Dthe number of lines one can form using these points is 56