Prove that $\sqrt{2}$ is an irrational number.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:N/a

We will use the division method to find the square root of 2.

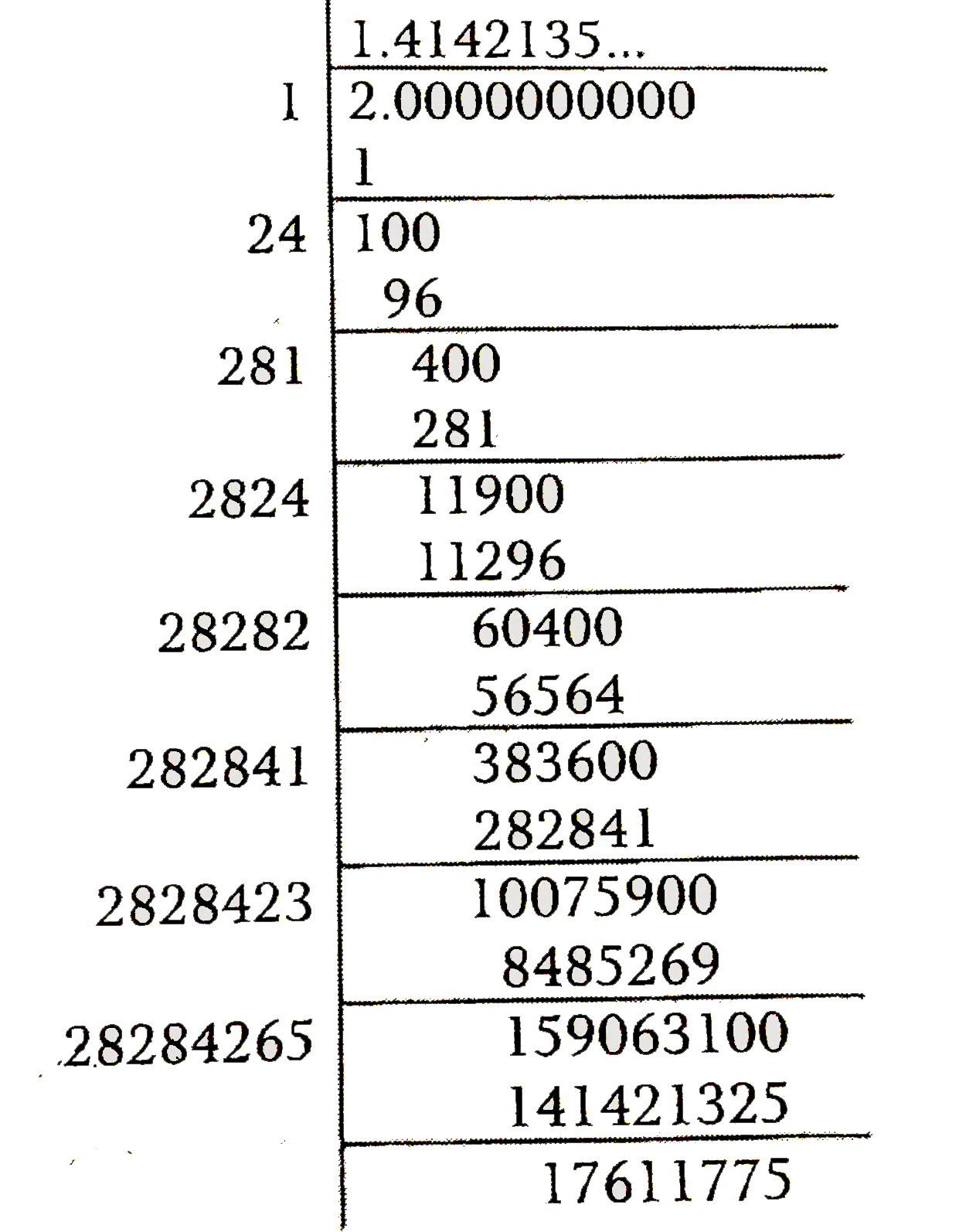
$\sqrt{2} = 1.4142135 . . .$
Alternative Method : We are going to prove it by the technique called "method of contradiction".
Let us assume that $\sqrt{2}$ is rational.
Then $\sqrt{2}$ can be expressed as
$\sqrt{2} = \frac{p}{q}, p, q \in Z, q \neq 0$
(in simplest form i.e., no factor other 1 is common to both p and q)
Squaring on both sides, we get
$2 = \frac{p^{2}}{q^{2}} \Rightarrow p^{2} = 2 q^{2}$ . . .(1)
$But 2 divides 2 q^{2}$
$\Rightarrow 2 divides p^{2}$ [form (1)]
$\Rightarrow 2 divides p (\begin{matrix} ∵ 2 divides 64 \Rightarrow 2 divides 8 also 2divides 36 \Rightarrow 2 divides 6 also etc . \end{matrix})$
$So, let p = 2 m, m \in Z$ . . .(2)
$\Rightarrow p^{2} = 4 m^{2} (on squaring)$
$\Rightarrow 2 q^{2} = 4 m^{2} [o m (1)]$
$\Rightarrow q^{2} = 2 m^{2} . . . (3)$
But 2 divides $2 m^{2}$
$\Rightarrow 2 divies q^{2}$ [from (3)]
$\Rightarrow 2 divides q$
Let q = 2n, n $\in Z$ . . . (4)
From (2) and (4), we see that 2 divides p and q both. But we have already assumed that p and q has no common factor other than 1.
So, this contradicts our assumption that $\sqrt{2}$ is a rational number.
It means our supposition is wrong.
Therefore, $\sqrt{2}$ is not a rational number.
Hence, $\sqrt{2}$ is irrational.

|

Updated on:21/07/2023

Class 9 MATHS NUMBER SYSTEMS

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN-NUMBER SYSTEM-Revision Exercise (short Answer Questions)

Home
Ask Doubt
Profile