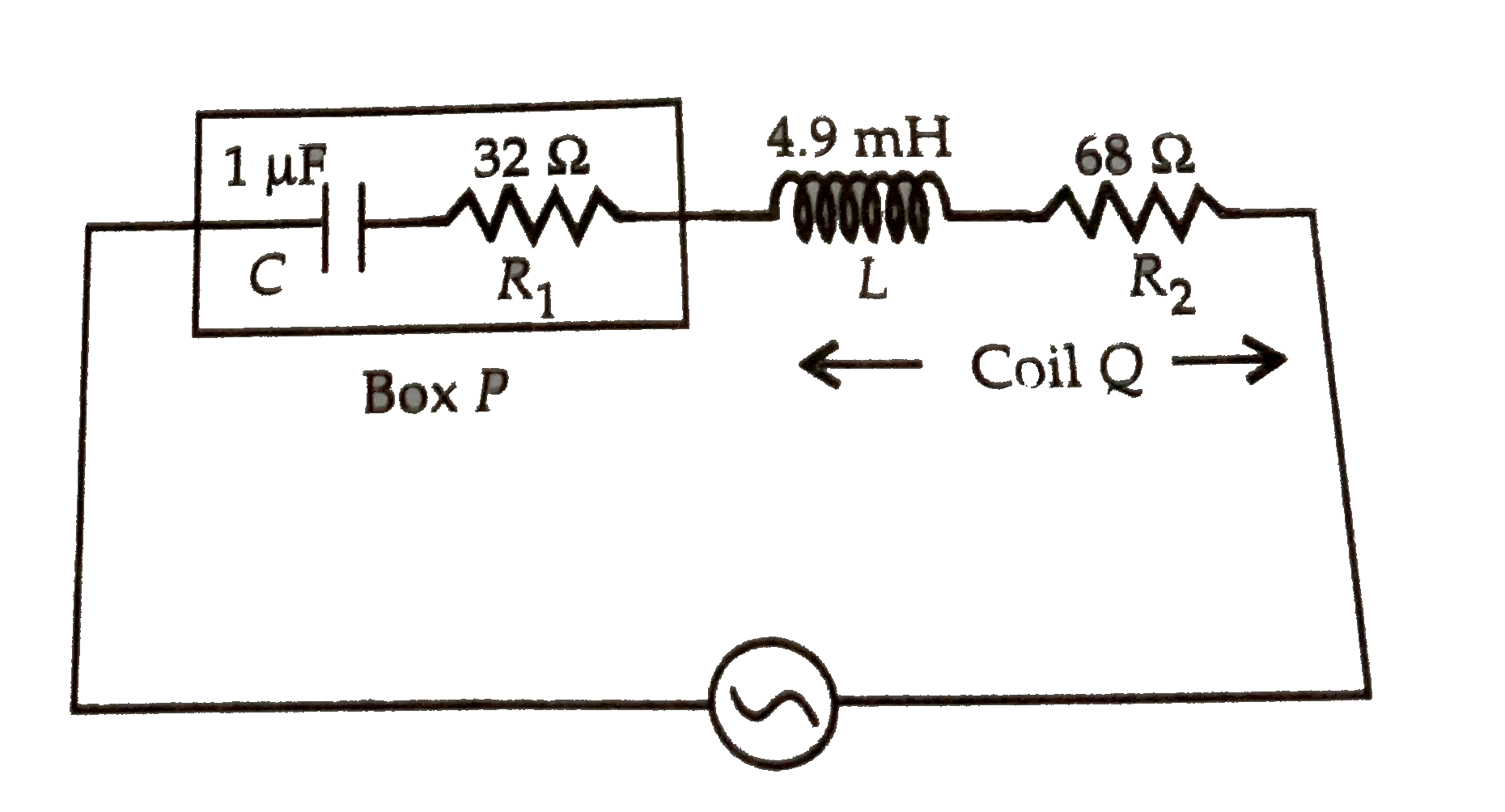
A box P and a coil Q are connected in series with an ac source of variable frequency. The emf of the source is constant at 10 V. Box P contains a capacitance of 32Ω. Coil Q has a self inductance of 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted so that maximum current flows in P and Q.
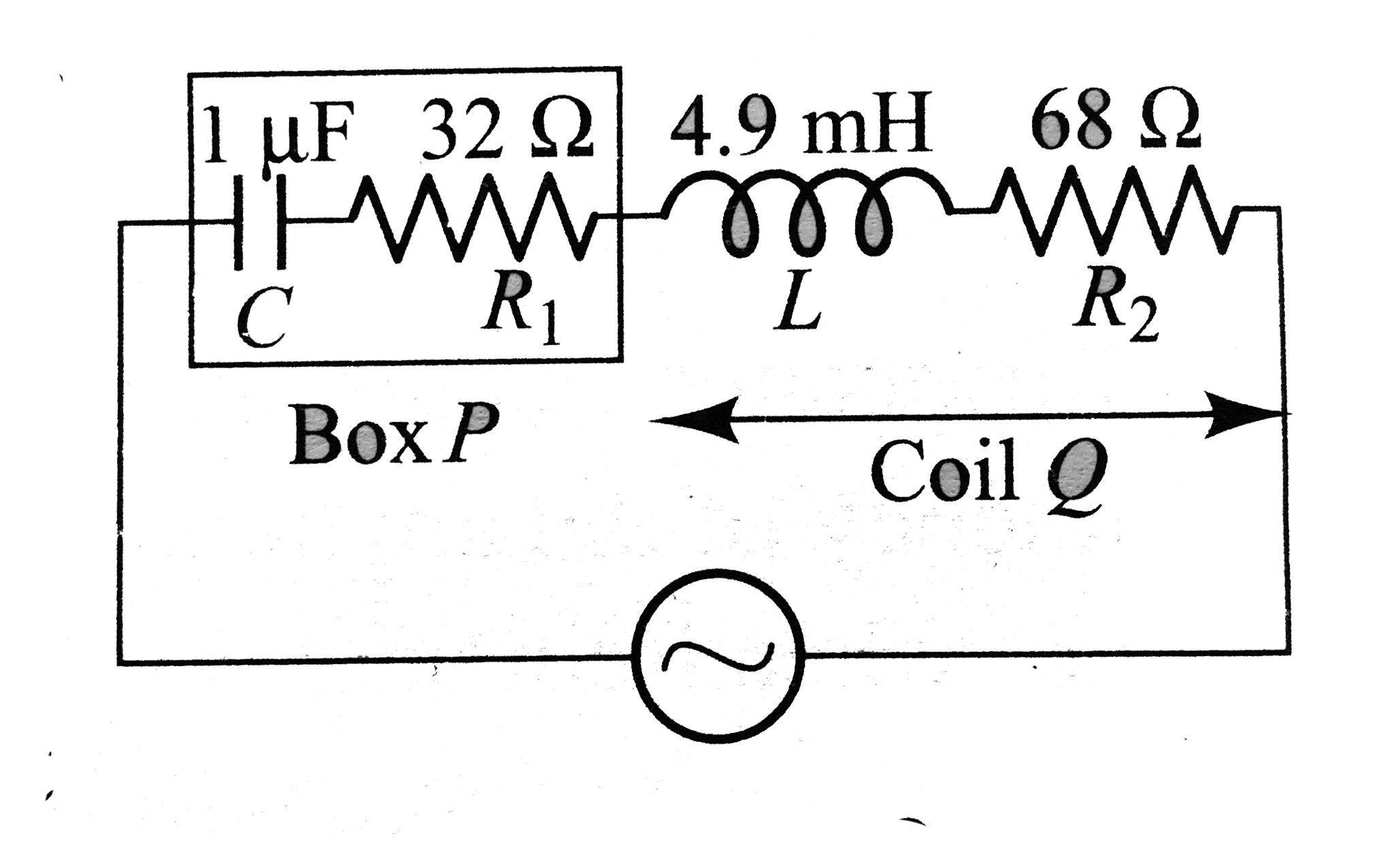
The impedance of Q at this frequency is
9.76V,8.92V
6.29V,7.96V
7.70V,10.92V
7.70V,9.76V
The correct Answer is:D
As this circuit is series LCR circuit current will be maximum at resonance,
∴ Resonance frequency
ω=1√LC=1√(4.9×10−3)(10−6)=1057rad s−1
and current Imax=ε0R=10(32+68)=110A
So the impedance for box P
ZP=[R21+(1ωC)2]1/2=[(32)2+(7105×10−6)2]1/2
=√5924=77Ω
and for coil Q,ZQ=[R22+(ωL)2]1/2
=[(68)2+(1057×4.9×10−3)2]1/2=√9524=97.6Ω
and hence , VP=ImaxZP=110×(77)=7.70V
(potential drop across P)
and VQ=ImaxZQ=110×(97.6)=9.76V
(potential drop across Q)
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - NCERT FINGERTIPSCHAPTER - ALTERNATING CURRENT EXERCISE - NCERT Exemplar
7 Videos
- ALTERNATING CURRENT BOOK - NCERT FINGERTIPSCHAPTER - ALTERNATING CURRENT EXERCISE - Assertion And Reason
15 Videos
- ALTERNATING CURRENT BOOK - NCERT FINGERTIPSCHAPTER - ALTERNATING CURRENT EXERCISE - Transformers
16 Videos
- ATOMS BOOK - NCERT FINGERTIPSCHAPTER - ATOMS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A box P and a coil Q are connected in series with an AC source of variable frequency.The EMF of source is constant at 10V.Box P contains a capacitance of 1μF in series with a resistance of 32Ω.Coil Q has a self inductance 4.9mH and a resistance of 68Ω.The frequency is adjusted so that the maximum current flows in P and Q.Find the impedance of P and Q at this frequency.Also find the voltage across P and Q respectively.
View SolutionA box P and a coil Q are connected in series with an ac source of variable freguency The emf of the source is constant at 28V The frequency is so adjusted that the maximum current flows in P and Q Find
(a) impedance of P and Q at this frequency
(b) voltage across P and Q
 .
.
View Solution
Knowledge Check
A box P and a coil Q are connected in series with an ac source of variable frequency. The emf of the source is constant at 10 V. Box P xontains a capacitance of 32Ω. Coil Q has a self inductance of 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted so that maximum current flows in P and Q.
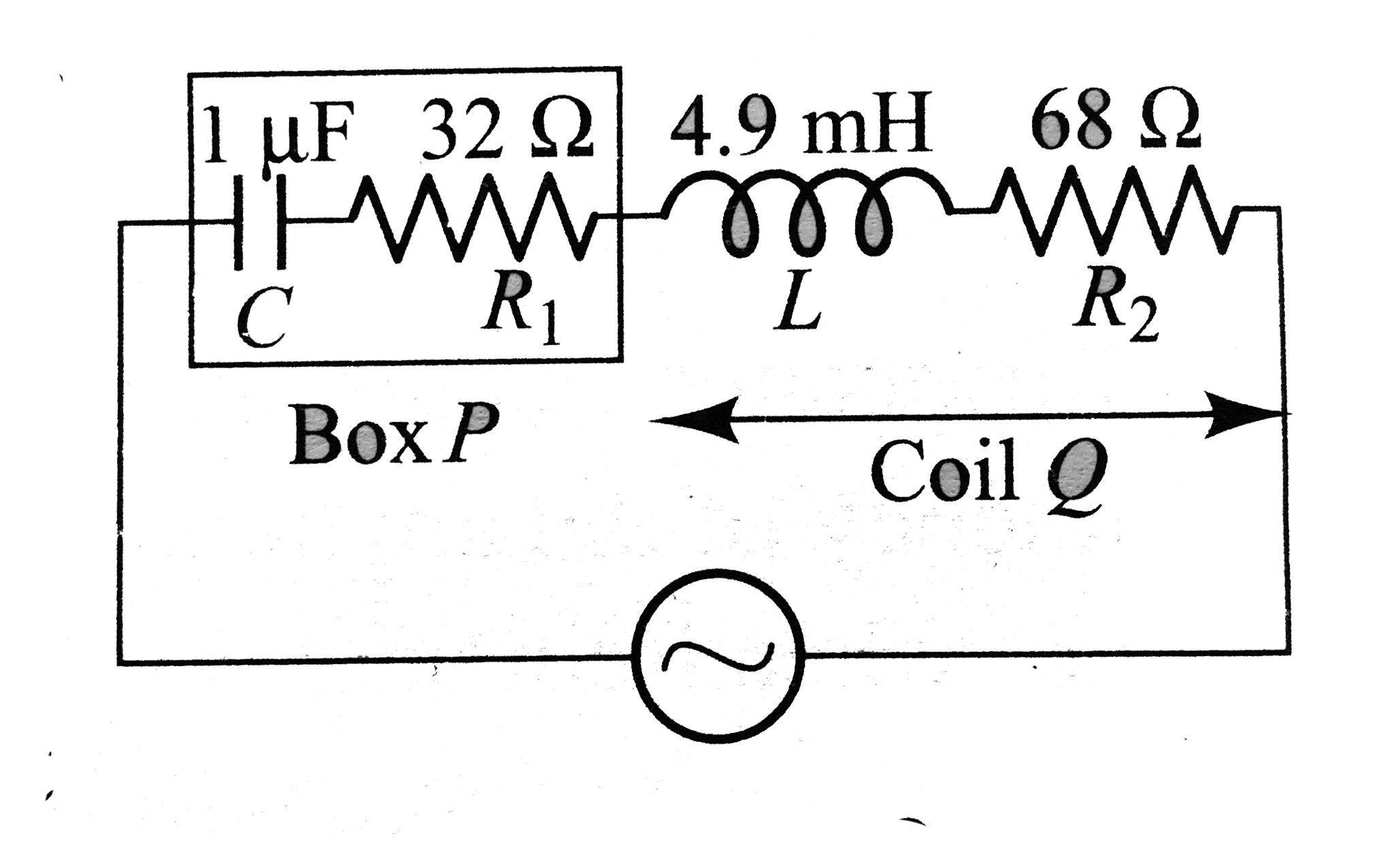
The impendance of Q at this frequency isA200ΩB√1350ΩC55ΩD√9524ΩA box P and a coil Q are connected in series with an ac source of variable frequency. The emf of the source is constant at 10 V. Box P xontains a capacitance of 32Ω. Coil Q has a self inductance of 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted so that maximum current flows in P and Q.
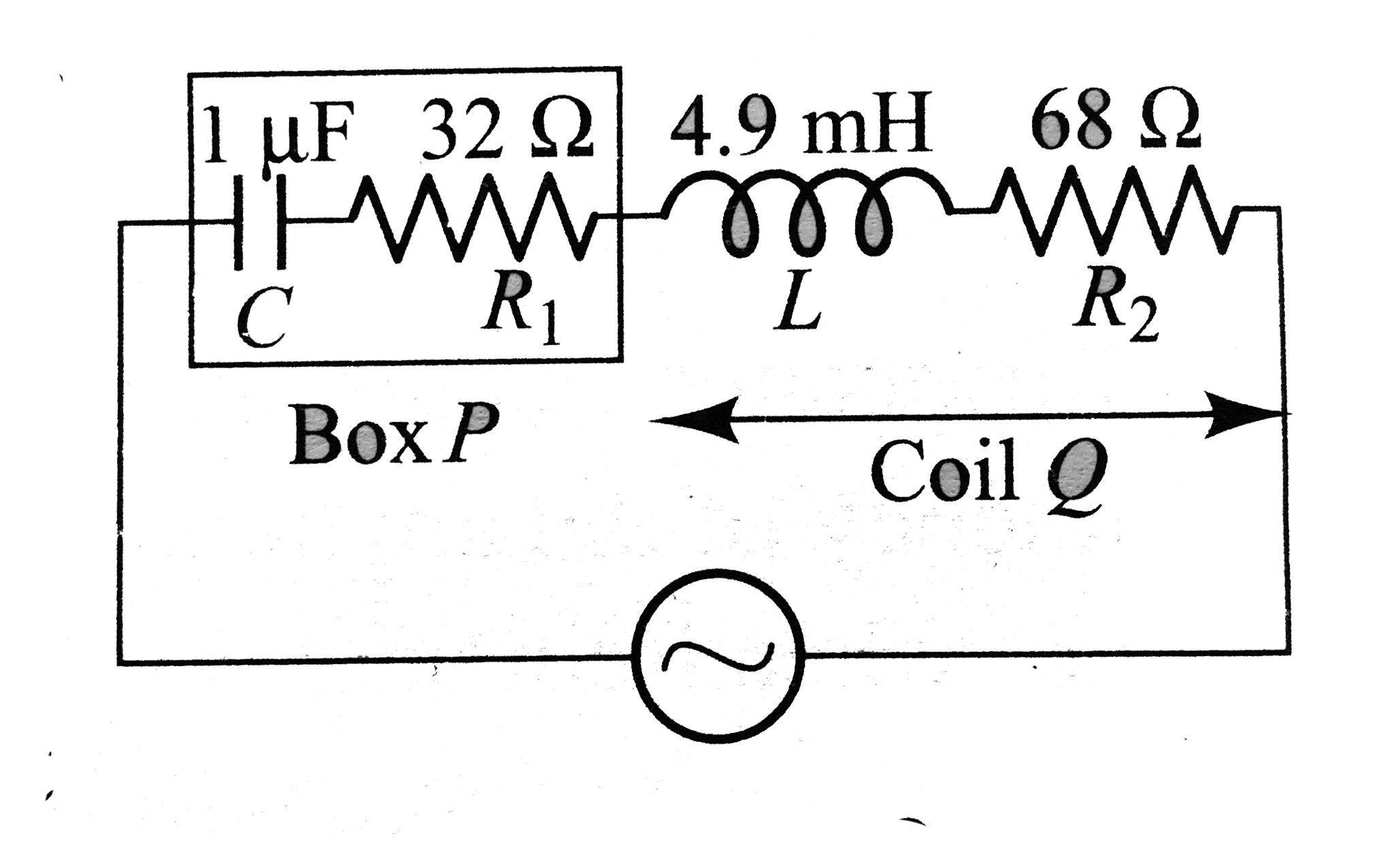
The impedance of P at this frequency isA77ΩB36ΩC40ΩD125ΩA box P and a coil Q are connected in series with an ac source of variable frequency. The emf of the source is constant at 10 V. Box P xontains a capacitance of 32Ω. Coil Q has a self inductance of 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted so that maximum current flows in P and Q.
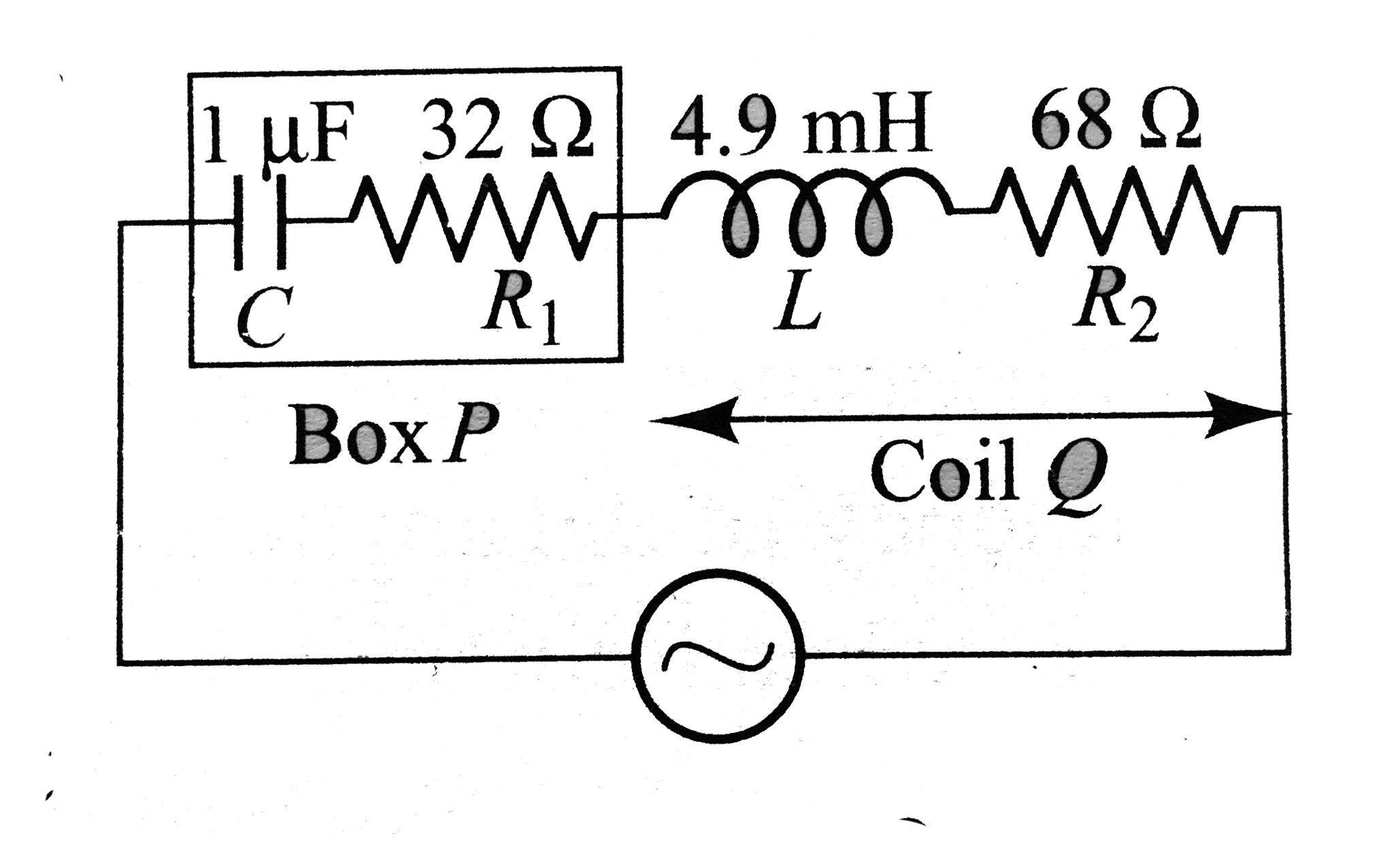
the voltage across Q isA20 VB√135010VC5.5VD√952410V
A box P and a coil Q are connected in series with an ac source of variable frequency. The emf of the source is constant at 10 V. Box P xontains a capacitance of 32Ω. Coil Q has a self inductance of 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted so that maximum current flows in P and Q.
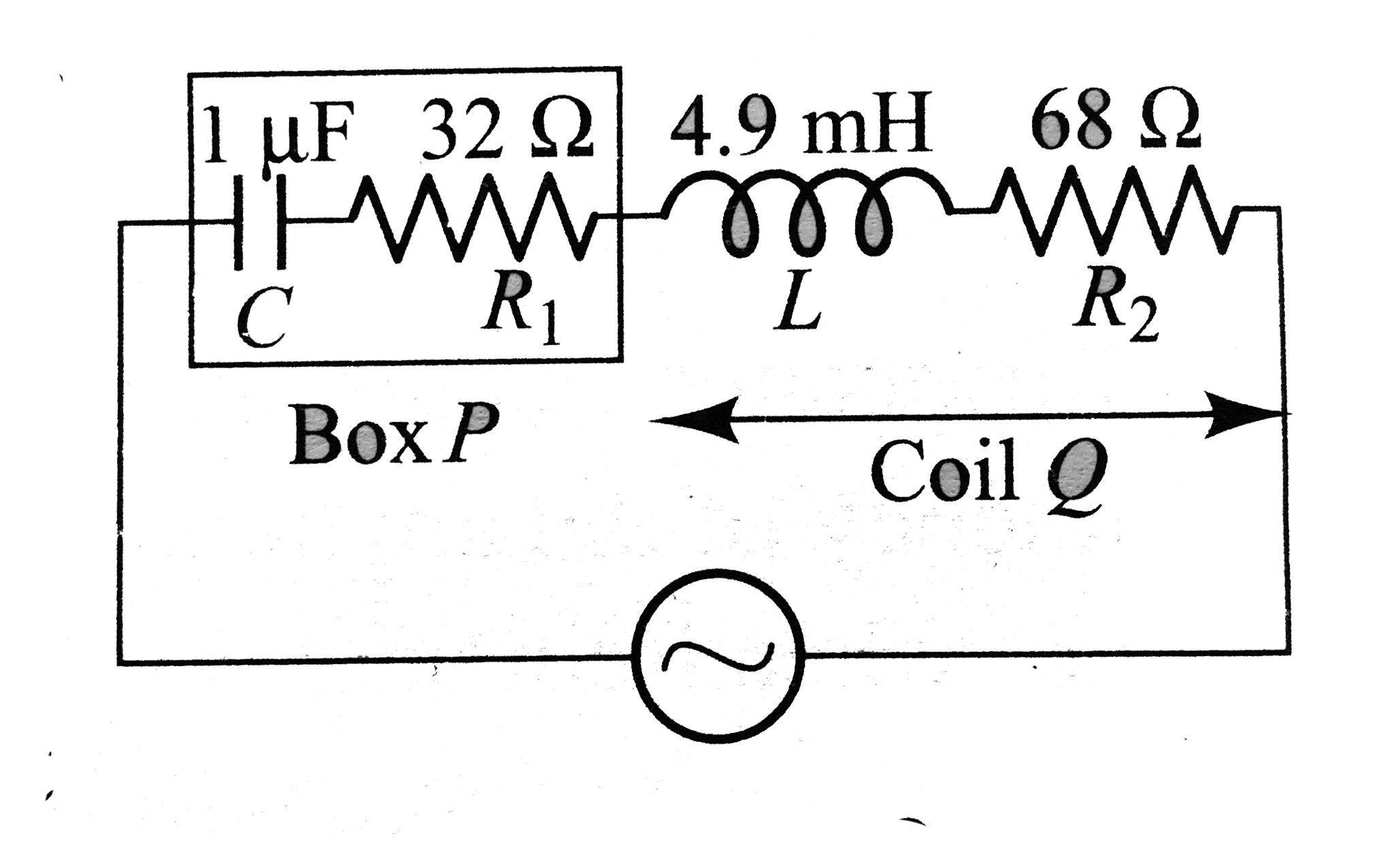
The voltage across P is
View SolutionA box P and a coil Q are connected in series with an AC source of variable frequency. The emf of the source is constant at 10V. Box P contains a capacitance of 1μF in series with a resistance of 32Ω. Coil Q has a self-inductance 4.9 mH and a resistance of 68Ω in series. The frequency is adjusted to that the maximum current flows in P and Q. At this frequency
(a) The impedance of P is 77Ω
(b) The impedance of Q is 85Ω
(c ) Voltage across P is 7.7V
(d) Voltage across Q is 9.7V
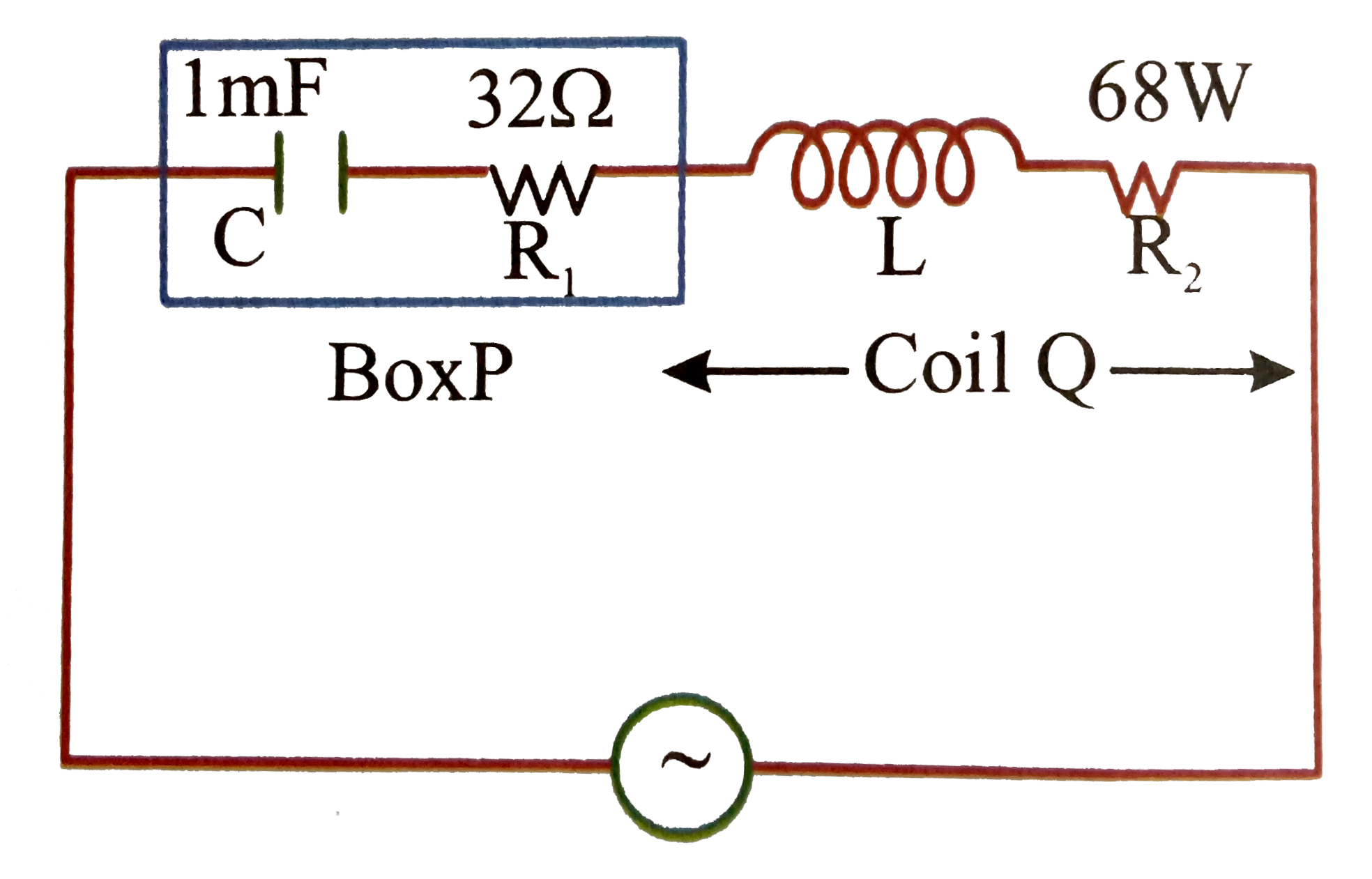
View SolutionIt is known to all of you that the impedance of a circuit is dependent on the frequency of source. In order to study the effect of frequency on the impedance, a student in a lab took 2 impedance boxes P and Q and connected them in series with an AC source of variable frequency. The emf of the source is constant at 10V Box P contains a capacitance of 1μF in series with a resistance of 32Ω. And the box Q has a coil of self-inductance 4.9mH and a resistance of 68Ωin series. He adjusted the frequency so that the maximum current flows in P and Q. Based on his experimental set up and the reading by him at various moment, answer the following questions.
Impedance of box P at the above frequency is
View SolutionIt is known to all of you that the impedance of a circuit is dependent on the frequency of source. In order to study the effect of frequency on the impedance, a student in a lab took 2 impedance boxes P and Q and connected them in series with an AC source of variable frequency. The emf of the source is constant at 10V Box P contains a capacitance of 1μF in series with a resistance of 32Ω. And the box Q has a coil of self-inductance 4.9mH and a resistance of 68Ωin series. He adjusted the frequency so that the maximum current flows in P and Q. Based on his experimental set up and the reading by him at various moment, answer the following questions.
Power factor of the circuit at maximum current is
View SolutionIt is known to all of you that the impedance of a circuit is dependent on the frequency of source. In order to study the effect of frequency on the impedance, a student in a lab took 2 impedance boxes P and Q and connected them in series with an AC source of variable frequency. The emf of the source is constant at 10V Box P contains a capacitance of 1μF in series with a resistance of 32Ω. And the box Q has a coil of self-inductance 4.9mH and a resistance of 68Ωin series. He adjusted the frequency so that the maximum current flows in P and Q. Based on his experimental set up and the reading by him at various moment, answer the following questions.
The angular frequency foer which he detects maximum current in the circuit is
View Solution
