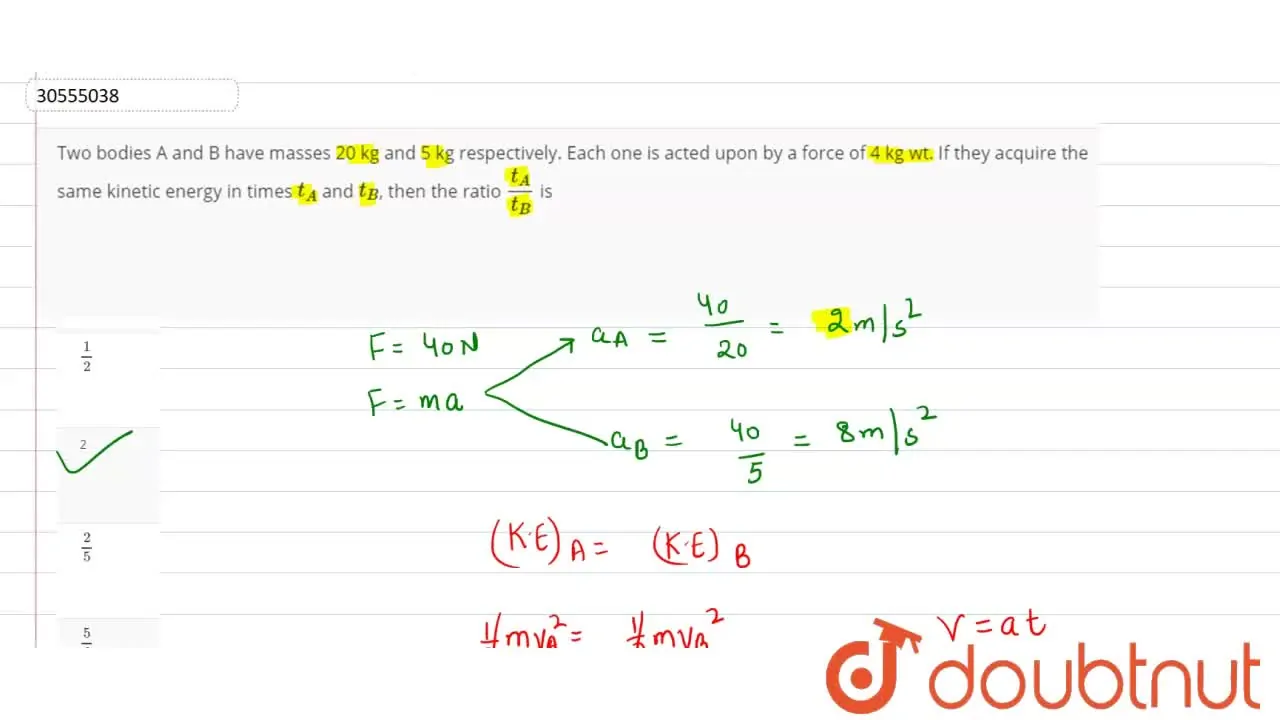
Two bodies A and B have masses 20 kg and 5 kg respectively. Each one is acted upon by a force of 4 kg wt. If they acquire the same kinetic energy in times $t_{A}$ and $t_{B}$ , then the ratio $\frac{t_{A}}{t_{B}}$ is

A

$\frac{1}{2}$

B

2

C

$\frac{2}{5}$

D

$\frac{5}{6}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To solve the problem, we need to find the ratio of the times $t_{A}$ and $t_{B}$ for two bodies A and B, which have masses of 20 kg and 5 kg respectively, and are acted upon by a force of 4 kg wt.
1. Convert the Force to Newtons:
The force given is 4 kg wt. To convert this to Newtons, we use the conversion factor $1 kg wt = 9.8 N$ .
$F = 4 kg wt \times 9.8 N/kg wt = 39.2 N \approx 40 N$
Hint: Remember to convert kg wt to Newtons using the acceleration due to gravity.
2. Calculate the Acceleration of Each Body:
Using Newton's second law, $F = m a$ , we can find the acceleration of each body.
- For body A:
$a_{A} = \frac{F}{m_{A}} = \frac{40 N}{20 kg} = 2 {m/s}^{2}$
- For body B:
$a_{B} = \frac{F}{m_{B}} = \frac{40 N}{5 kg} = 8 {m/s}^{2}$
Hint: Use the formula $a = \frac{F}{m}$ to find the acceleration of each body.
3. Relate Kinetic Energy and Velocity:
The kinetic energy $K E$ for both bodies is given by:
$K E_{A} = \frac{1}{2} m_{A} v_{A}^{2} and K E_{B} = \frac{1}{2} m_{B} v_{B}^{2}$
Since $K E_{A} = K E_{B}$ , we can set the equations equal to each other:
$\frac{1}{2} m_{A} v_{A}^{2} = \frac{1}{2} m_{B} v_{B}^{2}$
This simplifies to:
$m_{A} v_{A}^{2} = m_{B} v_{B}^{2}$
Hint: Remember that kinetic energy is proportional to the mass and the square of the velocity.
4. Express Velocity in Terms of Acceleration and Time:
The velocity of each body can be expressed as:
$v_{A} = a_{A} t_{A} and v_{B} = a_{B} t_{B}$
Substituting these into the kinetic energy equation gives:
$m_{A} (a_{A} t_{A})^{2} = m_{B} (a_{B} t_{B})^{2}$
Hint: Use the kinematic equation $v = a t$ to express velocity in terms of acceleration and time.
5. Rearranging the Equation:
Substituting the values of $m_{A}$ , $m_{B}$ , $a_{A}$ , and $a_{B}$ :
$20 (2 t_{A})^{2} = 5 (8 t_{B})^{2}$
Simplifying this gives:
$20 \cdot 4 t_{A}^{2} = 5 \cdot 64 t_{B}^{2}$
$80 t_{A}^{2} = 320 t_{B}^{2}$
Dividing both sides by 80:
$t_{A}^{2} = 4 t_{B}^{2}$
Hint: Simplify the equation step by step to isolate $t_{A}$ and $t_{B}$ .
6. Finding the Ratio of Times:
Taking the square root of both sides:
$\frac{t_{A}}{t_{B}} = \sqrt{4} = 2$
Hint: Remember that taking the square root of both sides will give you the ratio of the times.
Final Answer:
The ratio $\frac{t_{A}}{t_{B}}$ is $2$ .

Updated on:7/8/2024

|

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two blocks A and B have masses $m$ and $4 m$ , respectively. Each one is acted upon by a force $F$ . If they acquire the same kinetic energy in time $t_{A}$ and $t_{B}$ , then $(t_{A}) / (t_{B})$ is
A2
B $\frac{1}{2}$
C $3$
D $\frac{1}{3}$
A block of mass $m$ at rest is acted upon by a force $F$ for a time t. The kinetic energy of block after time t is
A $\frac{F t^{2}}{m}$
B $\frac{F^{2} t^{2}}{2 m}$
C $\frac{F t^{2}}{2 m}$
D $\frac{F^{2} t^{2}}{3 m}$
A particle of mass m at rest is acted upon by a force P for a time t. Its kinetic energy after an interval t is
A $\frac{p^{2} t^{2}}{m}$
B $\frac{p^{2} t^{2}}{2 m}$
C $\frac{p^{2} t^{2}}{3 m}$
D $\frac{p t}{2 m}$

NCERT FINGERTIPS-WORK , ENERGY AND POWER-Kinetic Energy

Home
Ask Doubt
Profile