Two rods of equal lengths(l) and equal mass M are kept along x and y axis respectively such that their centre of mass lie at origin. The moment of inertia about an line y = x, is
ml23
ml24
ml212
ml26
The correct Answer is:C
To find the moment of inertia of two rods of equal lengths L and equal masses M placed along the x-axis and y-axis respectively, about the line y=x, we can follow these steps:
1. Identify the Configuration:
- We have two rods: one along the x-axis and the other along the y-axis.
- Both rods have mass M and length L.
- The center of mass of the system is at the origin (0,0).
2. Moment of Inertia about the Axes:
- The moment of inertia Ix of a rod about an axis perpendicular to it through its center is given by:
Ix=112ML2
- For the rod along the x-axis, the moment of inertia about the x-axis is:
Ix=112ML2
- For the rod along the y-axis, the moment of inertia about the y-axis is:
Iy=112ML2
3. Using the Perpendicular Axis Theorem:
- The Perpendicular Axis Theorem states that for a planar body:
Iz=Ix+Iy
- Here, Iz is the moment of inertia about an axis perpendicular to the plane of the rods (z-axis).
- Therefore, we have:
Iz=112ML2+112ML2=16ML2
4. Finding Moment of Inertia about the Line y=x:
- The line y=x can be treated as a new axis. To find the moment of inertia about this line, we can use the fact that the moment of inertia about any axis can be calculated using the relationship:
Iy=x=12(Ix+Iy)
- Since Ix=Iy for our configuration, we can write:
Iy=x=12(112ML2+112ML2)=12(16ML2)=112ML2
5. Conclusion:
- The moment of inertia of the system about the line y=x is:
Iy=x=112ML2
Final Answer:
The moment of inertia about the line y=x is 112ML2.
---
- We have two rods: one along the x-axis and the other along the y-axis.
- Both rods have mass M and length L.
- The center of mass of the system is at the origin (0,0).
- The moment of inertia Ix of a rod about an axis perpendicular to it through its center is given by:
Ix=112ML2
- For the rod along the x-axis, the moment of inertia about the x-axis is:
Ix=112ML2
- For the rod along the y-axis, the moment of inertia about the y-axis is:
Iy=112ML2
- The Perpendicular Axis Theorem states that for a planar body:
Iz=Ix+Iy
- Here, Iz is the moment of inertia about an axis perpendicular to the plane of the rods (z-axis).
- Therefore, we have:
Iz=112ML2+112ML2=16ML2
- The line y=x can be treated as a new axis. To find the moment of inertia about this line, we can use the fact that the moment of inertia about any axis can be calculated using the relationship:
Iy=x=12(Ix+Iy)
- Since Ix=Iy for our configuration, we can write:
Iy=x=12(112ML2+112ML2)=12(16ML2)=112ML2
- The moment of inertia of the system about the line y=x is:
Iy=x=112ML2
The moment of inertia about the line y=x is 112ML2.
Similar Questions
Two rods of equal mass m and length l lie along the x & y axis with their centres origin. What is the moment of inertia of both about the line x=y.
View SolutionThree rods each of length L and mass M are placed along X, Y and Z axis in such a way that one end of each of the rod is at the origin. The moment of inertia of this system about Z axis is
View Solution
Knowledge Check
Three thin rods each of length Land mass M are placed along x, y and z-axes such that one of each rod is at origin. The moment of inertia of this system about z-axis is
A2.3ML2B(4ML^(2))/3C(5ML^(2))/3D(ML^(2))/3Two rods of equal lengths(l) and equal mass M are kept along x and y axis respectively such that their centre of mass lie at origin. The moment of inertia about an line y = x, is
AML23Bml24Cml212Dml26Three thin rods, each of length 2 m and mass 3 kg are placed along x, y and z axes, such that one end of each rod is at the origin. The moment of inertia of this system about the x axis is
A2kgm2B4kgm2C6kgm2D8kgm2
Three identical rods, each of mass m and length l are placed along x, y and z axis respectively. One end of each rod is at the origin. The moment of inertia of the rods x-axis will be
View SolutionThree rods each of length L and mass M are placed along X, Y and Z axis in such a way that one end of each of the rod is at the origin. The moment of inertia of this system about Z axis is
View SolutionA rod is placed along the line,y=2x with its centre at origin. The moment of inertia of the rod is maximum about.
View SolutionIn the diagram shown below all three rods are of equal length L and equal mass M. The system is rotated such that rod B is the axis. What is the moment of inertia of the system ?
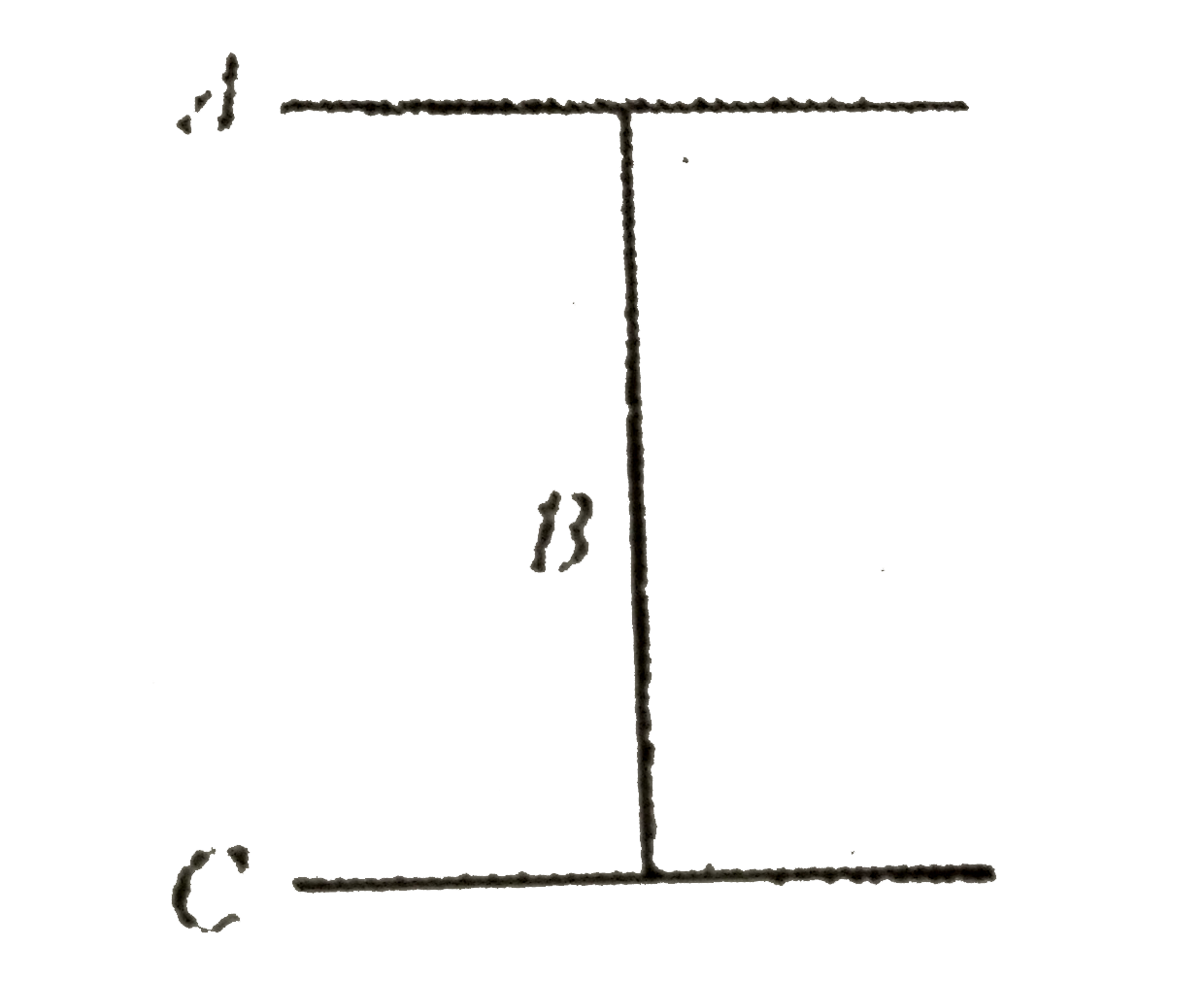
View SolutionParticles each of mass 1kg are placed at 1m, 2m and 4m on X-axis with respect to origin. Then moment of inertia of the system about Y-axis is
View Solution