Question

Volume and surface area of sphere

Explanation

Volume and Surface Area of Sphere

A sphere is a solid object having a curved surface in a manner that every point on the surface is equidistant from the centre. A sphere is a round geometrical object in three-dimensional space, just like a surface of a ball. We are familiar with the 2-Dimensional geometrical shape called a circle, a sphere is a three-dimensional figure, and it can be defined as a set of points lying at the same distance from the centre point in the three-dimensional space. The points that lie on the opposite sides of a sphere is termed as "antipodes".

Surface Area of a Sphere

The surface area of a sphere is characterized as the measure of space covered by the sphere surface. A sphere is a three-dimensional solid object with a round structure. Sphere has a three-dimensional structure that forms by turning a disk that is round with one of the diagonals. To understand the concept of the surface area better, let us take an example of painting a ball. To paint the entire surface of the ball, the quantity of paint required has to be known beforehand. Thus the area of each face has to be known to ascertain the paint amount for painting the same. We characterize this term as the total surface area. The surface area of a sphere is equivalent to the areas of the entire face enclosing it.

Formula for Surface Area of a Sphere

To find the surface area of a sphere, we use the formula,

A = 4 π r² sq. units

The area of any 3-D geometrical object can be classified into three types.

Lateral Surface Area: It is the area of all the surfaces other than bases, top and bottom.

Curved Surface Area: It is the area of all the curved surfaces of the solid figure.

Total Surface Area: It is the area of all the faces, including the top and bottom face of the solid figure.

Since the sphere does not have any flat surface, so the total surface area of a sphere becomes the same as the curved surface area of a sphere

Volume of Sphere

The volume of the sphere is the number of cubic units required to fill the space inside a sphere. The sphere is distinguished as the three-dimensional round solid figure where every point on its surface is equidistant from a central point. The fixed equidistant space is known as the radius of the sphere as well as the fixed point is known as the centre of the sphere. At the point, once the circle is rotated, we will observe a different shape of that circle. Thus, the spherical three-dimensional shape is obtained from the rotation of the circle.

The volume of a spherical object could be found via Archimedes principle. It says that when a solid is immersed in a totally filled beaker, the amount of the good object can be determined by measuring the quantity of water that emanates in the beaker is equivalent to the volume of the strong ( in the special case, a spherical object).

Formula for Volume of Sphere

To find the volume of a sphere having radius ‘r’ we use the formula:

The volume of a sphere = 4/3 πr³

S.I. unit is given cubic meters ( m³)

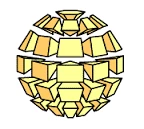
Volume of the sphere  = Summation of the volumes of all pyramids (as shown in figure above)

= 1/3A₁r + 1/3A₂r  + 1/3A₃r+….+1/3A_nr

= 1/3(A₁ + A₂ + A₃ + …..+ A_n) r

=1/3 (Surface area of the sphere) r

=1/3(4πr²)×r  (since Surface area of the sphere = 4 πr²)

Volume of sphere =4/3(πr³)

In the derivation of sphere volume formula, the sphere is divided into pyramids, and the volume of the sphere is equivalent to the volume of all the pyramids. The total volume is determined by the addition of the pyramids volumes.

Properties of Sphere

Sphere has following characteristics are as follows:

• Sphere has no edge or flat faces.


• A sphere is 3-D symmetrical figure.


• All the points that are on the surface of the sphere at an equal distance from the centre of the sphere.


• It has only a curved surface.

How to Find the Volume of a Sphere?

In order to calculate the sphere volume, we have to follow the steps mentioned below:

First: Get the radius of a sphere and multiply it thrice or find the cubic value of it.

Second: Multiply the above value with the product of 4/3 π.

Last: Write the resultant value in the proper cubic units.

Related Videos:

• Volume and surface area of cube


• Volume and surface area of cone


• Volume and Total surface area of cuboid


• Area and Perimeter of rectangle


• Area and Perimeter of square


• Area and Perimeter of triangle


• Area and Perimeter of trapezium

Solved Examples

Example 1: Calculate the volume of a sphere of diameter 18 m. ( pi = 3.14).

Solution:

Given: Diameter of the sphere (d) = 18 m

Let us first find the radius:

Radius of the sphere (r) = d/2 = 18/2 = 9 m

As we know, the volume of the sphere (V) = 4/3 π r³ cubic units.

V = 4/3 x 3.14 x 9³

V = 4/3 x 3.14 x 9 x 9 x 9

V= 4 x 3.13 x 3 x 9 x 9

V = 3052.08

Hence the volume of the sphere is 3052.08 m³.

Example 2: A knife cuts the sphere through the centre of a sphere and forms a circle with a radius of 6 m. Find the surface area of the sphere?

Solution:

Radius of the sphere = 6 m

Surface area of the sphere (V) = 4 π r² sq. units

= 4 x 3.14 x 6 x 6

= 452.16

Hence area of the sphere is 452.16 m².

Example 3: What is the cost required to paint a spherical ball with a radius of 14 cm. If the cost of painting 1 cm²  area is Rs. 3. (pi = 22/7)

Solution: Radius of ball = 14 cm

 The total surface area of a sphere =  4 π r² sq.units

= 4 × (22/7) × 14 × 14

= 4 x 22 x 2 x 14

= 2464 cm²

Thus, total cost of painting the ball = 3 × 2464 = Rs. 7392

Example 5- Find the curved surface area of a sphere having a radius of 2.1 cm. (pi= 22/7)

Solution–

In case of a sphere, we know that

Curved surface area of sphere = Total surface area of sphere (since there are no edges and no flat faces)

Therefore, CSA of sphere = 4 π r² sq. units

= 4 × (22/7) × 2.1 × 2.1

=4 x 22 x 0.3 x 2.1

Therefore, the curved surface area of a sphere= 55.44 cm²

Important Chapters:

• NCERT Solutions for Maths


• NCERT Solution for Class 6 Maths Chapter Mensuration


• NCERT Solution for Class 8 Maths Chapter Mensuration