The taxi fare in a city is as follows: For the first kilometre, the fare is Rs 8 and for the subsequent distance it is Rs 5 per km. Taking the distance covered as x km and total fare as Rs y, write a linear equation for this information, and draw its graph.
To solve the problem, we need to derive a linear equation based on the given taxi fare structure and then graph it.
Step 1: Understand the fare structure
- For the first kilometer, the fare is Rs 8.
- For every subsequent kilometer, the fare is Rs 5 per kilometer.
Step 2: Define the variables
Let:
- x = total distance covered in kilometers
- y = total fare in rupees
Step 3: Formulate the equation
1. If the distance x is 1 km, then the fare y is Rs 8.
2. If the distance x is more than 1 km (let's say x km where x>1), the fare can be calculated as follows:
- The fare for the first kilometer = Rs 8
- The fare for the remaining (x−1) kilometers = Rs 5 per km
Thus, the total fare y can be expressed as:
y=8+5(x−1)
This simplifies to:
y=8+5x−5
y=5x+3
Step 4: Write the linear equation
The linear equation representing the taxi fare based on the distance covered is:
y=5x+3
Step 5: Determine points for graphing
To graph the equation, we can find a few points by substituting values for x:
1. If x=0:
y=5(0)+3=3(Point: (0, 3))
2. If x=1:
y=5(1)+3=8(Point: (1, 8))
3. If x=2:
y=5(2)+3=13(Point: (2, 13))
4. If x=3:
y=5(3)+3=18(Point: (3, 18))
Step 6: Plot the points and draw the graph
- Plot the points (0, 3), (1, 8), (2, 13), and (3, 18) on a graph.
- Draw a straight line through these points to represent the linear equation.
Summary of the solution:
The linear equation for the taxi fare based on the distance covered is:
y=5x+3
- For the first kilometer, the fare is Rs 8.
- For every subsequent kilometer, the fare is Rs 5 per kilometer.
Let:
- x = total distance covered in kilometers
- y = total fare in rupees
1. If the distance x is 1 km, then the fare y is Rs 8.
2. If the distance x is more than 1 km (let's say x km where x>1), the fare can be calculated as follows:
- The fare for the first kilometer = Rs 8
- The fare for the remaining (x−1) kilometers = Rs 5 per km
y=8+5(x−1)
This simplifies to:
y=8+5x−5
y=5x+3
The linear equation representing the taxi fare based on the distance covered is:
y=5x+3
To graph the equation, we can find a few points by substituting values for x:
1. If x=0:
y=5(0)+3=3(Point: (0, 3))
2. If x=1:
y=5(1)+3=8(Point: (1, 8))
3. If x=2:
y=5(2)+3=13(Point: (2, 13))
4. If x=3:
y=5(3)+3=18(Point: (3, 18))
- Plot the points (0, 3), (1, 8), (2, 13), and (3, 18) on a graph.
- Draw a straight line through these points to represent the linear equation.
The linear equation for the taxi fare based on the distance covered is:
y=5x+3
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:LINEAR EQUATIONS IN TWO VARIABLESExercise:EXERCISE 4.2
Explore 4 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:LINEAR EQUATIONS IN TWO VARIABLESExercise:SOLVED EXAMPLES
Explore 9 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBook:NCERTChapter:LINEAR EQUATIONS IN TWO VARIABLESExercise:EXERCISE 4.4
Explore 2 Videos
- INTRODUCTION TO EUCLIDS GEOMETRYBook:NCERTChapter:INTRODUCTION TO EUCLIDS GEOMETRYExercise:Solved Examples
Explore 3 Videos
- LINES AND ANGLESBook:NCERTChapter:LINES AND ANGLESExercise:SOLVED EXAMPLES
Explore 8 Videos
Similar Questions
The taxi fare in a city is as follows: For the first kilometer, the fare is Rs.8 and for the subsequent distance it is Rs 5 per km. Taking the distance covered as x km and total fare as Rs. y, writes a linear equation for this information, and draw its graph.
View SolutionThe taxi fare in a city is as follows: For the first kilometer, the fare is Rs.8 and for the subsequent distance it is Rs 5 per km. Taking the distance covered as x km and total fare as Rs. y, writes a linear equation for this information, and draw its graph.
View SolutionThe taxi fare in a city is charged according to the rates stated as : For the first 3 km distance, the fare is Rs. 10 (even for less than 3 km) and for the subsequent distance is Rs.4 per km. Taking the distance covered as x km and total fare as Rs. y, write a linear equation for the above and draw its graph From the graph, find the distance travelled when the amount paid is Rs. 60.
View SolutionA taxi charges .Rs 20 for the first kilometre and @12 .Rs per km for subsequent distance covered. Taking the total distance covered as x km and total fare .Rs y, write a linear equation depicting the relation between x and y. Draw the graph between x and y.
Form your graph, find the taxi charges for covering
(a) 12 km and (b) 20 km.
View SolutionaA three-wheeler scooter charges 15 for first kilometer and 8 each for every subsequent kilometer. For a distance of x km, an amount of y is paid. Write the equation representing the above information
View SolutionThe parking charges of a car in a private parking is Rs 20 for the first hour and Rs 10 for subsequent hours.Taking total parking charges to be y & total parking time as x hours form a linear equation.Write it in standard form.
View SolutionThe taxi fare after each km when the fare is Rs 15 for the first km and Rs 8 for each additional km
View SolutionA taxi meter shows charges of Rs. 50 for the first two kilometres of journey and Rs. 16 for every subsequent kilometre travelled. Manju pays Rs. 258 as fare to travel fromher house to the railway station. How far is the railway station from her home?
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
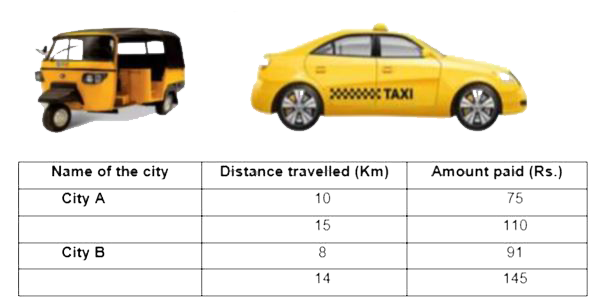
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
If the fixed charges of auto rickshaw be Rs x and the running charges be Rs y km/hr, the pair of linear equations representing the situation is
View SolutionThe taxi charges in a city comprise of a fixed charge together with the charge for the distance covered. For a journey of 10 km the charge paid is Rs 75 and for a journey of 15 km the charge paid is Rs 110. What will a person have to pay for travelling a distance of 25 km?
View Solution