In a triangle OAB, E is the midpoint of OB and D is a point on AB such that AD:DB=2:1. IF OD and AE intersects at P, then ratio of OPPD is equal to
3:2
2:3
3:4
4:3
The correct Answer is:A
To solve the problem, we need to find the ratio of OP to PD in triangle OAB where E is the midpoint of OB and D divides AB in the ratio 2:1.
1. Define the Points Using Vectors:
Let the position vectors of points O, A, and B be represented as →O=→0, →A=→a, and →B=→b.
2. Find the Position Vector of Point E:
Since E is the midpoint of OB:
→E=→O+→B2=→0+→b2=→b2
3. Find the Position Vector of Point D:
Point D divides AB in the ratio 2:1. Using the section formula:
→D=2→B+1→A2+1=2→b+→a3
4. Express the Points P on Lines AE and OD:
Let P divide AE in the ratio λ:1. Then:
→P=λ→E+1→Aλ+1=λ(→b2)+→aλ+1=λ→b2+→aλ+1
5. Express P on Line OD:
Let P divide OD in the ratio γ:1:
→P=γ→D+1→Oγ+1=γ(2→b+→a3)+→0γ+1=2γ→b+γ→a3γ+1
6. Equate the Two Expressions for →P:
Set the two expressions for →P equal to each other:
λ→b2+→aλ+1=2γ→b+γ→a3γ+1
7. Cross Multiply to Eliminate the Denominators:
Cross-multiplying gives:
(λ→b2+→a)(γ+1)=(2γ→b+γ→a)(λ+1)
8. Expand and Rearrange:
Expanding both sides and rearranging will lead to:
λγ→b2+λ→a+γ→b2+→a=2λγ→b+λγ→a+2γ→b+γ→a
9. Solve for λ and γ:
After simplifying, we will find values for λ and γ.
10. Find the Ratio OPPD:
From the expressions for OP and PD:
OP=35ODandPD=25OD
Thus, the ratio:
OPPD=35OD25OD=32
Final Answer:
The ratio OPPD is 32.
Let the position vectors of points O, A, and B be represented as →O=→0, →A=→a, and →B=→b.
Since E is the midpoint of OB:
→E=→O+→B2=→0+→b2=→b2
Point D divides AB in the ratio 2:1. Using the section formula:
→D=2→B+1→A2+1=2→b+→a3
Let P divide AE in the ratio λ:1. Then:
→P=λ→E+1→Aλ+1=λ(→b2)+→aλ+1=λ→b2+→aλ+1
Let P divide OD in the ratio γ:1:
→P=γ→D+1→Oγ+1=γ(2→b+→a3)+→0γ+1=2γ→b+γ→a3γ+1
Set the two expressions for →P equal to each other:
λ→b2+→aλ+1=2γ→b+γ→a3γ+1
Cross-multiplying gives:
(λ→b2+→a)(γ+1)=(2γ→b+γ→a)(λ+1)
Expanding both sides and rearranging will lead to:
λγ→b2+λ→a+γ→b2+→a=2λγ→b+λγ→a+2γ→b+γ→a
After simplifying, we will find values for λ and γ.
From the expressions for OP and PD:
OP=35ODandPD=25OD
Thus, the ratio:
OPPD=35OD25OD=32
The ratio OPPD is 32.
Topper's Solved these Questions
- VECTORBook:FIITJEEChapter:VECTORExercise:ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-II
Explore 18 Videos
- VECTORBook:FIITJEEChapter:VECTORExercise:COMPREHENSIONS
Explore 3 Videos
- VECTORBook:FIITJEEChapter:VECTORExercise:ASSIGNMENT PROBLEMS (SUBJECTIVE) LEVEL-II
Explore 6 Videos
- TRIGONOMETIC EQUATIONS Book:FIITJEEChapter:TRIGONOMETIC EQUATIONS Exercise:NUMERICAL BASED
Explore 3 Videos
Similar Questions
In a △OAB,E is the mid point of OB and D is the point on AB such that AD:DB=2:1 If OD and AE intersect at P then determine the ratio of OP:PD using vector methods
View SolutionIn a △OAB, E is the mid-point of BO and D is a point on AB such that AD : DB = 2:1. If OD and AE intersect at P, determine the ratio OP: PD using vector method.
View Solution
Knowledge Check
In triangle ABC,D is the midpoint of AB, E is the midpoint of DB and F is the midpoint of BC. If the area of ΔABC is 96, the area of ΔAEF is
A16B24C32D36D,E,F are the mid points of BC,CAandAB of ΔABC. If ADandBE intersect in G, then AG+BG+CG is equal to
AAD=BE=CFB23(AD+BE+CF)C32(AD+BE+CF)D13(AD+BE+CF)
In ΔABC,D is point on side AB and E is a point on side AC such that ∠ADE=∠ABC,AD=2,BD=3 and AE=3, then what is the value of CE ?
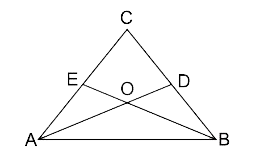
View SolutionIn the given figure ∠ABC=∠BAC , D and E are pointts on BC and AC respectively such that DB = AE . If AD and BE intersect at O then prove that OA = OB
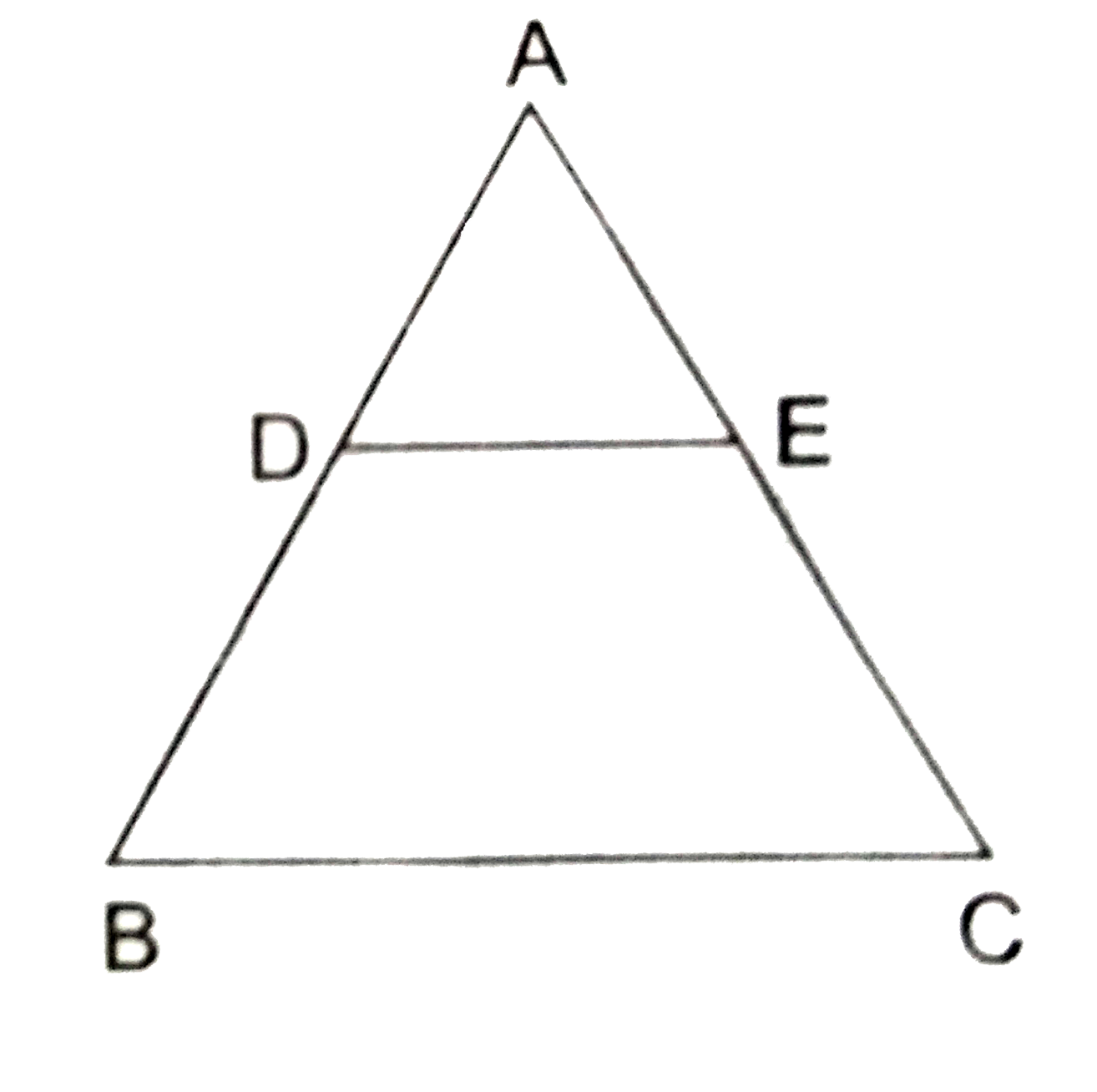
View SolutionIn an equilateral ΔABC,D is the midpoint of AB and E is the midpoint of AC. Then, ar(ΔABC):ar(ΔADE)=?
View SolutionIn the given figure, in △ABC, D is the mid-point cf AB and P is any point on BC. If CQ∣∣PD meets AB in Q and area of △ABC=30cm2, then, area of △BPQ is
View SolutionGiven that AD is the median on BC of ΔABC,point on AD such that AE=13AD. The line CE intersects AB at F. If AF=1.2cm, find the length of AB.
View SolutionIn △ABC, D and E are the points on sides AB and BC respectively such that DE || AC. If AD : DB = 5 : 3, then what is the ratio of the area of △BDE to that of the trapezium ACED?
View Solution