Question

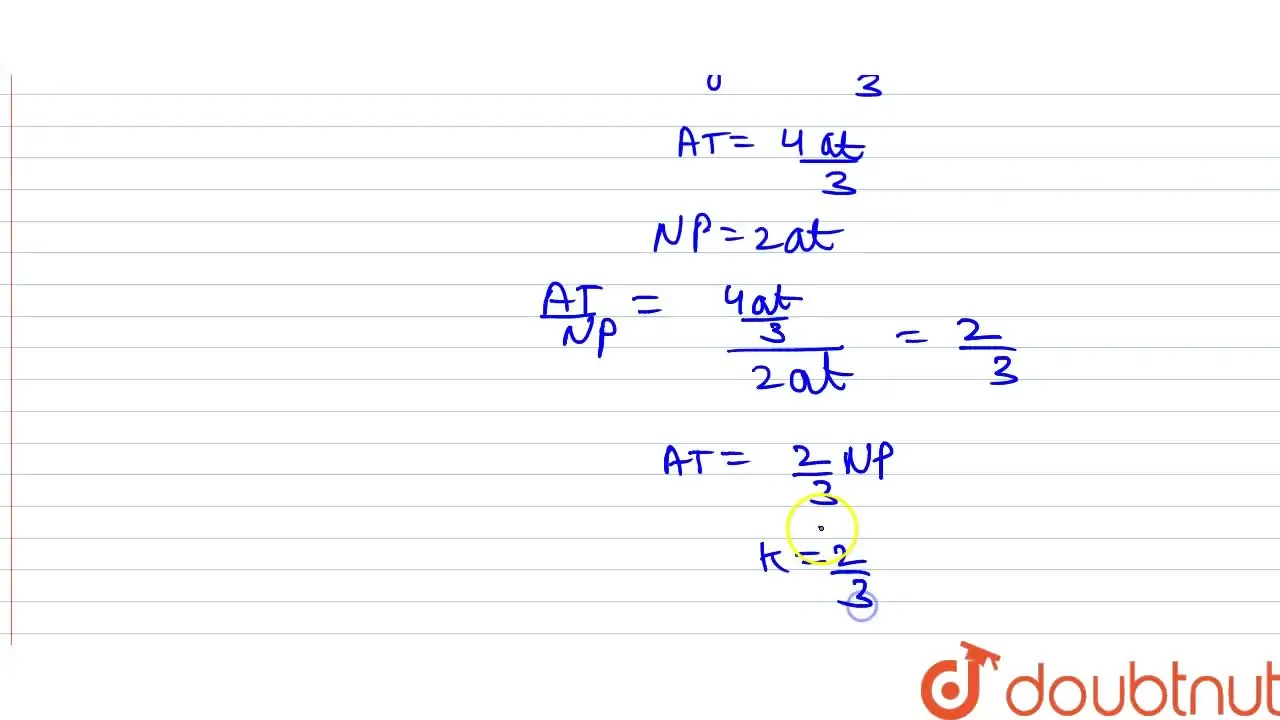
A straight line is drawn parallel to the x-axis to bisect an ordinate PN of the parabola $y^2=4ax$ and to meet the curve at Q. Moreever , NQ meets the tangent at the vertex A of the parabola at T. $AT=Knp$ , then k is equal to

Options

A. `1/3`
B. `2/3`
C. `3/2`
D. 1

Answer

B

Explanation

To solve the problem step by step, we will follow the reasoning given in the video transcript and derive the necessary equations and values.

Step 1: Understand the Parabola and Points
The given parabola is \( y^2 = 4ax \). For simplicity, we can assume \( a = 1 \), so the equation becomes \( y^2 = 4x \).

Step 2: Identify Points P and N
Let the point \( P \) be \( (at^2, 2at) \) which can be simplified to \( (t^2, 2t) \) since we assumed \( a = 1 \). The ordinate \( PN \) is a vertical line at \( x = t^2 \). The point \( N \) on the x-axis corresponding to \( P \) is \( (t^2, 0) \).

Step 3: Find the Midpoint of PN
The midpoint of the line segment \( PN \) is given by:
\( \text{Midpoint} = \left(t^2, \frac{2t + 0}{2}\right) = \left(t^2, t\right) \)
This midpoint lies on the line parallel to the x-axis, which is \( y = t \).

Step 4: Find Intersection Point Q
To find the intersection point \( Q \) of the line \( y = t \) with the parabola \( y^2 = 4x \), substitute \( y = t \) into the parabola's equation:
\( t^2 = 4x \implies x = \frac{t^2}{4} \)
Thus, the coordinates of point \( Q \) are \( \left(\frac{t^2}{4}, t\right) \).

Step 5: Find the Tangent at Vertex A
The vertex \( A \) of the parabola \( y^2 = 4x \) is at the origin \( (0, 0) \). The equation of the tangent to the parabola at the vertex is:
\( y = 0 \)

Step 6: Find the Equation of Line NQ
The slope of line \( NQ \) can be calculated as follows:
\( \text{slope} = \frac{y_2 - y_1}{x_2 - x_1} = \frac{t - 0}{\frac{t^2}{4} - t^2} = \frac{t}{\frac{t^2}{4} - \frac{4t^2}{4}} = \frac{t}{-\frac{3t^2}{4}} = -\frac{4}{3t} \)
Using point-slope form, the equation of line \( NQ \) is:
\( y - 0 = -\frac{4}{3t}(x - t^2) \implies y = -\frac{4}{3t}x + \frac{4}{3} \)

Step 7: Find Intersection Point T
To find point \( T \), set \( y = 0 \) in the equation of line \( NQ \):
\( 0 = -\frac{4}{3t}x + \frac{4}{3} \implies \frac{4}{3t}x = \frac{4}{3} \implies x = t \)
Thus, the coordinates of point \( T \) are \( (t, 0) \).

Step 8: Calculate Lengths AT and NP
- The length \( AT \) is the distance from \( A(0, 0) \) to \( T(t, 0) \):
\( AT = t \)
- The length \( NP \) is the distance from \( N(t^2, 0) \) to \( P(t^2, 2t) \):
\( NP = 2t \)

Step 9: Establish the Relationship
According to the problem, we have:
\( AT = k \cdot NP \implies t = k \cdot 2t \)
Dividing both sides by \( t \) (assuming \( t \neq 0 \)):
\( 1 = 2k \implies k = \frac{1}{2} \)

Final Answer
Thus, the value of \( k \) is:
\( \boxed{\frac{1}{2}} \)