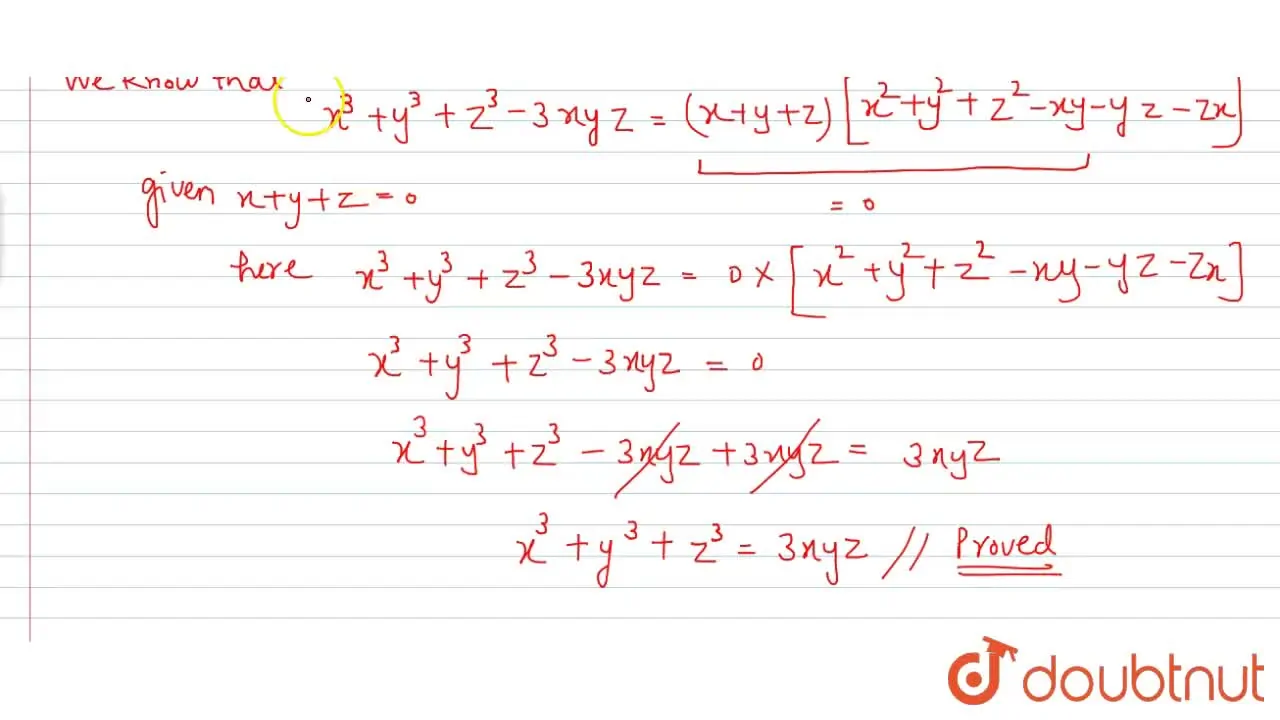
If x+y+z=0 show that x3+y3+z3=3xyz.
Video Solution
Text Solution
We have this formula,
x3+y3+z3−3xyz=(x+y+z)(x2+y2+z2−xy−yz−zx)
Here, we are given, x+y+z=0,
So,our formula becomes
x3+y3+z3−3xyz=0∗(x2+y2+z2−xy−yz−zx)
x3+y3+z3−3xyz=0
x3+y3+z3=3xyz
|
Updated on:21/07/2023
Similar Questions
If x+y+z=0 show that x3+y3+z3=3xyz.
View SolutionIf x+y+z=0 then x3+y3+z3+3xyz equal to
View SolutionIf x=y+zthenx3−y3−z3 is
View SolutionIf x+y+z=xyz, show that : 3x−x31−3x2+3y−y31−3y2+3z−z31−3z2=3x−x31−3x2.3y−y31−3y2.3z−z31−3z2
View SolutionShow that : ∣∣xyzx2y2z2x3y3z3∣∣=xyz(x−y)(y−z)(z−x).
View Solution(i) Factorize the experssions 8a6+5a3+1
(ii) show that (x−y)3+(z−x)3+(z−x)3=3(x−y)(y−z)(z−x)View SolutionIf x + y + z = 0 , then (x+y−z)3+(y+z−x)3+(z+x−y)3=k(xyz) , where k is equal to :
View SolutionSolve the given equations:
x+y+z=0
x3+y3+z3=18
x7+y7+z7=2058 where x,y,z∈RView SolutionIf x,y,z gt0,then(x^3+y^3+z^3)/(x^3y^3z^3)
View SolutionIf x=y+zthenx3−y3−z3 is
View Solution