Suppose ABCDEF is a hexagon such that AB=BC=CD=1 and DE=EF=FA=2. If the vertices A,B,C,D,E,F, are concylic, the radius of the circle passing throught them is-
√52
√73
√115
√2
The correct Answer is:B
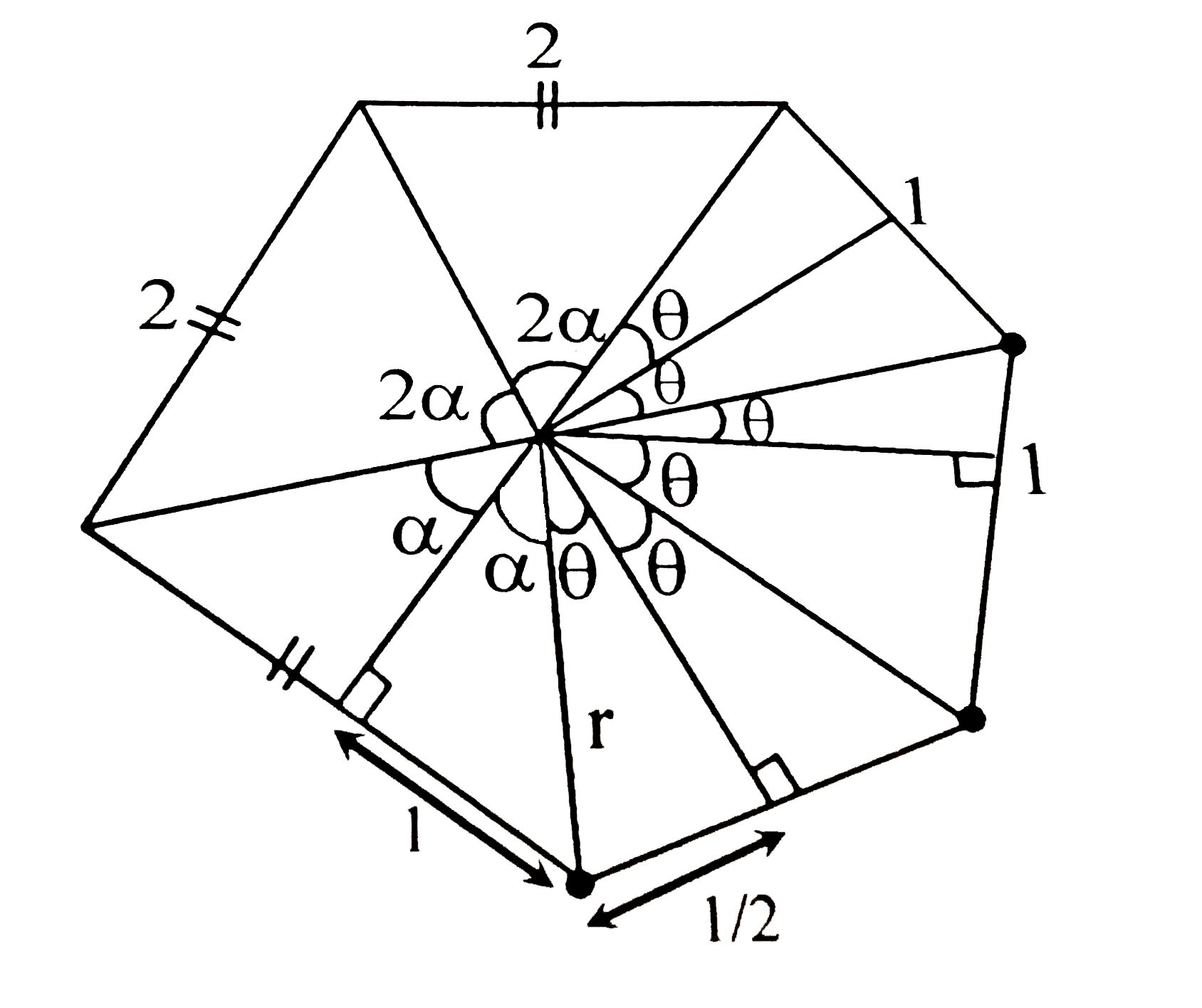
From the figure :
sinθ=12r&sinα=1r
3×(2θ)+(2α)×3=360∘
θ+α=60∘
Now, cos(θ+α)=12
⇒cosθ.cosα−sinθ.sinα=12
⇒√1−14r2√1−1r2−12r⋅1r=12
⇒√4r2−1√r2−1−1=r2
⇒(4r2−)(r2−1)=(r2+1)2
⇒4r4−5r2+1=r4/+2r2+1
⇒3r4=7r2
⇒3r4=7r2
⇒r2=73
⇒r=√73
Similar Questions
Knowledge Check
If AB =112cm, BC=16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is
A90∘B120∘C60∘D150∘If ABCDEF is a regular hexagon , then A→D+E→B+F→C equals
A2A→BB→0C3A→BD4A→BLet A, B, C, D be points on a vertical line such that AB = BC = CD. If a body is released from position A, the times of descent through AB, BC and CD are in the ratio.
A1:(√3−√2):(√3+√2)B1:(√2−1):(√3−√2)C1:(√2−1):√3D1:√2:(√3−1)
ABCDEF is a regular hexagon. With vertices A,B,c,D,E and F as the centres, circles same radius r are drawn. Find the area of the shaded portion shown in the given figure.
View SolutionLet ABCDEF be a regular hexagon and −−→AB=→a,−−→BC=→b,−−→CD=→c then −−→AE is
View SolutionLet ABCDEF be a regular hexagon whose centre is at the origin. (A,B,C,D,EandF are in anticlockwise direction). If the coordinates of A be 1+i then C lies in
View SolutionThe equiangular convex hexagon ABCDEF has AB=1,BC=4,CD=2,andDE=4. The area of the hexagon is
View SolutionAB and CD are two parallel chords of a circle such that AB = 6 cm and CD = 2AB. Both chords are on the same side of the centre of the circle. If the distance between is equal to one-fourth of the length of CD, then the radius of the circle is-
AB और CD किसी वृत्त की दो ऐसी समानांतर जीवाएं है कि AB = 6 सेमी है और CD = 2AB है | दोनों जीवाएं वृत्त के केंद्र के एक ही तरफ हैं | यदि बीच की दूरी CD की लंबाई के एक चौथाई के बराबर है, तो वृत्त की त्रिज्या ज्ञात करें |
View Solution